Two identical metal plates are positively charged to Q1 and Q2(Q2<Q1). If they are brough near each other to form a parallel plate capacitor of capacitance C, then what will be the potential differences between the plates?
Let the area of the metal plates be A and intensity of the electric fields at any point between the plates due to the first and second metal plates be E1 and E2 respectively.
Electric field at any between the plates due to first plate,
E1=Q12A∈0
Electric field at any point between the plates due to second plate,
E2=Q22A∈0
So net electric field,
E=E1−E2=(Q1−Q22A∈0) [ where ∈0= permittivity of free space ]
Again capacitance of parallel plate capacitor,
C=∈0Ad[d= distance between the two plates ]
We know, potential difference = net electric field × distance
∴V=(E1−E2)d=(Q1−Q22A∈0)d=(Q1−Q22)dA∈0
Hence potential difference,
V=Q1−Q22C[∴C=∈0Ad].
Topper's Solved these Questions
- BOOK - CHHAYA PUBLICATIONCHAPTER - CAPACITANCE AND CAPACITOREXERCISE - NCERT TEXTBOOK QUESTIONS12videos
CAPACITANCE AND CAPACITOR
- BOOK - CHHAYA PUBLICATIONCHAPTER - CAPACITANCE AND CAPACITOREXERCISE - MULTIPLE CHOICE QUESTIONS29videos
CAPACITANCE AND CAPACITOR
- BOOK - CHHAYA PUBLICATIONCHAPTER - CAPACITANCE AND CAPACITOREXERCISE - SECTION RELATED QUESTIONS30videos
CAPACITANCE AND CAPACITOR
- BOOK - CHHAYA PUBLICATIONCHAPTER - ATOMIC NUCLEUSEXERCISE - CBSE SCANNER14videos
ATOMIC NUCLEUS
- BOOK - CHHAYA PUBLICATIONCHAPTER - COMMUNICATION STSTEMEXERCISE - CBSE SCANNER28videos
COMMUNICATION STSTEM
Similar Questions
Two identical metal plates are given poistive charges Q1 and Q2 (<Q1) respectively. If they are now brought close together to form a parallel plate capacitor with capacitance C, the potencial difference between them is
View SolutionImagine a light planet revolving around a very massive star in a circular orbit of radius r with direction T. On what power of r, will the square of time period depends if the gravitational force of attraction between the planet and the star is proportional to r−5/2 .
View Solution
Knowledge Check
- Question 1 - Select One
Two identical metal plates are given positive charges Q1 and Q2 (<Q1) respectively. If they are now brought close together to form a parallel plate capacitor with capacitance C, the potential difference between them is :
AQ1+Q22CBQ1+Q2CCQ1−Q2CDQ1−Q22C - Question 2 - Select One
Two identical metal plates are given positive charges Q1 and Q2 (<Q1) respectively. If they are now brought close together to form a parallel plate capacitor with capacitance C, the potential difference between them is :
AQ1+Q22CBQ1+Q2CCQ1−Q2CDQ1−Q22C - Question 3 - Select One
Two identical metal plates are given poistive charges Q1 and Q2 (<Q1) respectively. If they are now brought close together to form a parallel plate capacitor with capacitance C, the potencial difference between them is
A(a) (Q1+Q2)/(2C)B(b) (Q1+Q2)/CC(c) (Q1−Q2)/CD(d) (Q1−Q2)/(2C)
In an isolated parallel plate capacitor of capacitance C, the plates have charge Q1 and Q2. The potential difference between the plates is
View SolutionTwo plates ( area =s ) charged to +q1 and +q2(q2<q1) are brought closer to form a capacitor of capacitance C. The potential difference across the plates is
View SolutionAn isolated parallel-plate capacitor of capacitor C has paltes X and Y. If plate X is given charge Q, the potential difference between X and Y Is
View SolutionIn an isolated parallel plate capacitor of capacitancee C, the four surface have chrges Q1,Q2,Q−(3) and Q4 as shown. The potential difference between the plates is
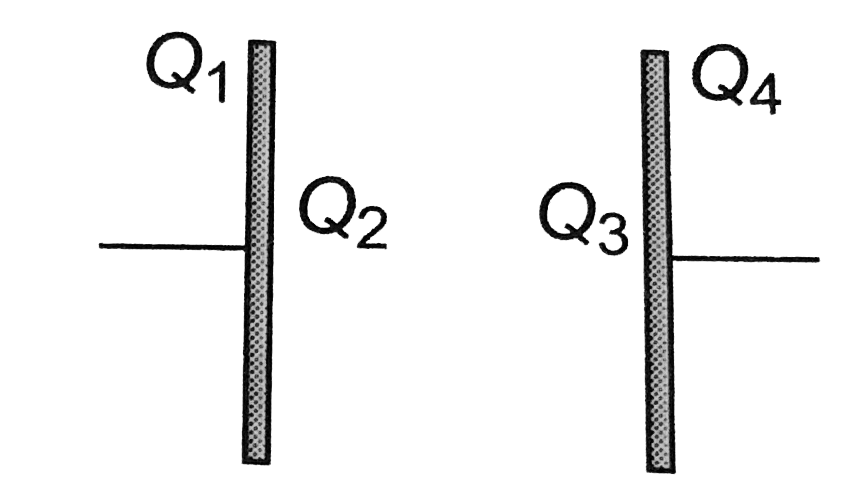 View Solution
View SolutionIn a parallel-plate capacitor of plate area A, plate separation d and charge Q the force of attraction between the plates is F.
View Solution

