Two identical parallel plate capacitors are connected in series to a battery of 100V. A dielectric slab of dielectric constant 4.0 is inserted between the plates of second capacitor. The potential difference across the capacitors will now be respectively
50V,50V
80V,20V
20V,80V
75V,25V
The correct Answer is:B
To solve the problem, we need to determine the potential difference across two identical parallel plate capacitors connected in series, where the second capacitor has a dielectric slab inserted. Here’s the step-by-step solution:
Step 1: Understand the Initial Setup
We have two identical capacitors, both with capacitance C, connected in series to a 100V battery.
Step 2: Calculate Initial Voltage Distribution
In a series connection, the total voltage is divided across the capacitors. Since both capacitors are identical, the voltage across each capacitor is the same. Therefore, the potential difference across each capacitor before inserting the dielectric is:
V1=V2=100V2=50V
Step 3: Insert the Dielectric
Now, we insert a dielectric slab with a dielectric constant K=4.0 into the second capacitor. The capacitance of the second capacitor becomes:
C′2=K⋅C=4C
Step 4: Set Up the Voltage Equation
In a series circuit, the charge Q on both capacitors is the same. The relationship between charge, capacitance, and voltage is given by:
Q=C1V1=C′2V2
Where:
- C1=C (first capacitor)
- C′2=4C (second capacitor with dielectric)
Step 5: Relate the Voltages
From the charge equations, we can express V1 and V2:
V1=QCandV2=Q4C
From these, we can relate V1 and V2:
V1=4V2
Step 6: Write the Total Voltage Equation
The total voltage across both capacitors is still 100V:
V1+V2=100V
Step 7: Substitute and Solve
Substituting V1=4V2 into the total voltage equation:
4V2+V2=100V
5V2=100V
V2=100V5=20V
Step 8: Calculate V1
Now, substituting V2 back to find V1:
V1=4V2=4×20V=80V
Final Result
Thus, the potential differences across the capacitors are:
- V1=80V (across the first capacitor)
- V2=20V (across the second capacitor)
Conclusion
The potential difference across the capacitors will now be respectively 80V and 20V.
---
We have two identical capacitors, both with capacitance C, connected in series to a 100V battery.
In a series connection, the total voltage is divided across the capacitors. Since both capacitors are identical, the voltage across each capacitor is the same. Therefore, the potential difference across each capacitor before inserting the dielectric is:
V1=V2=100V2=50V
Now, we insert a dielectric slab with a dielectric constant K=4.0 into the second capacitor. The capacitance of the second capacitor becomes:
C′2=K⋅C=4C
In a series circuit, the charge Q on both capacitors is the same. The relationship between charge, capacitance, and voltage is given by:
Q=C1V1=C′2V2
Where:
- C1=C (first capacitor)
- C′2=4C (second capacitor with dielectric)
From the charge equations, we can express V1 and V2:
V1=QCandV2=Q4C
From these, we can relate V1 and V2:
V1=4V2
The total voltage across both capacitors is still 100V:
V1+V2=100V
Substituting V1=4V2 into the total voltage equation:
4V2+V2=100V
5V2=100V
V2=100V5=20V
Now, substituting V2 back to find V1:
V1=4V2=4×20V=80V
Thus, the potential differences across the capacitors are:
- V1=80V (across the first capacitor)
- V2=20V (across the second capacitor)
The potential difference across the capacitors will now be respectively 80V and 20V.
Topper's Solved these Questions
- ELECTRIC POTENTIAL & CAPACITANCEBOOK - A2ZCHAPTER - ELECTRIC POTENTIAL & CAPACITANCEEXERCISE - Assertion Reason
8 Videos
- ELECTRIC CHARGE, FIELD & FLUXBOOK - A2ZCHAPTER - ELECTRIC CHARGE, FIELD & FLUXEXERCISE - Section D - Chapter End Test
29 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - A2ZCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section D - Chapter End Test
30 Videos
Similar Questions
A capacitor and a bulb are connected in series with a source of alternating emf. If a dielectric slab is inserted between the plates of the capacitor, then
View SolutionTwo idential parallel plate capacitors are connected in sereis to a cell of 120V. A dielectric slab (K=3) in placed in one of the capacitor. The p.d., across the capacitors will now be
View Solution
Knowledge Check
Two identical parallel plate capacitors are connected in series and then joined in series will a batter of 100V. A slab of dielectric constant K=3 is inserted between the plates of the first capacitor. Then, the potential difference across the capacitor will be, respectively.
A25V,75VB75V,25VC20V,80VD50V,50VA parallel plate capacitor is connected across a battery. Now, keeping the battery connected, a dielectric slab is inserted between the plates. In the process,
Ano work doneBwork is done by the battery and the stored energy increases.Cwork is done by the external agent, and the stored energy decteases. ltDwork is done by the battery as well as the external agent, but the stored energy done not charge.Two identical parallel plate air capacitors are connected in series to a battery of emf V. If one of the capacitor is completely filled with dielectric material of constant K, then potential difference of the other capacitor will become
AKV(K+1)BKVK+1CK−1KVDVK(K−1)
A parallel plate capacitor of capacitance 90 pF is connected to a battery of emf 20 V. If a dielectric material of dielectric constant K=53 is inserted between the plates, the magnitude of the induced charge will be :
View SolutionTwo identical capacitor C1 and C2 are connected in series with a battery. They are fully charged. Now a dielectric slab is inserted between the plates of C2. The potential across C1 will :
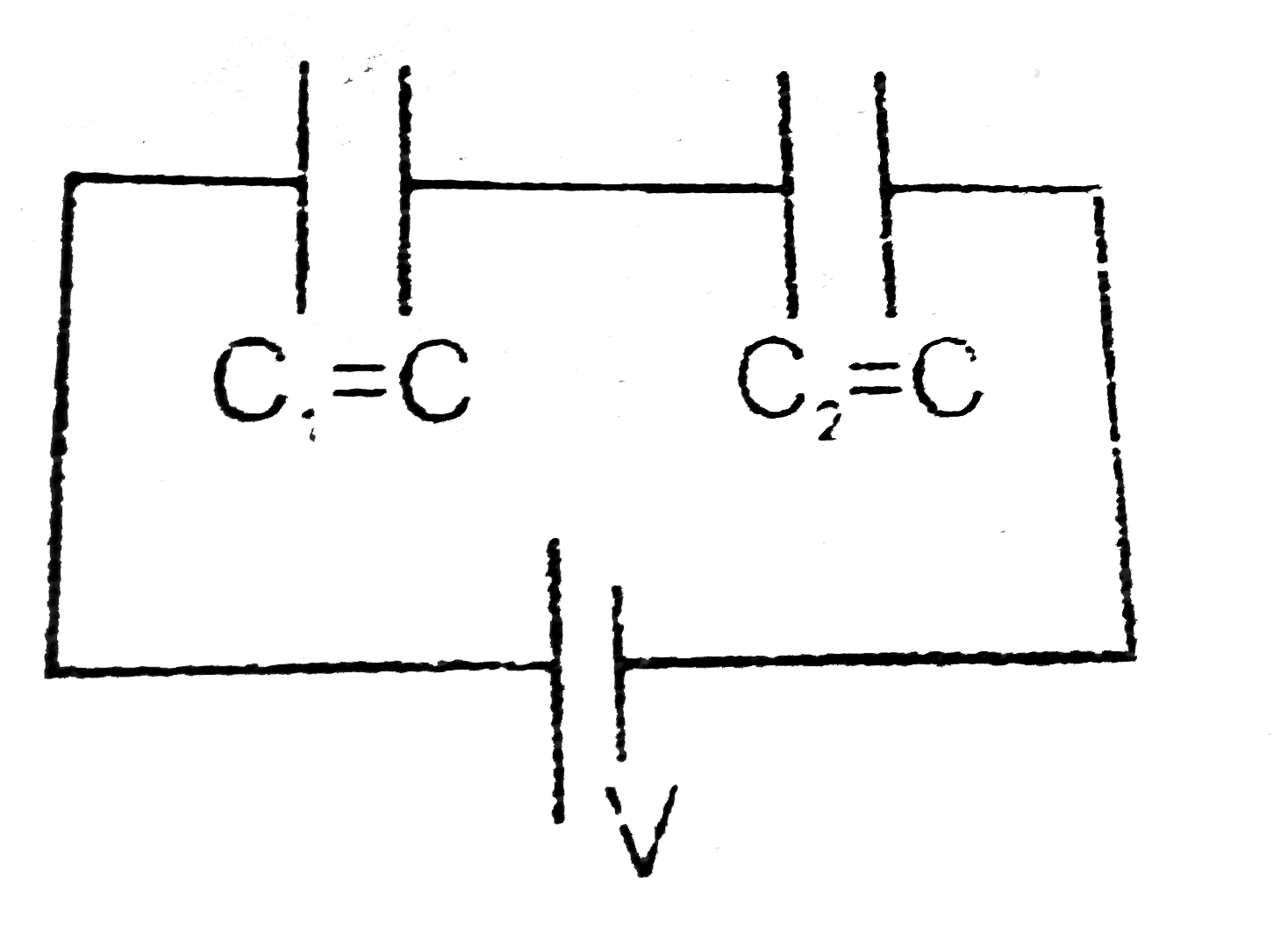
View SolutionA parallel plate capacitor carries a harge Q. If a dielectric slab with dielectric constant K=2 is dipped between the plates, then
View SolutionA parallel capacitor of capacitance C is charged and disconnected from the battery. The energy stored in it is E. If a dielectric slab of dielectric constant 6 is inserted between the plates of the capacitor then energy and capacitance will become.
View SolutionTwo identical capacitors A and B shown in the given circuit are joined in series with a battery. If a dielectric slab of dielectric constant K is slipped between the plates of capacitor B and battery remains connected, then the energy of capacitor A will-
View Solution
