Two masses 2m and m are attached to the ends of the card which passes over a pulley of mass m and radius R. Calculate the tension in the wire on both sides of the pulley. Assume no slipping.
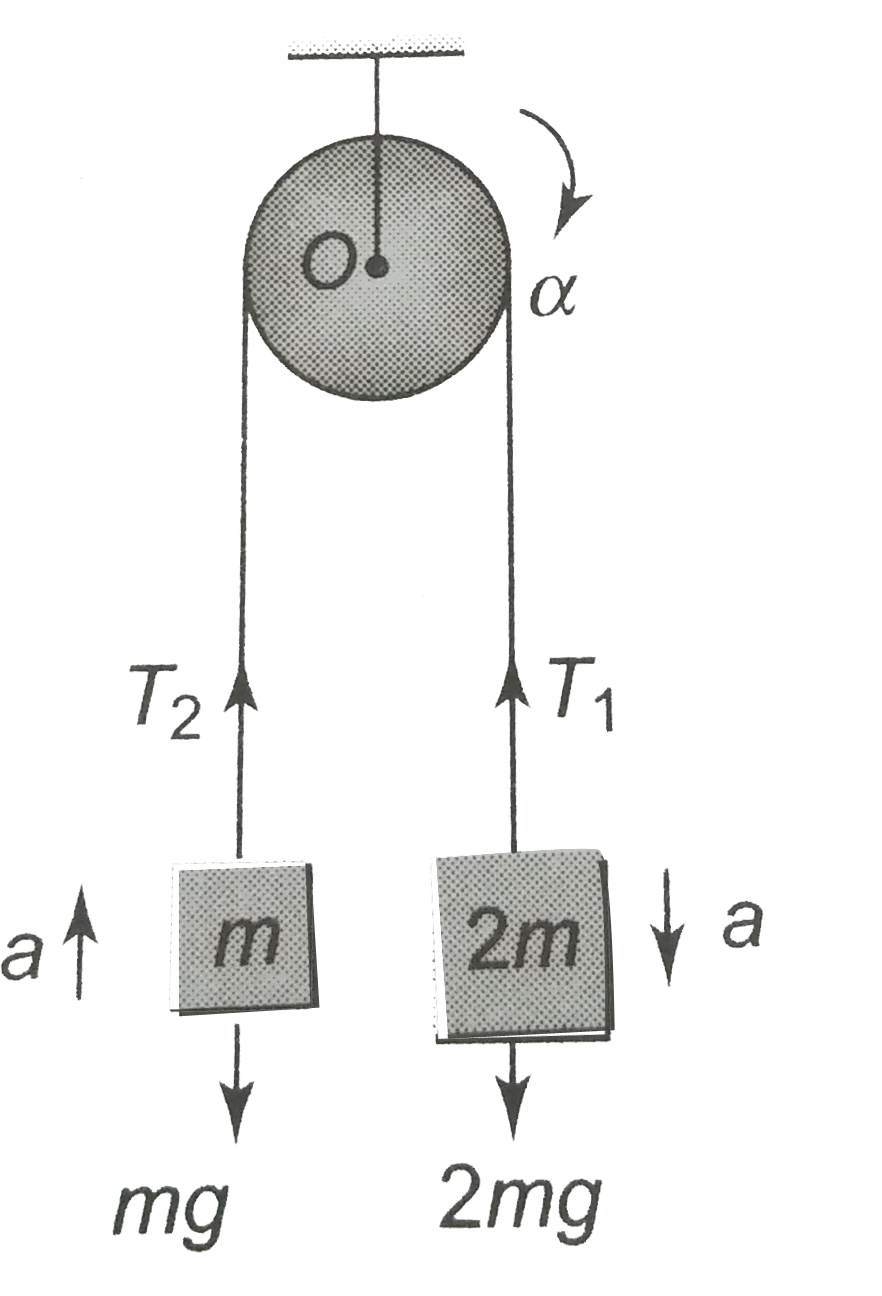
2mg−T1=2ma (i)
T2−mg=ma (ii)
pulley:
τ0=(T1−T2)R=Iα (iii)
I=12mR2 (iv)
a=Rα (v)
From (iii),(iv) and (v),
(T1−T2)R=12mR2(aR)
T1−T2=12ma (vi)
Adding (i). (ii) and (vi), we get
mg=72ma⇒a=2g7
2mg−T1=2m×2g7=4mg7⇒T1=10mg7
T2=m(g+a)=m(g+2g7)=9mg7
Alternatively: considering (blocks + pulley) as system and taking torque about O
τ0=2mgR−mgR=mgR
I0=2mR2+mR2+12mR2=72mR2
α=τ0I0=mgR72mR2=2g7R
a=Rα=2g7

Topper's Solved these Questions
Similar Questions
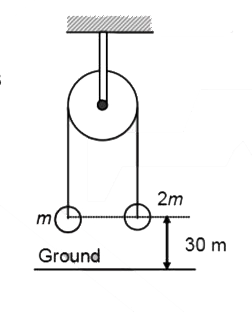
Two masses m and 2m are connected by a massless string, which passes over a pulley as shown in figure. The masses are held initially with equal lengths of the strings on either side of the pulley. Find the velocity of masses at the instant the lighter mass moves up a distance of 15m. ( g=10m/s2 )
 View Solution
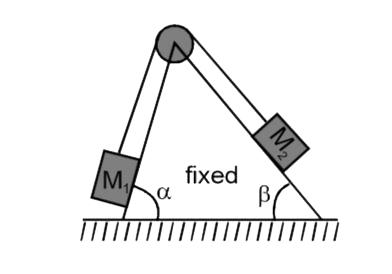
View SolutionTwo masses M1 and M2 are attached to the ends of a light string which passes over a massless pulley attached to the top of a double inclined smooth plane of angles of inclination α and β.The tension in the string is :
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Two masses 2 kg and 3 kg are attached to the end of the string passed over a pulley fixed at the top. The tension and acceleration are
A7g8,g8B21g8,g8C21g8,g5D12g5,g5 - Question 2 - Select One
A block of mass m is attached at the end of an inextensible string which is wound over a rough pulley of mass M and radius R. Assume the string does not slide over the pulley. Find the acceleration of the block when released.
Amg2m+MB2mgm+MCmgm+MD2mg2m+M - Question 3 - Select One
Two bodies of masses 4kg and 6kg are attached to the ends of a string which passes over a pulley, the 4kg mass is attached to the table top by another string.The tension in this string T1 is equal to
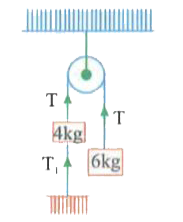 A10NB10.6NC25ND20N
A10NB10.6NC25ND20N
Two blocks of masses 2m and m are connected by a light string going over a pulley of mass 2m and radius R as shown in the figure. The system is released from rest. Find the angular momentum of system when the mass 2m has descended through a height h. Assume no slipping.
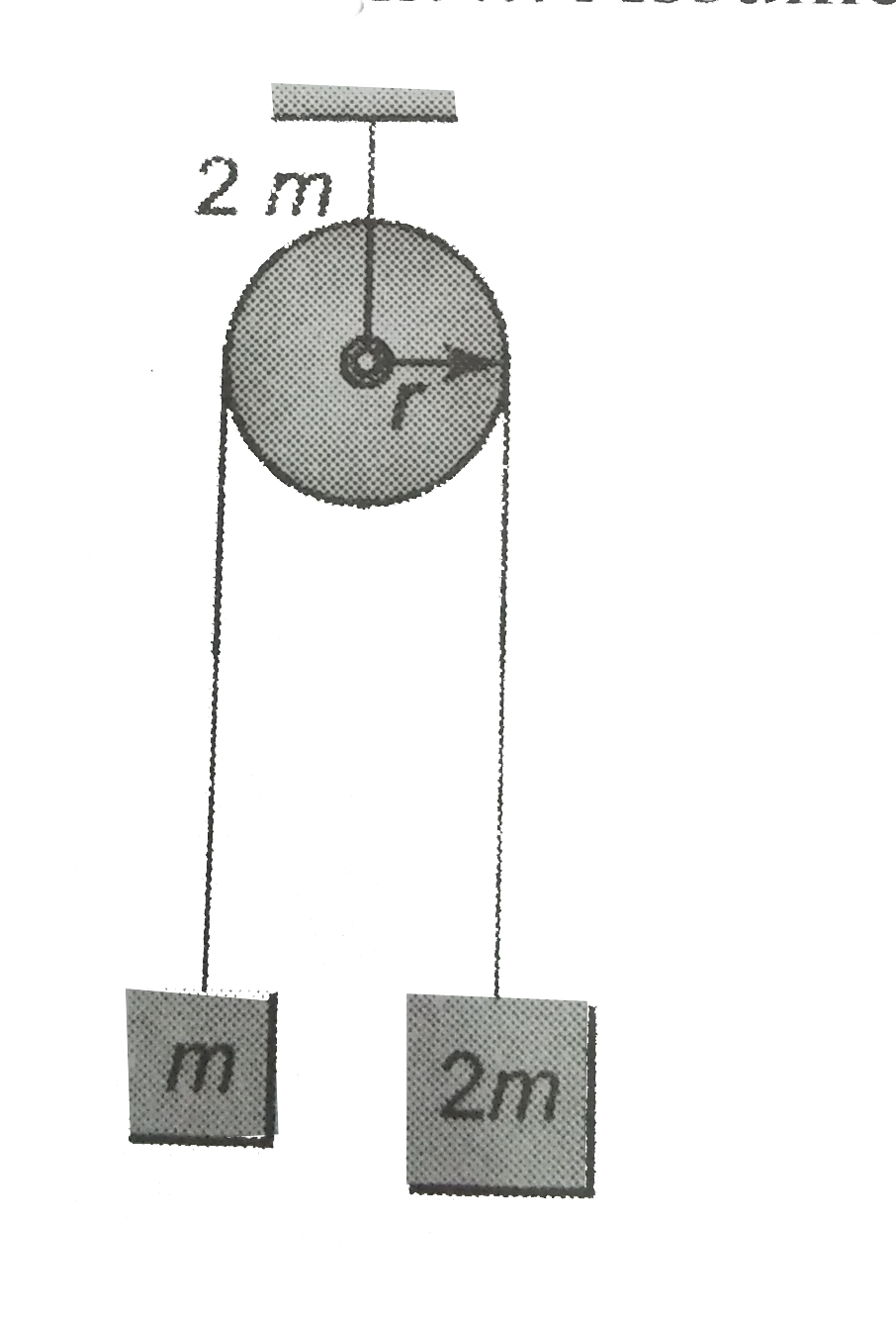 View Solution
View SolutionTwo masses M1 and M2 are attached to the ends of a string which passes over a pulley attached to the top of an inclined plane. The angle of inclination of the plane is 30∘ and M1=10kg,M2=5kg. What is the acceleration of mass M2 ?
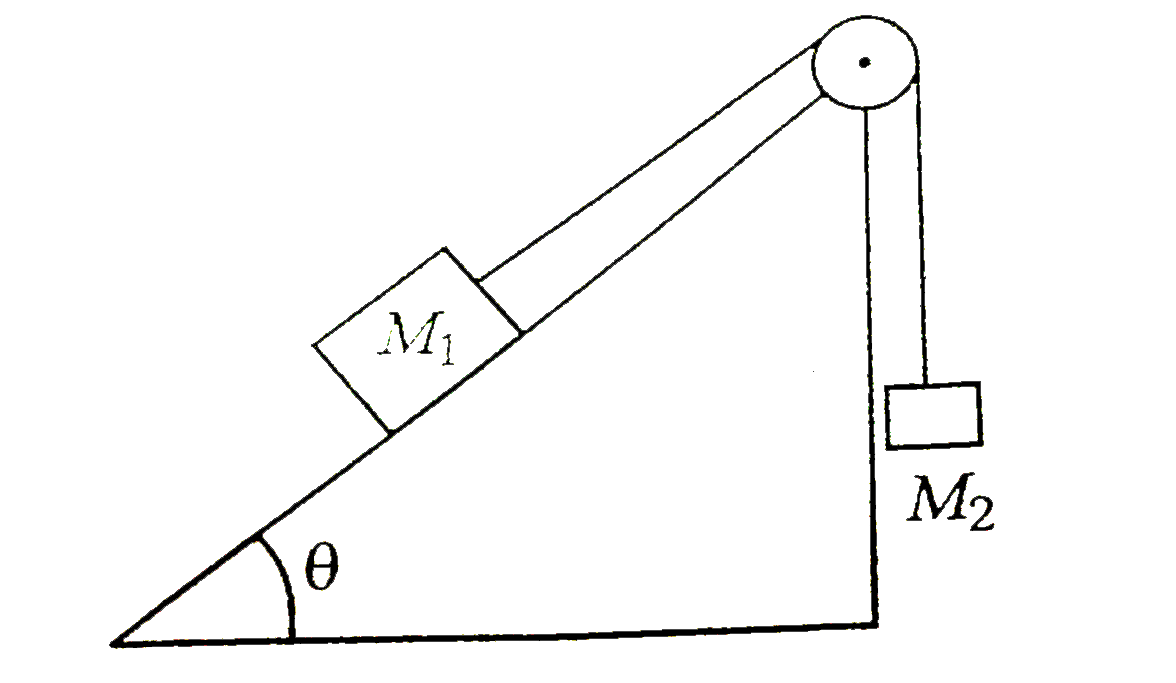 View Solution
View SolutionTwo masses M1andM2 are attached to the ends of string which passes over the pulley attached to the top of a double inclined plane. The angles of inclination of the inclined planes are αandβ. Take g=10ms−2. If M1=M2 and α=β, what is the acceleration of the system?
View SolutionTwo bodies of masses m and M are attached to the two ends of a light string passing over a fixed ideal pulley (M>>m). When the bodies are in motion, the tension in the string is approximately
View SolutionTwo blocks of masses m1=4kg and m2=2kg are connected to the ends of a string which passes over a massless, frictionless pulley. The total downwards thrust on the pulley is nearly
 View Solution
View Solution

