Two masses of 500kg each are placed at two points A and B, distance 5m apart. There is a point P such that AP=3m and BP=4m. Find (i) the magnitude of gravitational intensity at P and
(ii) gravitational potential at P.
Given G=6.67×10−11Nm2kg−2.
Refer to Fig.
52=32+42 or AB2=(AP)2+(BP)2
So ∠APB=90∘
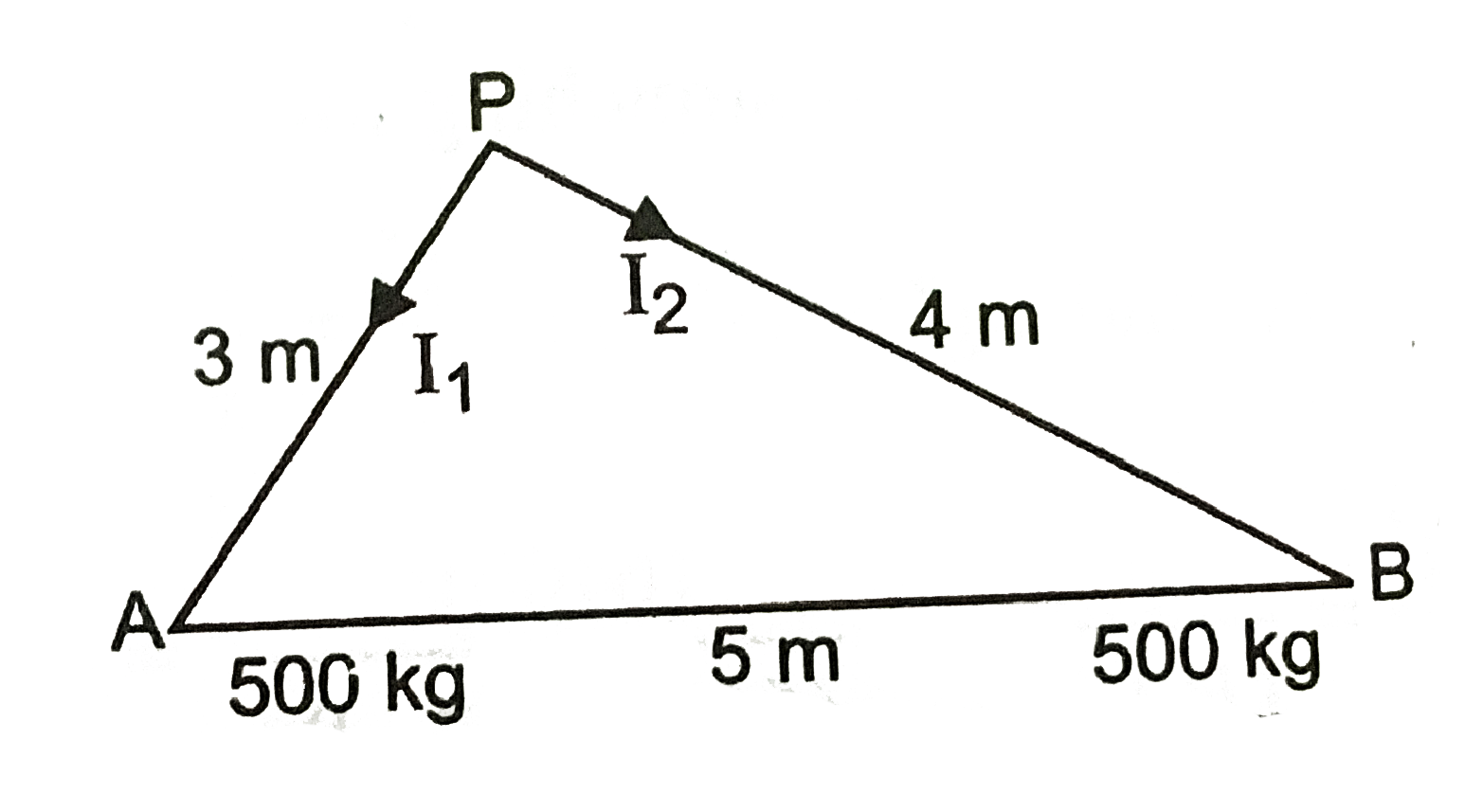
(i) Gravitational intensity at P due to mass at A is
I1=G×500(3)2=5009GalongPA
Gravitational intensity at P due to mass at B is
I2=G×500(4)2=50016GalongPB
As ∠APB=90∘, so the resultant gravitational intensity (in magnitude) at P is
I=√I21+I22=√(5009G)2+(500G16)2
=−6.67×10−11×500×712
=−1.945×10−8Jkg−1
Topper's Solved these Questions
- GRAVIATIONBOOK - PRADEEPCHAPTER - GRAVIATIONEXERCISE - PROBLEMS FOR PRACTIVE B
1 Videos
- GRAVIATIONBOOK - PRADEEPCHAPTER - GRAVIATIONEXERCISE - PROBLEMS FOR PRACTIVE C
1 Videos
- GRAVIATIONBOOK - PRADEEPCHAPTER - GRAVIATIONEXERCISE - PROBLEMS FOR PRACTIVE A
1 Videos
- BEHAVIOUR OF PERFECT GAS & KINETIC THEORYBOOK - PRADEEPCHAPTER - BEHAVIOUR OF PERFECT GAS & KINETIC THEORYEXERCISE - Assertion - Reason Type questions
14 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 NCERT Comprehension
4 Videos
Similar Questions
Two bodies of masses 100kg and 10,000kg are at a distance 1m apart. At which point on the line joining them will the resultant gravitational field intensity is zero? What is the gravitational potential at that point ?
G=6.67×10−11Nm2kg−2.
View SolutionWhat is the value of gravitational constant G=6.67×10−11Nm2/kg2 in CGS units?
View Solution
Knowledge Check
Two point masses m and 2m are kept at points A and B as shown. E represents magnitude of gravitational field strength and V the gravitational potential. As we move from A to B
 AE will first decreases then increasesBE will first increases then decreasesCV will first decreases then increasesDV will first increases then decrease
AE will first decreases then increasesBE will first increases then decreasesCV will first decreases then increasesDV will first increases then decreaseThe bodies of mass m and 4m are placed at a distance of 6m apart. P is the point on the line joining two bodies where gravitational field is zero. The gravitational potential at this point is
A−32GmB−52GmC−92GmD−72Gm
Give the order of the following :
Gravitational constant
(6.67×10−11N−m2/kg2)
View SolutionA point mass body of mass 2kg is placed at a distance 20cm from one end of a uniform rod of length 2m and mass 10kg. Calculate
(i) gravitational intensity at the location of point mass body due to the rod.
(ii) gravitational force on the body due to the rod. Use G=6.67×10−11Nm2kg−2.
View SolutionThree masses of 1 kg, 2kg, and 3 kg are placed at the vertices of an equilateral triangle of side 1m. Find the gravitational potential energy of this system
(Take, G=6.67×10−11N-m−2kg−2)
View SolutionThree masses of 1kg, 2kg, and 3kg, are placed at the vertices of an equilateral traingle of side 1m. Find the gravitational potential energy of this system.
Take G=6.67×10−11N−m2/kg2.
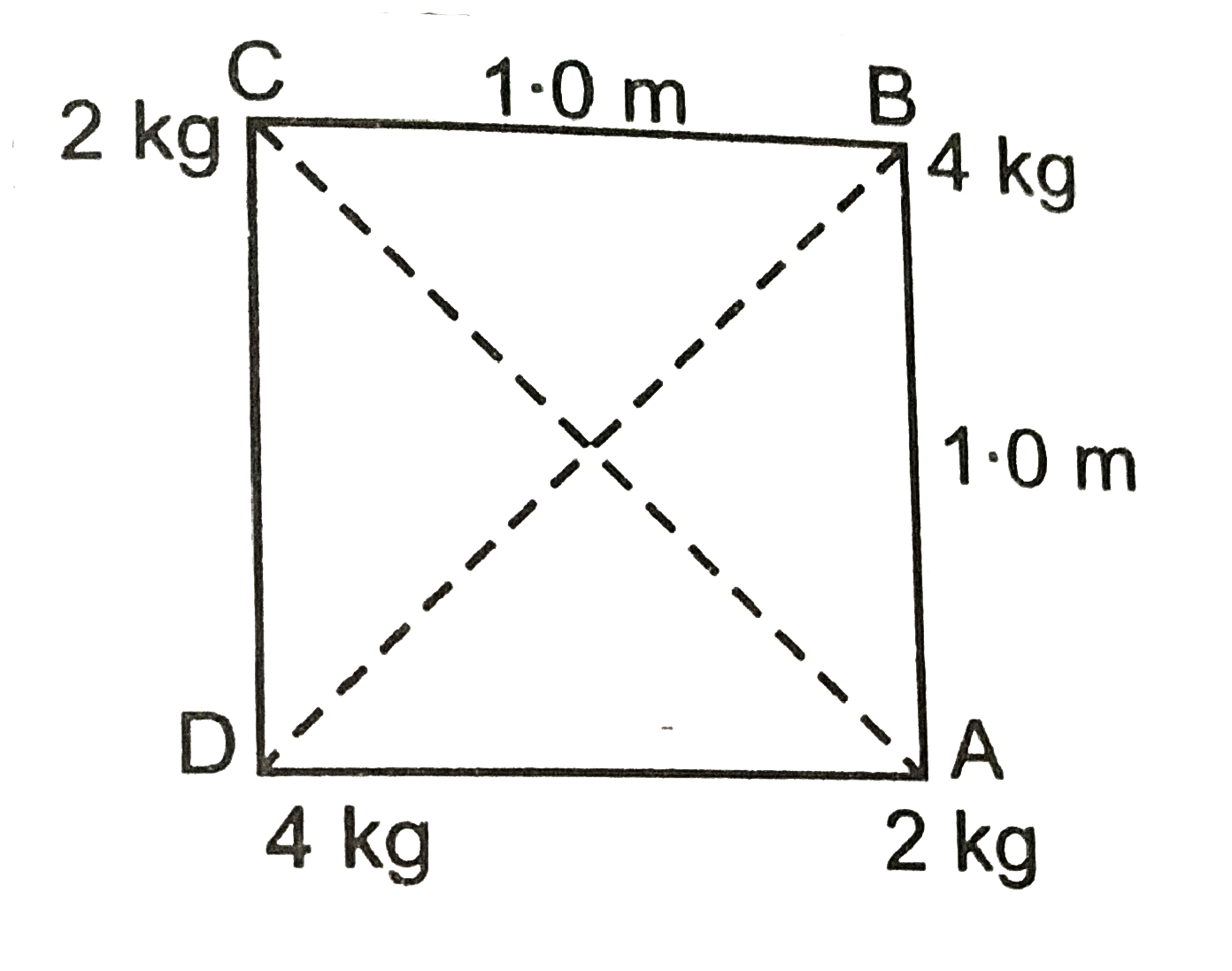
View SolutionFour point mass bodies of masses as shown in Fig. are placed at the vertices of a square ABCD, gravitational force on the body at A. Given, G=6.6×10−11Nm2kg−2
View SolutionA(1,4)andB(4,8) are two points. P is a point on AB such that AP=AB+BP. If AP=10, find the coordinates of P.
View Solution
