Two monochromatic beam A and B of equal intensity I, hit a screen. The number of photons hitting the screen by beam A is twice that by beam B. Then what inference can you make about their frequencies?
To solve the problem, we will analyze the relationship between the number of photons, intensity, and frequency of the two beams of light, A and B.
1. Understanding the Given Information:
- We have two beams of light, A and B, both with equal intensity I.
- The number of photons hitting the screen from beam A (nA) is twice that from beam B (nB).
- Therefore, we can write:
nA=2nB
2. Using the Definition of Intensity:
- The intensity I of a beam of light can be expressed as the energy per unit area per unit time.
- Mathematically, we can express the intensity of each beam as:
IA=nA⋅EA
IB=nB⋅EB
- Here, EA and EB are the energies of the photons from beams A and B, respectively.
3. Relating Energy to Frequency:
- The energy of a photon is given by the equation:
E=hν
where h is Planck's constant and ν is the frequency of the light.
- Thus, we can rewrite the energies for beams A and B:
EA=hνA
EB=hνB
4. Substituting Energies into the Intensity Equations:
- Now substituting the expressions for energy into the intensity equations, we have:
IA=nA⋅(hνA)
IB=nB⋅(hνB)
5. Setting Intensities Equal:
- Since the intensities are equal (IA=IB), we can set the two equations equal to each other:
nA⋅(hνA)=nB⋅(hνB)
6. Canceling Planck's Constant:
- We can cancel h from both sides (assuming h≠0):
nA⋅νA=nB⋅νB
7. Substituting the Relationship of Number of Photons:
- Now substituting nA=2nB into the equation:
(2nB)⋅νA=nB⋅νB
8. Simplifying the Equation:
- Dividing both sides by nB (assuming nB≠0):
2νA=νB
9. Final Relation Between Frequencies:
- Rearranging gives us the relationship between the frequencies:
νB=2νA
Conclusion:
The frequency of beam B is twice that of beam A.
- We have two beams of light, A and B, both with equal intensity I.
- The number of photons hitting the screen from beam A (nA) is twice that from beam B (nB).
- Therefore, we can write:
nA=2nB
- The intensity I of a beam of light can be expressed as the energy per unit area per unit time.
- Mathematically, we can express the intensity of each beam as:
IA=nA⋅EA
IB=nB⋅EB
- Here, EA and EB are the energies of the photons from beams A and B, respectively.
- The energy of a photon is given by the equation:
E=hν
where h is Planck's constant and ν is the frequency of the light.
- Thus, we can rewrite the energies for beams A and B:
EA=hνA
EB=hνB
- Now substituting the expressions for energy into the intensity equations, we have:
IA=nA⋅(hνA)
IB=nB⋅(hνB)
- Since the intensities are equal (IA=IB), we can set the two equations equal to each other:
nA⋅(hνA)=nB⋅(hνB)
- We can cancel h from both sides (assuming h≠0):
nA⋅νA=nB⋅νB
- Now substituting nA=2nB into the equation:
(2nB)⋅νA=nB⋅νB
- Dividing both sides by nB (assuming nB≠0):
2νA=νB
- Rearranging gives us the relationship between the frequencies:
νB=2νA
The frequency of beam B is twice that of beam A.
Topper's Solved these Questions
- DUAL NATURE OF RADIATION AND MATTERBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - LONG ANSWER TYPE QUESTION
5 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
5 Videos
- CURRENT ELECTRICITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Very Short Answer Type Question.
20 Videos
- ELECTRIC CHARGES AND FIELDBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDEXERCISE - Long Answer Type Question
6 Videos
Similar Questions
Two coherent monochromatic light beams of intensities I and 4I are superposed. The maximum and minimum possible intensities in the resulting beam are
View SolutionTwo coherent monochromatic light beams of intensities I and 4I are superposed. The maximum and minimum possible intensities in the resulting beam are
View SolutionTwo coherent monochromatic light beams of intensities I and 4I are superposed. The maximum and minimum possible intensities in the resulting beam are
View SolutionTwo separate monochromatic light beams A and B of the same intensity (energy per unit area per unit time) are falling normally on a unit area of a metallic surface. Their wavelength are λA and λB respectively. Assuming that all the the incident light is used in ejecting the photoelectrons, the ratio of the number of photoelectrons from beam A to that from B is
View SolutionTwo beams of ligth having intensities I and 4I interface to produce a fringe pattern on a screen. The phase difference between the beams is π2 at point A and π at point B. Then the difference between the resultant intensities at A and B is
View SolutionWhen two coherent monochromatic light beams of intensities I and 4I are superimposed, the ratio between maximum and minimum intensities in the resultant beam is
View SolutionA monochromatic beam of light is used for the formation of fringes on a screen by illuminating the two slits in the Young,s double slit interference experiment. When a thin film of mic is interposed in the path of one of the interfering beams
View SolutionFig. shows a thin beam of white light from a source S striking on one face of a prism.
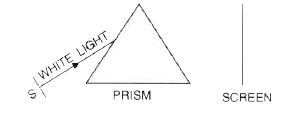
(a) Complete the diagram to show the effect of the prism on the beam and to show what is seen on the screen.
(b) If a slit is placed in between the prism and the screen to pass only the light of green colour, what will you then observe on the screen?
(c) What conclusion do you draw from the observation in part (b) above?
View SolutionA parallel beam of uniform, monochromatic light of wavelength 6600 Å has an intensity of 900Wm−2. The number of photons in 1mm2 of this radiation are 1×10X then find out value of X.
View Solution_E01_107_Q01.png)
A narrow monochromatic beam of light of intensity I is incident on a galss plate as shown in figure. Another identical glass plate is kept close to the first one & parallel to it. Each glass plate reflects 25% of the light incident on it % transmits the remaining find the ratio of the minimum & the maximum intensities in the interference pattern formed by the two beams obtained after one reflection at each plate.
View Solution
