Two small satellies move in a circular orbits around the earth, at disatnce r and (r+dr) from the centre of the earth. Their time periods of rotation ate T and T+dT(Δr<<br,ΔT<<T). Then
ΔT=32TΔrr
ΔT=−32TΔrr
ΔT=23TΔrr
ΔT=TΔrr
The correct Answer is:A
As T2∝r3 or T2=Kr3 ..(i)
Differentating it, we have
2TΔT=3Kr2Δr ..(ii)
Dividing (ii) by (i), we have
2TΔTT2=3kr2ΔrKr3 or ΔT=32TΔrr
Similar Questions
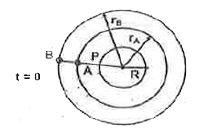
Two satellite A and B are travelling in the same plane and same sense in circular orbit around the earth at an atitude rA and rB from the centre of the earth respectively. If at time t=0 the satellite are aligned as shown in the figure, knowing that the radius of the earth R. Determine the time at which again the satellite will be aligned as they were at t=0
View SolutionA satellite takes years to move around the earth in its permissible orbit of radius R. the period when it revolves round the earth in an orbit of radius 2R is
View Solution
Knowledge Check
Two small satellites are moving in circular orbits around the earth at a distance R and R+ΔR from the centre of the earth. If their time period of rotation are T and T+ΔT respectively, then
AΔT=TΔRRBΔT=3TΔRRCΔT=32TΔRRDΔT=23TΔRRTwo small satellites are moving in circular orbits around the earth at a distance R and R+ΔR from the centre of the earth. If their time period of rotation are T and T+ΔT respectively, then
AΔT=TΔRRBΔT=3TΔRRCΔ=32TΔRRDΔT=23TΔRRThe time period of a satellite in a circular orbit around the earth is T . The kinetic energy of the satellite is proportional to T−n . Then, n is equal to :
A12B23C43D32
A satellite of mass is in a stable circular orbit around the earth at an altitude of about 100 km. If M is the mass of the earth, R its radius and g the acceleration due to gravity, then the time period T of the revolution of the satellite is
View SolutionA satellite is revolving round the earth in an orbit of radius r with time period T. If the satellite is revolving round the earth in an orbit of radius r+Δr(Δr<<r) with time period T+ΔT(ΔT<<T) then.
View SolutionThe acceleration due to gravity is g at a point distant r from the centre of earth of radius R. If r<R, then
View SolutionThe time period of a satellite in a circular orbit of radius R is T. The radius of the orbit in which time period is 8 T is
View SolutionTwo satellites revolve round the earth with orbital radii 4R and 16 R, if the time period of first satellite is T then that of the other is
View Solution
