Two soap bubbles coalesce to form a single large drop. Match the following.
∣∣
∣
∣
∣
∣
∣
∣
∣
∣∣Table-1Table-2(A)Surface energy in the(P)increaseprocess will(B)Temperature of the(Q)decreasedrop will(C)Pressure inside the(R)remain samesoap bubble will∣∣
∣
∣
∣
∣
∣
∣
∣
∣∣
The correct Answer is:A, B, C
To solve the problem of matching the effects of two soap bubbles coalescing into a single larger drop, we can analyze each statement in Table 1 and determine the corresponding effects in Table 2.
1. Understanding Coalescence: When two soap bubbles coalesce, they combine to form a single larger bubble. This process involves changes in surface area, surface energy, pressure, and temperature.
2. Surface Energy:
- When two bubbles combine, the total surface area decreases because the larger bubble has a smaller surface area relative to its volume compared to the two smaller bubbles.
- Since surface energy is directly related to surface area, the surface energy of the system will decrease.
- Match A with R: Surface energy in the process will decrease (A) → (R) decrease.
3. Temperature of the Drop:
- The volume of the gas remains constant during the coalescence. However, the pressure inside the larger bubble decreases due to the larger radius.
- According to the ideal gas law (PV=nRT), if the pressure decreases while the volume remains constant, the temperature must also decrease.
- Match B with Q: Temperature of the drop will decrease (B) → (Q) decrease.
4. Pressure Inside the Soap Bubble:
- As mentioned earlier, the pressure inside the larger bubble is lower than that of the smaller bubbles due to the larger radius.
- Therefore, the pressure inside the soap bubble will decrease.
- Match C with Q: Pressure inside the soap bubble will decrease (C) → (Q) decrease.
Final Matching:
- A → R (Surface energy will decrease)
- B → Q (Temperature will decrease)
- C → Q (Pressure will decrease)
Summary of Matches:
- A (Surface energy in the process will) → R (decrease)
- B (Temperature of the drop will) → Q (decrease)
- C (Pressure inside the soap bubble will) → Q (decrease)
- When two bubbles combine, the total surface area decreases because the larger bubble has a smaller surface area relative to its volume compared to the two smaller bubbles.
- Since surface energy is directly related to surface area, the surface energy of the system will decrease.
- Match A with R: Surface energy in the process will decrease (A) → (R) decrease.
- The volume of the gas remains constant during the coalescence. However, the pressure inside the larger bubble decreases due to the larger radius.
- According to the ideal gas law (PV=nRT), if the pressure decreases while the volume remains constant, the temperature must also decrease.
- Match B with Q: Temperature of the drop will decrease (B) → (Q) decrease.
- As mentioned earlier, the pressure inside the larger bubble is lower than that of the smaller bubbles due to the larger radius.
- Therefore, the pressure inside the soap bubble will decrease.
- Match C with Q: Pressure inside the soap bubble will decrease (C) → (Q) decrease.
- A → R (Surface energy will decrease)
- B → Q (Temperature will decrease)
- C → Q (Pressure will decrease)
- A (Surface energy in the process will) → R (decrease)
- B (Temperature of the drop will) → Q (decrease)
- C (Pressure inside the soap bubble will) → Q (decrease)
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Comprehension
32 Videos
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
Similar Questions
A cube is floating in a liquid as shown in figure. Match the following.
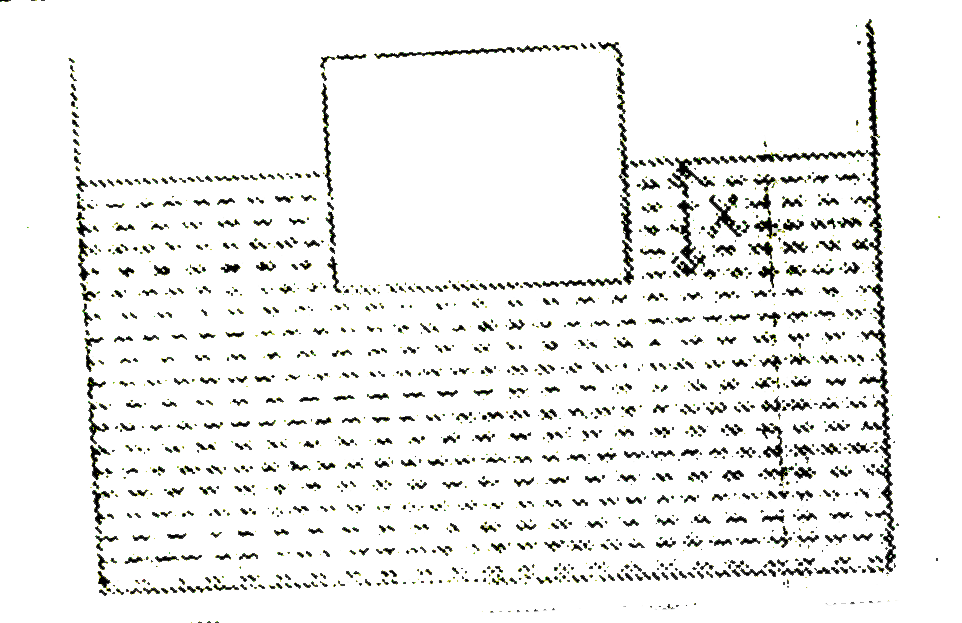
∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣Table-1Table-2(A)If density liquid(P)increasedecreases x will(B)If size of cube is(Q)decreaseincreased x will(C)If the whole system is(R)remain sameaccelerated upwards xwill∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣
View SolutionThere are two point A and B inside a liquid as shown in figure. Now the vessel starts moving upwards with an acceleration a. Match the following.
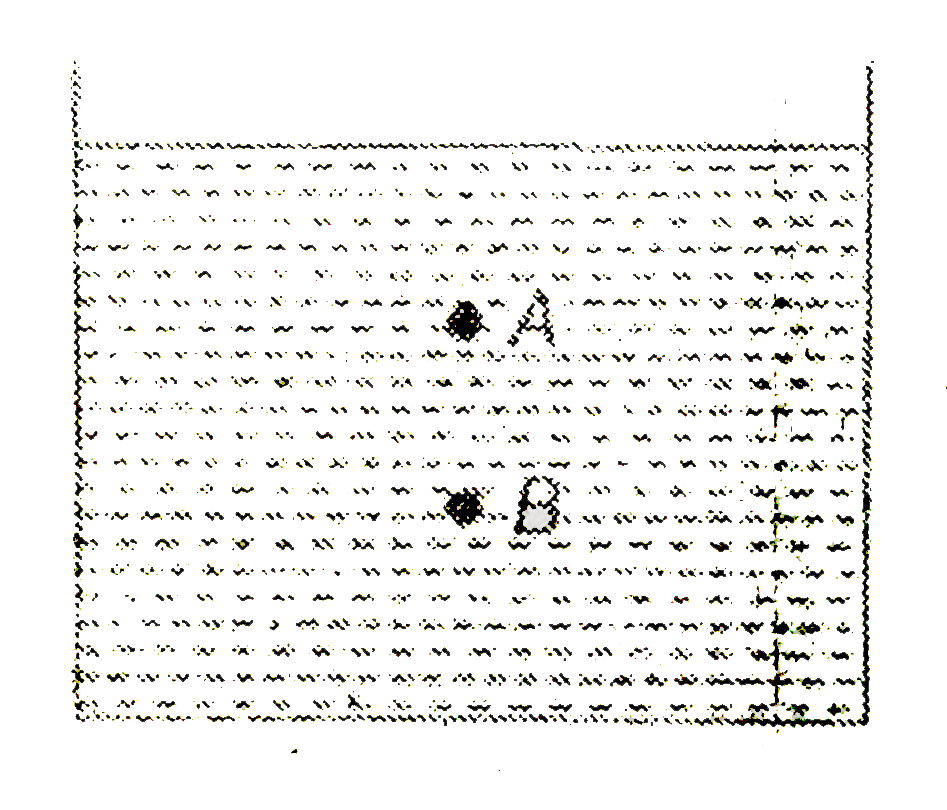
∣∣ ∣ ∣ ∣ ∣ ∣ ∣∣Table-1Table-2(A)Pressure at B will(P)increase(B)Pressure difference(Q)decreasebetween A and B will(C)Upthrust on an object(R)remain sameinside the verssel will∣∣ ∣ ∣ ∣ ∣ ∣ ∣∣
View SolutionA solid is immersed completely in a liquid. The temperature coefficients of volume expansion of solid and the liquid are γ1 and γ2(<γ1). If temperatures of both are increased, then match the following.
∣∣ ∣ ∣ ∣ ∣ ∣∣Table-1Table-2(A)upthrust on the solid will(P)increase(B)apparent weight of the solid will(Q)decrease(C)fraction of volume immersed in(R)remain samethe liquid if allowed to float will∣∣ ∣ ∣ ∣ ∣ ∣∣
View SolutionA tube is inverted in a mercury vessel as shown in figure. If pressure P is increased, then
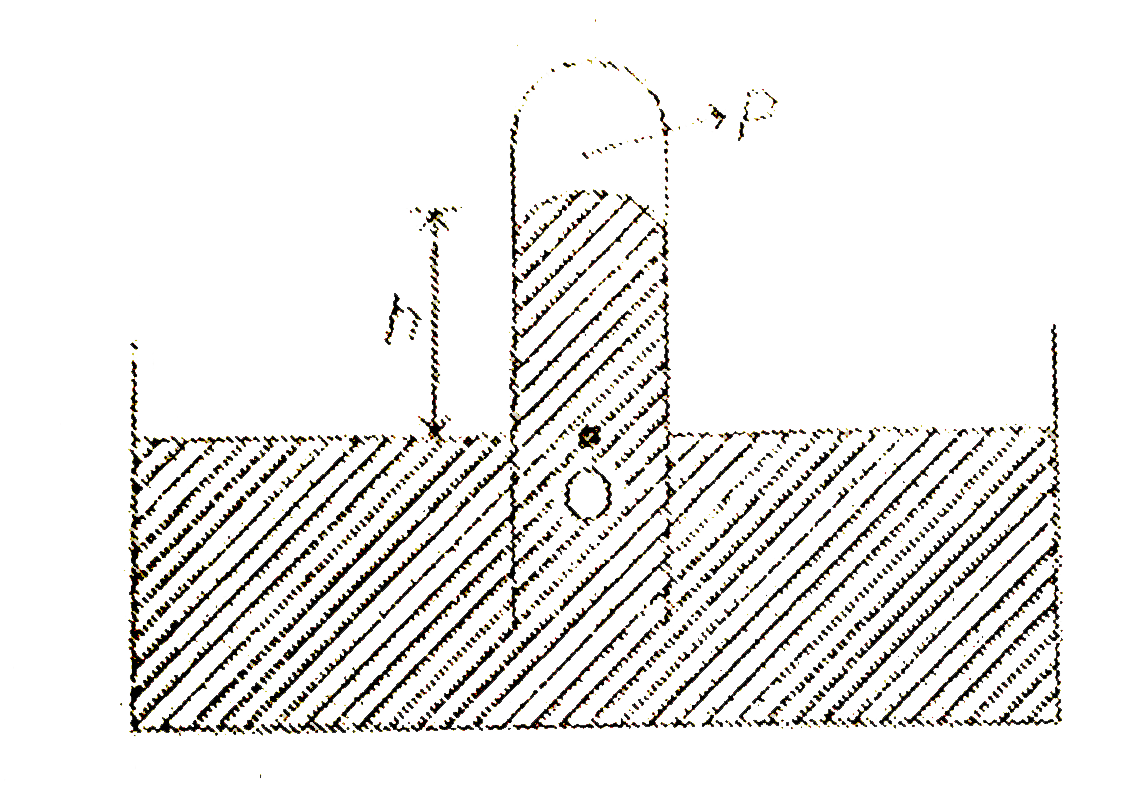
∣∣ ∣ ∣ ∣ ∣ ∣∣Table-1Table-2(A)Height h(P)will increase(B)Pressure at O(Q)will decrease(C)Pressure at 1 cm(R)will remain sameabove O∣∣ ∣ ∣ ∣ ∣ ∣∣
View SolutionMatch the following.
∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣Table-1Table-2(A)Coefficient of viscosity(P)[M2L−1T−2](B)Surface tension(Q)[ML0T−2](C)Modulus of elasticity(R)[ML−1T−2](D)Energy per unit volume(S)Noneof a fiuid∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣
View SolutionMatch the following
∣∣ ∣ ∣ ∣ ∣ ∣ ∣∣Table-1Table-2(A)Stress×Strain(P)J(B)YAl(Q)N/m(C)Yl3(R)J/m3(D)FlAY(S)m∣∣ ∣ ∣ ∣ ∣ ∣ ∣∣
View SolutionMatch the following
Column-IColumn-II(A)Enthalpy(p)Extensive property(B)Entropy(q)H - TS(C)Free energy(r)E + PV(D)Pressure(s)Intensive property
View SolutionMatch the following
∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣ Table-1 Table-2(A) Steel(P) Young's modulus of elasticity(B) Water(Q) Bulk modulus of elasticity(C) Hydrogen gas filled(R) sheer modulus of in a chamber elasticity∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣
View SolutionIn the figures shown, match the following

∣∣ ∣ ∣ ∣ ∣∣Table-1Table-2(A)x=2h, y=h(P)R=2√3h(B)x=h, y=3h(Q)R=2√2h(C)x=3h, y=h(R)R=2h∣∣ ∣ ∣ ∣ ∣∣
View SolutionMatch the following
Column-IColumn-II(A)Isothermal process(p)Temperature changes(B)Isobaric process(q)Pressure changes(C)Isochoric process(r)Volume change(D)Cyclic process(s)Initial and final states are same
View Solution
