Using the definition of the group velocity u, derive Rayleigh's fromula (5.5d). Demonstrate that in the vicinity of λ=λ' the velocity u is equal to the segment υ' cut by the tangent of the curve υ(λ) at the point λ' (Fig.)
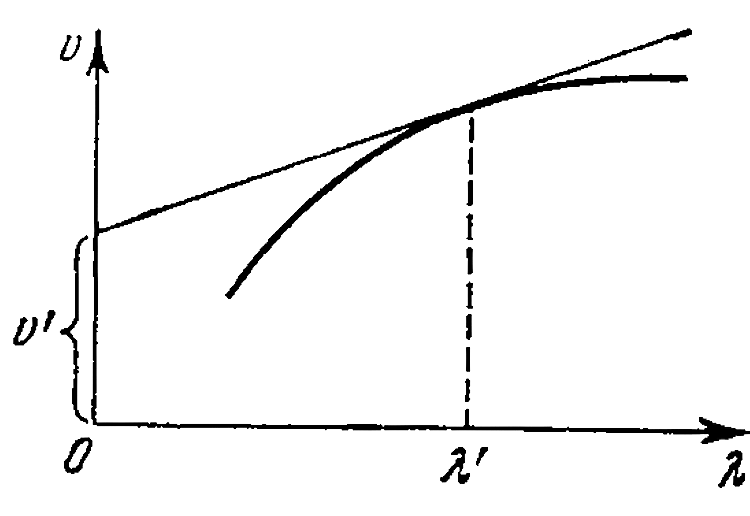
By definition
u=dωdk=ddk(vk) as ω=vk=v+kdvdk
Now k=2πλ so dk=−2πλ2dλ
Thus u=v−λdvdλ.
Its interpretation is the following:
(dvdλ)λ−λ' is the slope of the v−λ curve at λ=λ'.
Thus as is obvious from the diagram
v'=v(λ')−λ'(dvdλ)λ=λ' is the group velocity for λ=λ'

Topper's Solved these Questions
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Optics Of Moving Sources
22 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Thermal Equation
47 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Polarization Of Light
43 Videos
- MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - MECHANICSEXERCISE - Mechanics Problems
92 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
Similar Questions
Let (a,b) and (λ,u) be two points on the curve y=f(x). If the slope of the tangent to the curve at (x,y) be ?
View SolutionIf the perpendicular distance of the point (65,8) from the Y-axis is 5λ units, then λ is equal to
View SolutionIf the perpendicular distance of the point (6,5,8) from the Y-axis is 5λ units, then λ is equal to
View SolutionIf the perpendicular distance of the point (6,5,8) from the Y-axis is 5λ units, then λ is equal to
View SolutionIf the perpendicular distance of the point (65,8) from the y-axis is 5λ units, then λ is equal to ___
View SolutionA metallic ring of field. If z is the radius l (ring being horizontal is falling under gravity in a region haivng a magnetic field. If z is the vertical direction, the z-component of magnetic field is Bz=B0(1+λz). If R the resistance of the ring and if the ring falls with a velocity υ, find the energy lost in the resistance If the ring has reached a constant velocity, use the conservation of energy to determine υ in terms of m, B, λ and acceleration due to gravity g.
View SolutionA metallic ring of field. If z is the radius l (ring being horizontal is falling under gravity in a region haivng a magnetic field. If z is the vertical direction, the z-component of magnetic field is Bz=B0(1+λz). If R the resistance of the ring and if the ring falls with a velocity υ, find the energy lost in the resistance If the ring has reached a constant velocity, use the conservation of energy to determine υ in terms of m, B, λ and acceleration due to gravity g.
View SolutionA spectrograph's trihedral prism is manufactured from glass whose refractive index varies with wavelength as n=A+B/λ2, where A and B are constats, with B being equal to 0.010μm2. Making use of the formula from the foregoing problem, find:
(a) how the resolving power of the prism depends on λ, calculate the value of λ/δλ in the vicinity of λ1=434nm and λ2=656nm if the width of the prism's base is b=5.0cm,
(b) the width of the prism's base capacble of resolving the yellow doublet of sodium (589.0 and 589.6nm).
View SolutionThe velocity υ of sound waves in a medium may depend upon modulus of elasticity (E ), density (d) and wavelength (λ) of of the waves. Use method of dimensions to deerive the formula for υ.
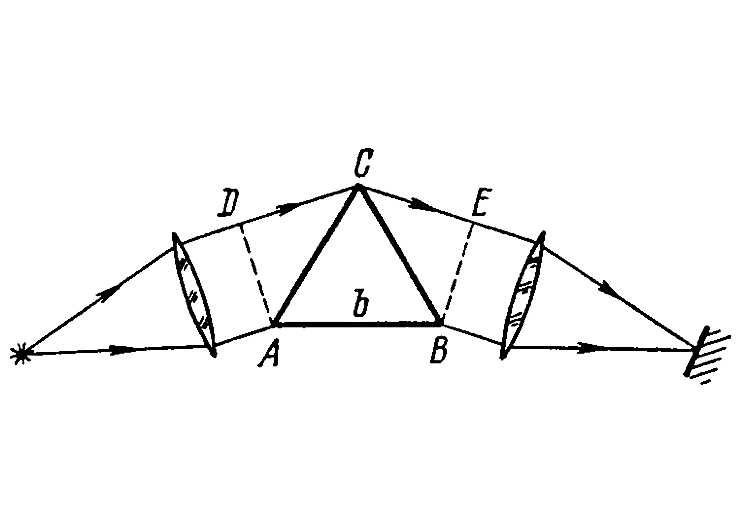
View SolutionThe ultimate resolving power λ/δλ of the spectrograph's trihedral prism is determined by diffraction of light at the prism edges (as in the case of a slit). When the prism is oriented to the least deviation angle in accordance with Rayleigh's criterion,
λ/δλ=b|dn/dλ|,
where b is the width of the prism's base (Fig.) and dn/dλ is the dispersion of its material. Derive this formula.
View Solution
