Varun has been selected by his School to design logo for Sports Day T-shirts for students and staff . The logo design is as given in the figure and he is working on the

fonts and different colours according to the theme. In given figure, a circle with centre O is inscribed in a ΔABC, such that it touches the sides AB, BC and CA at points D, E and F respectively. The lengths of sides AB, BC and CA are 12 cm, 8 cm and 10 cm respectively.
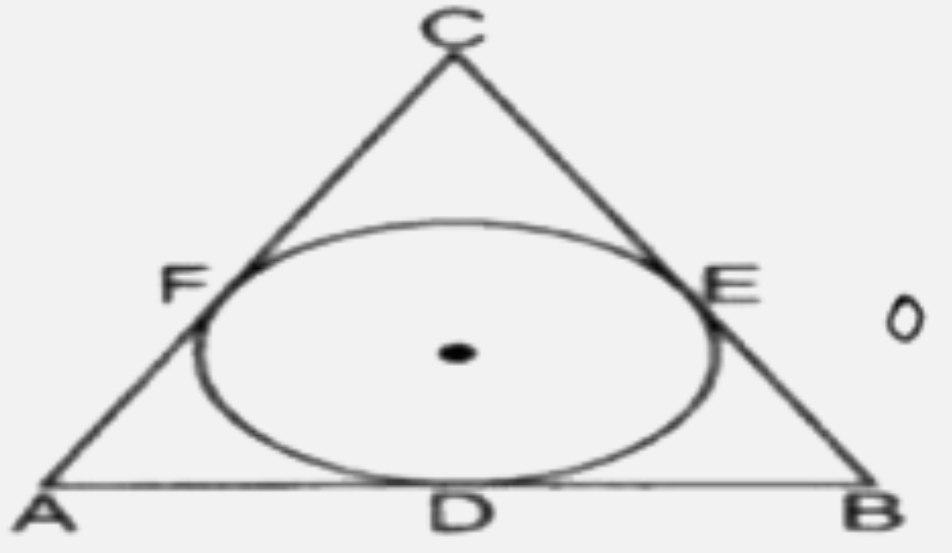
if radius of the circle is 4 cm , Find the area of ΔAOB.
20
36
24
48
The correct Answer is:C
Step by step video & image solution for Varun has been selected by his School to design logo for Sports Day T-shirts for students and staff . The logo design is as given in the figure and he is working on the fonts and different colours according to the theme. In given figure, a circle with centre O is inscribed in a DeltaABC, such that it touches the sides AB, BC and CA at points D, E and F respectively. The lengths of sides AB, BC and CA are 12 cm, 8 cm and 10 cm respectively. if radius of the circle is 4 cm , Find the area of Delta AOB. by Physics experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - CBSE MODEL PAPERCHAPTER - QUESTION BANK 2021EXERCISE - SOME APPLICATION OF TRIGONOMETRY (Case Study 1)5videos
QUESTION BANK 2021
- BOOK - CBSE MODEL PAPERCHAPTER - QUESTION BANK 2021EXERCISE - SOME APPLICATION OF TRIGONOMETRY(Case Study 2)5videos
QUESTION BANK 2021
- BOOK - CBSE MODEL PAPERCHAPTER - QUESTION BANK 2021EXERCISE - CIRCLES (Case Study 1)4videos
QUESTION BANK 2021
- BOOK - CBSE MODEL PAPERCHAPTER - PRACTICE PAPER 2022EXERCISE - Multiple Choice Questions60videos
PRACTICE PAPER 2022
- BOOK - CBSE MODEL PAPERCHAPTER - SAMPLE PAPER (MATHEMATICS STANDARD)EXERCISE - PART - B16videos
SAMPLE PAPER (MATHEMATICS STANDARD)
Similar Questions
Varun has been selected by his School to design logo for Sports Day T-shirts for students and staff . The logo design is as given in the figure and he is working on the

fonts and different colours according to the theme. In given figure, a circle with centre O is inscribed in a ΔABC, such that it touches the sides AB, BC and CA at points D, E and F respectively. The lengths of sides AB, BC and CA are 12 cm, 8 cm and 10 cm respectively.

Find area of ΔABC.View SolutionVarun has been selected by his School to design logo for Sports Day T-shirts for students and staff . The logo design is as given in the figure and he is working on the

fonts and different colours according to the theme. In given figure, a circle with centre O is inscribed in a ΔABC, such that it touches the sides AB, BC and CA at points D, E and F respectively. The lengths of sides AB, BC and CA are 12 cm, 8 cm and 10 cm respectively.

Find the lenght of BE .View Solution
Knowledge Check
- Question 1 - Select One
The in circle of ΔABC touches the sides BC, CA and AB at D, E and F respectively. Then AF + BD + CE is equal to :
A12 (Perimeter of Δ ABC)B13 (Perimeter of Δ ABC)C14 (Perimeter of Δ ABC)DPerimeter of Δ ABC
Varun has been selected by his School to design logo for Sports Day T-shirts for students and staff . The logo design is as given in the figure and he is working on the

fonts and different colours according to the theme. In given figure, a circle with centre O is inscribed in a ΔABC, such that it touches the sides AB, BC and CA at points D, E and F respectively. The lengths of sides AB, BC and CA are 12 cm, 8 cm and 10 cm respectively.

Find the length of AD.View SolutionVarun has been selected by his School to design logo for Sports Day T-shirts for students and staff . The logo design is as given in the figure and he is working on the

fonts and different colours according to the theme. In given figure, a circle with centre O is inscribed in a ΔABC, such that it touches the sides AB, BC and CA at points D, E and F respectively. The lengths of sides AB, BC and CA are 12 cm, 8 cm and 10 cm respectively.

Find the lenght of CF.View SolutionIn the given figure, a circle inscribed in a triangle ABC touches the sides AB, BC and CA at points D, E and F respectively. If AB = 14 cm, BC = 8 cm and CA = 12 cm. Find the lengths AD, BE and CF.
View SolutionIn the given figure, a circle inscribed in a triangle ABC, touches the sides AB, BC and AC at points D, E and F respectively. If AB= 12 cm, BC= 8 cm and AC = 10 cm, find the lengths of AD, BE and CF.
View SolutionA circle touch sides of A ΔABC i.e. AB, BC, AC at P, Q, R repectively length of side AB, BC and CA are 10, 12 and 14. Find AP
View SolutionThe incircle of an isoceles triangle ABC, with AB=AC, touches the sides AB,BC and CA at D,E and F respecrively. Prove that E bisects BC.
View SolutionThe incircle of an isosceles triangle ABC, with AB=AC, touches the sides AB, BC, CA at D,E and F respectively. Prove that E bisects BC.
View Solution

