Question

What is the ground state of an atom ? And what is the ionization energy and excitation energy of a hydrogen atom ?

Explanation

Total energy of electron in atom is
`E_(n)=-(mZ^(2)e^(4))/(8n^(2)h^(2)epsi_(0)^(2))`
`:. E_(n)=-(13.6Z^(2))/(n^(2))eV`
Negative sign indicates that as the value of .n. increases the negative `E_(n)` decreases that is energy increases that is the energy of the electron increases gradually in far orbits from nucleus.
When electron is revolving in an orbit closest to the nucleus mean in n = 1 orbit, the absolute value of the energy (maximum negative value) is smaller. Such a lowest state of the atom is called the ground state.
The energy of electron orbiting in orbit of Bohr radius `a_(0)` is ,
`E_(1)=(13.6Z^(2))/(n^(2))eV`
For hydrogen Z=1,n=1
`:. E_(1)=-13.6eV`
Therefore, the minimum energy required to free the electron from the ground state of hydrogen atom is 13.6 eV is called the ionization energy.
At room temperature, most of the hydrogen atoms are in ground state. When a hydrogen atom receives energy by processes, the atom may acquire sufficient energy to raise the electron to higher energy states.
The atom is then said to be in an excited state. The energy required to excite an electron in hydrogen atom to its first excited state (n = 2)
`E_(2)-E_(1)=-(13.6)/(2^(2))-(-(3.6)/(1^(2)))`
`=(13.6-(13.6)/(4))eV` energy needed
`=13.6-3.4=10.eV`
And the energy required to bring an electron in H atom from ground state to second excited state (n=3) is
`E_(3)-E_(1)=-(13.6)/(3^(2))-(-(13.6)/(1^(2)))`
`=13.6-1.51`
`=12.09eV`
Hence, the energy required to bring an electron in H atom from ground state to second excited state is 12.09 eV.
Similarly, the energy required to bring electron in third excited state and fourth excited state and infinite excited state are 12.75 eV, 13.06 eV and 13.6 eV respectively.
If the electron returns from a excited state to a low energy state, a photon is emitted in this process.
Thus, as the excitation of hydrogen atom increases that is as n increases, the value of minimum energy required to free the electron from the excited atom decreases.
Thus, from equation `E_(n)=-(13.6)/(n^(2))eV`,the energy level diagram for stationary states of a hydrogen atom is shown in figure.

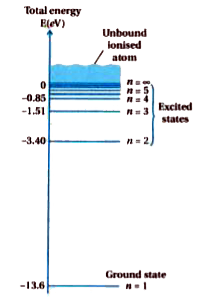
The energies corresponding to different orbits as shown by horizontal lines on vertical axis.
The energy levesl of the hydrogen atom are shown by horizontal line.
As the quantum nuber increases the energy difference between the two successive energy levels decreases.