What is the perpendicular distance between the force and the turning point if a force of 25 N produces a moment of force of 12.5 Nm ?
To find the perpendicular distance between the force and the turning point when a force of 25 N produces a moment of force (torque) of 12.5 Nm, we can use the relationship between torque, force, and distance.
1. Understand the relationship: The moment of force (torque) is given by the formula:
τ=d×F×sin(θ)
where:
- τ is the torque (moment of force),
- d is the perpendicular distance from the line of action of the force to the pivot point,
- F is the force applied,
- θ is the angle between the force and the line from the pivot to the point of application of the force.
2. Identify the given values: From the problem, we have:
- Torque (τ) = 12.5 Nm,
- Force (F) = 25 N,
- Since the distance is perpendicular, θ=90∘ and sin(90∘)=1.
3. Rearrange the formula: Since sin(90∘)=1, we can simplify the torque formula to:
τ=d×F
Rearranging gives us:
d=τF
4. Substitute the known values: Now, plug in the values for torque and force:
d=12.5Nm25N
5. Calculate the distance: Performing the division:
d=0.5m
6. Convert to centimeters: Since 1 meter = 100 centimeters, we convert the distance:
d=0.5m=50cm
7. Final answer: Therefore, the perpendicular distance between the force and the turning point is:
The perpendicular distance is 50cm.
τ=d×F×sin(θ)
where:
- τ is the torque (moment of force),
- d is the perpendicular distance from the line of action of the force to the pivot point,
- F is the force applied,
- θ is the angle between the force and the line from the pivot to the point of application of the force.
- Torque (τ) = 12.5 Nm,
- Force (F) = 25 N,
- Since the distance is perpendicular, θ=90∘ and sin(90∘)=1.
τ=d×F
Rearranging gives us:
d=τF
d=12.5Nm25N
d=0.5m
d=0.5m=50cm
The perpendicular distance is 50cm.
Topper's Solved these Questions
- FORCE AND PRESSURE BOOK - ICSECHAPTER - FORCE AND PRESSURE EXERCISE - EXERCISE 3.1 (FILL IN THE BLANK)
5 Videos
- FORCE AND PRESSURE BOOK - ICSECHAPTER - FORCE AND PRESSURE EXERCISE - EXERCISE 3.1 (TRUE OR FALSE)
10 Videos
- ENERGYBOOK - ICSECHAPTER - ENERGYEXERCISE - THEME ASSIGNMENT
4 Videos
- HEAT TRANSFER BOOK - ICSECHAPTER - HEAT TRANSFER EXERCISE - Picture Study
2 Videos
Similar Questions
If the perpendicular distance between the pivot and the point of application of the force is more, then torque is ......(more/less).
View Solution.................. (Pressure/Torque) is equal to the product of the force applied and the perpendicular distance between the pivot and the point of application of the force.
View Solution
Knowledge Check
If the distance between two point particles is doubled, then the gravitational force between them
Adecreases by a factor of 4Bdecreases by a factor of 2Cincreases by a factor of 2Dincreases by a factor of 4
What is meant by the term 'moment of force' ?
View SolutionWhat is the difference between London dispersion forces and dipole-dipole forces ?
View SolutionWhat is the work done by a force when the force is:
Normal to the displacement produced.
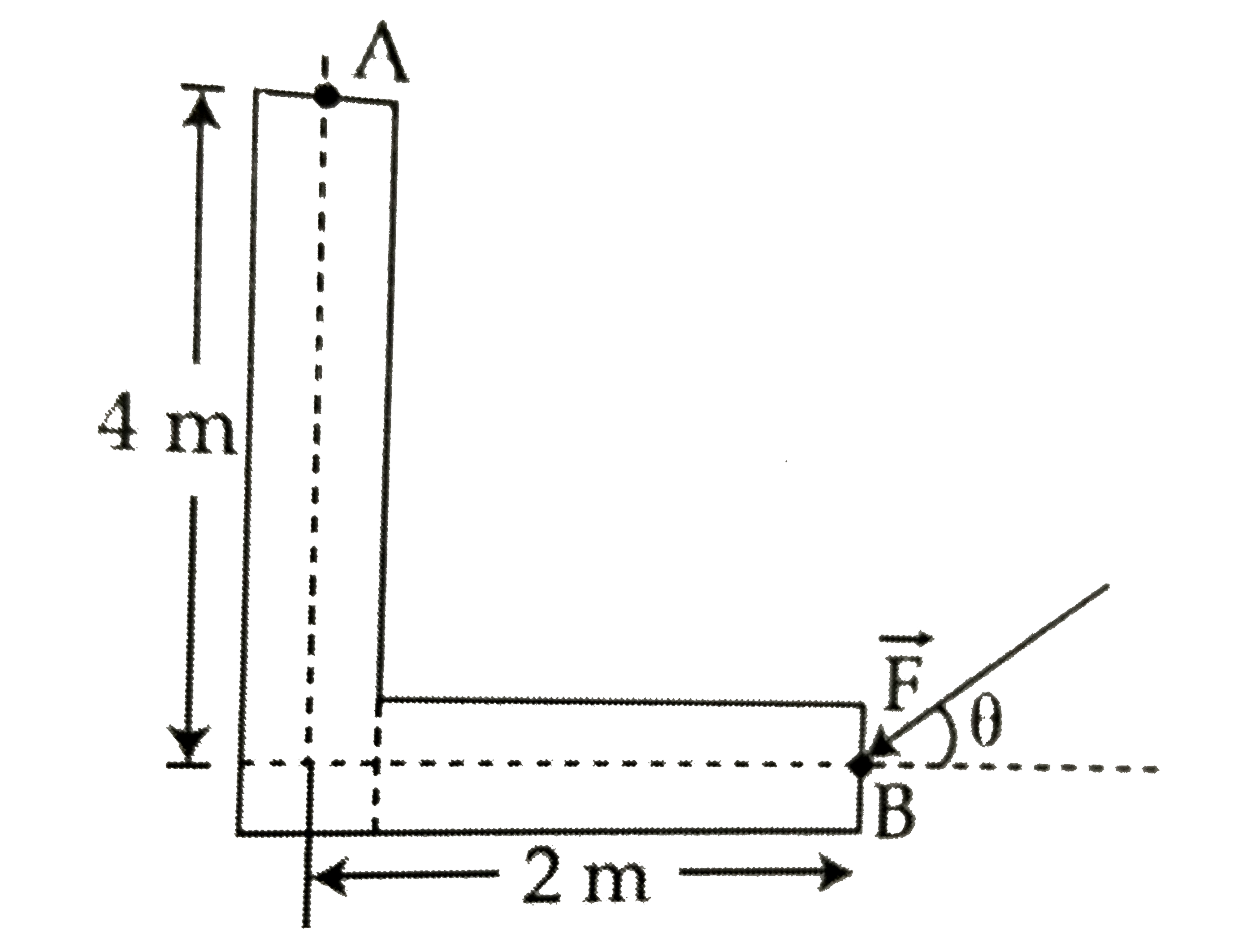
View SolutionA force of 40 N acts on a point B at the end of the L-shaped object, as shown in the figure. The angle θ that will produce maximum moment of the force about point A is given by :
View SolutionCalculate the angle between a 20 N force and a 30 N force so that their resultant is 40 N
View SolutionThe dimensional formula of "Moment of force" is
View SolutionPoint out the difference between London dispersion forces and dipole-dipole forces.
View Solution
