Question

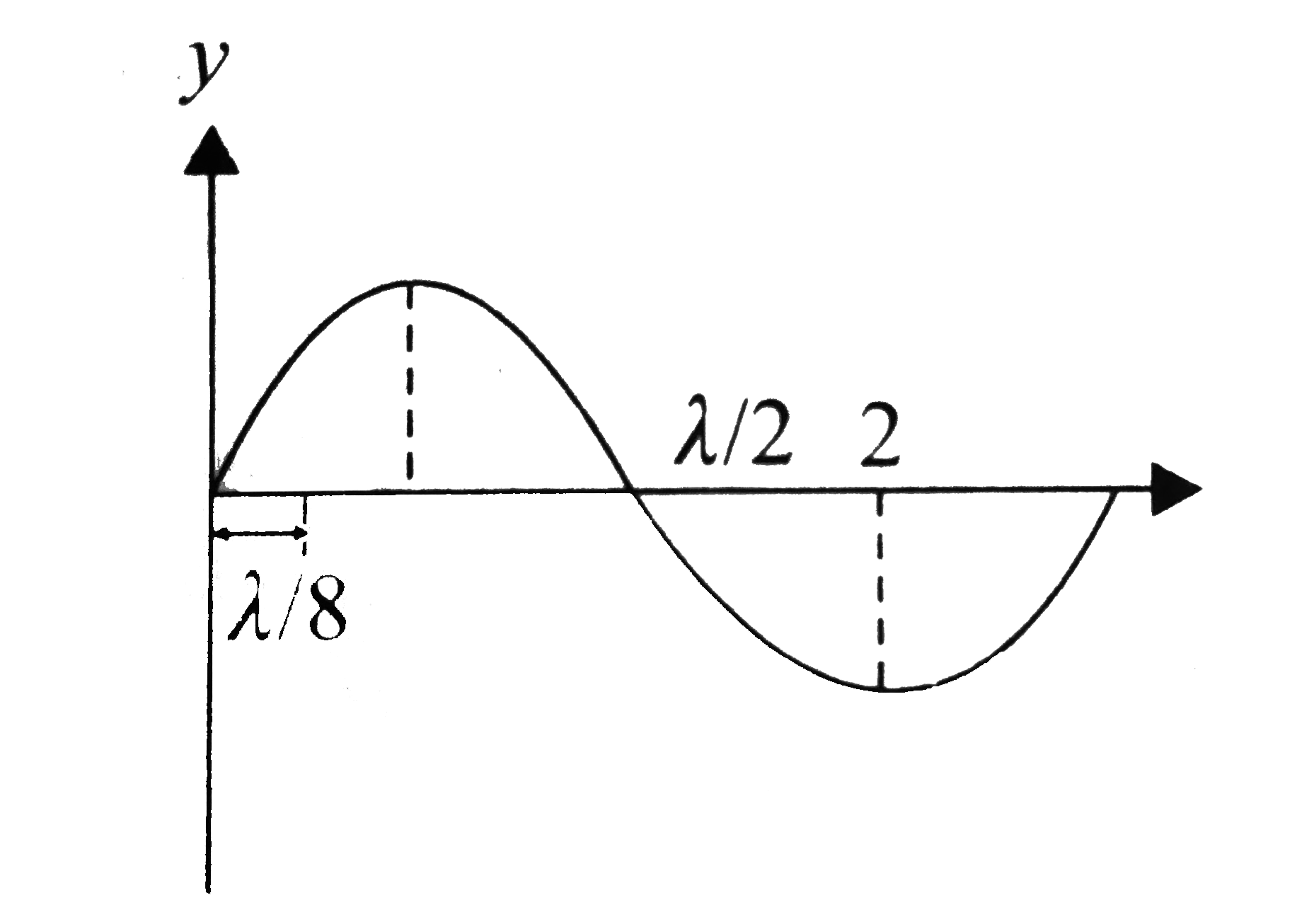
What is the phase difference between particles being on either side of a node?

Explanation

To determine the phase difference between particles located on either side of a node in a stationary wave, we can follow these steps:

1. Understanding Nodes in Stationary Waves:
- A node is a point in a stationary wave where the amplitude is zero. This means that at the node, there is no displacement of the medium.

2. Visualizing the Stationary Wave:
- Draw a diagram of a stationary wave. Identify the node, which is typically located at the midpoint between two antinodes.

3. Observing Particle Motion:
- Consider two particles: one located just to the left of the node and the other just to the right of the node.

4. Direction of Vibration:
- At any given moment, the particle on the left side of the node will vibrate in one direction (e.g., upwards), while the particle on the right side of the node will vibrate in the opposite direction (e.g., downwards).

5. Phase Relationship:
- Since the particles on either side of the node are moving in opposite directions at the same time, their phase difference can be analyzed.

6. Calculating the Phase Difference:
- The phase difference between two points in a wave is given by the formula:
\( \text{Phase Difference} = \phi = 2\pi \times \frac{\Delta x}{\lambda} \)
where \(\Delta x\) is the distance between the two points and \(\lambda\) is the wavelength. For points on either side of a node, this distance is half the wavelength (\(\frac{\lambda}{2}\)).
- Substituting \(\Delta x = \frac{\lambda}{2}\):
\( \phi = 2\pi \times \frac{\frac{\lambda}{2}}{\lambda} = \pi \)
- This means the phase difference is \(180^\circ\).

7. Conclusion:
- Therefore, the phase difference between particles on either side of a node is \(180^\circ\).