What is the temperature of steel-copper jumction in the steady state of the system shown in fig. Length of the steel rod = 30.0 cm, length of the copper rod = 20.0 cm, temperature of the furnace = 300∘C, temperature of cold end =0∘C . The area of cross-section of the steel rod is twice that of the copper rod. thermal conductivity of steel = 50.2Js−1m−1.∘C−1 and of copper = 358Js−1m−1.∘C−1.
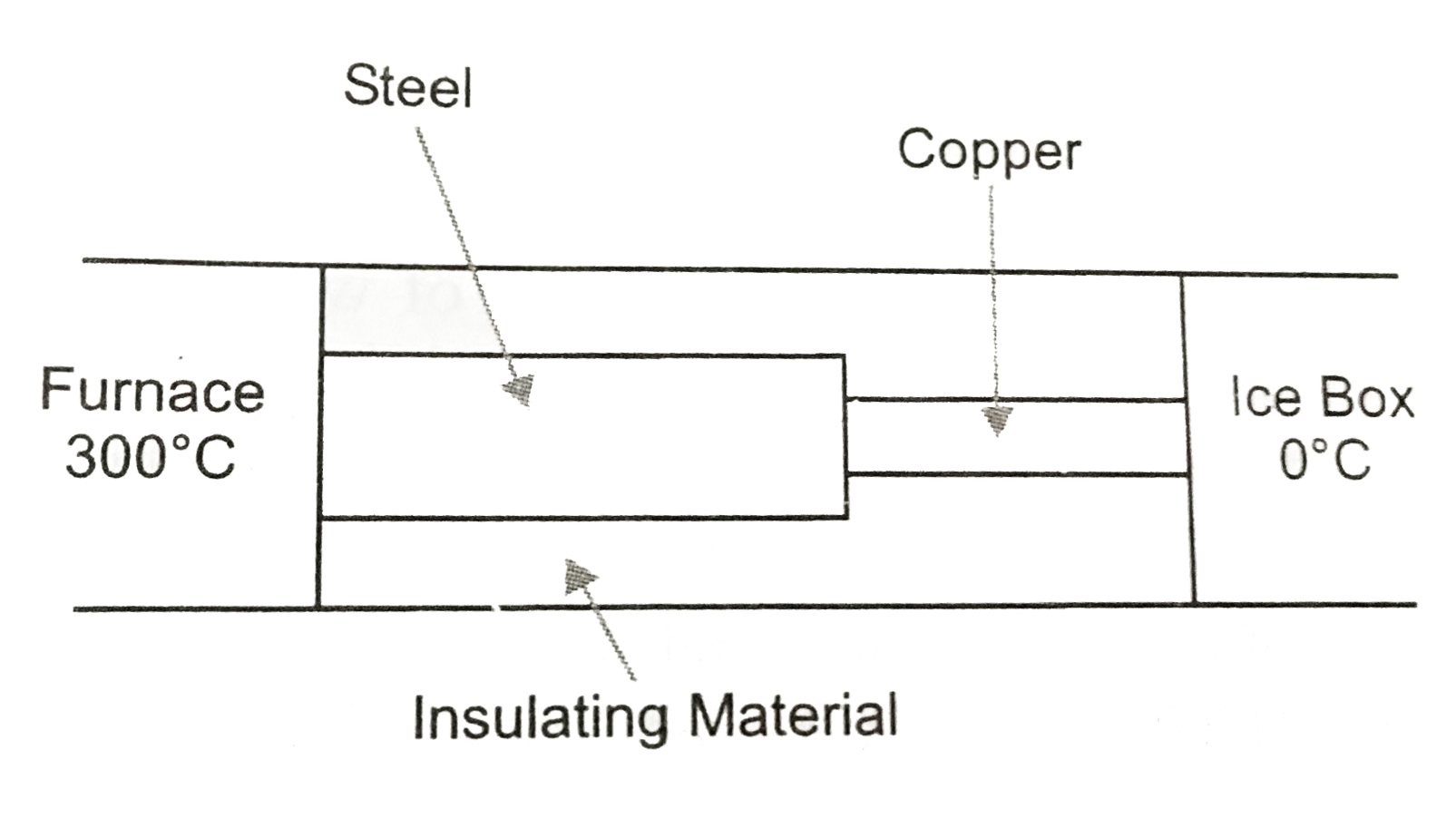
In the steady state, let T be the temperature of steel-copper junction.
Then heat flowing per second of steel = heat flowing per second of copper.
K1A1(300−T)x1=K2A2(T−0)x2or50.2×2A2×(300−T)30=385×A2(T−0)20
or, 100.4(300−T)×20=385T×30
On solving , T=44.4∘C
Topper's Solved these Questions
- PROPERTIES OF BULK MATTERBook:PRADEEPChapter:PROPERTIES OF BULK MATTERExercise:Curiosity Questions
Explore 9 Videos
- PROPERTIES OF BULK MATTERBook:PRADEEPChapter:PROPERTIES OF BULK MATTERExercise:Very short answer questions
Explore 163 Videos
- PHYSICAL WORLD AND MEASUREMENTBook:PRADEEPChapter:PHYSICAL WORLD AND MEASUREMENTExercise:Competiton Focus Jee Medical Entrance
Explore 18 Videos
- RAY OPTICSBook:PRADEEPChapter:RAY OPTICSExercise:Problem For Practice(a)
Explore 25 Videos
Similar Questions
What is the temperature of the steel-copper junction in the steady state system show in Fig. 7(e).17? Length of steel rod = 15.0 cm, length of the copper rod = 10.0 cm, temperature of the furnace =300∘C, temperature of other end 0∘C. The are of cross-section of the steel rod is twice that of the copper rod. (Thermal conductivity of steel =50.2js−1m−1K−1 and of copper =3895js−1m−1K−1).
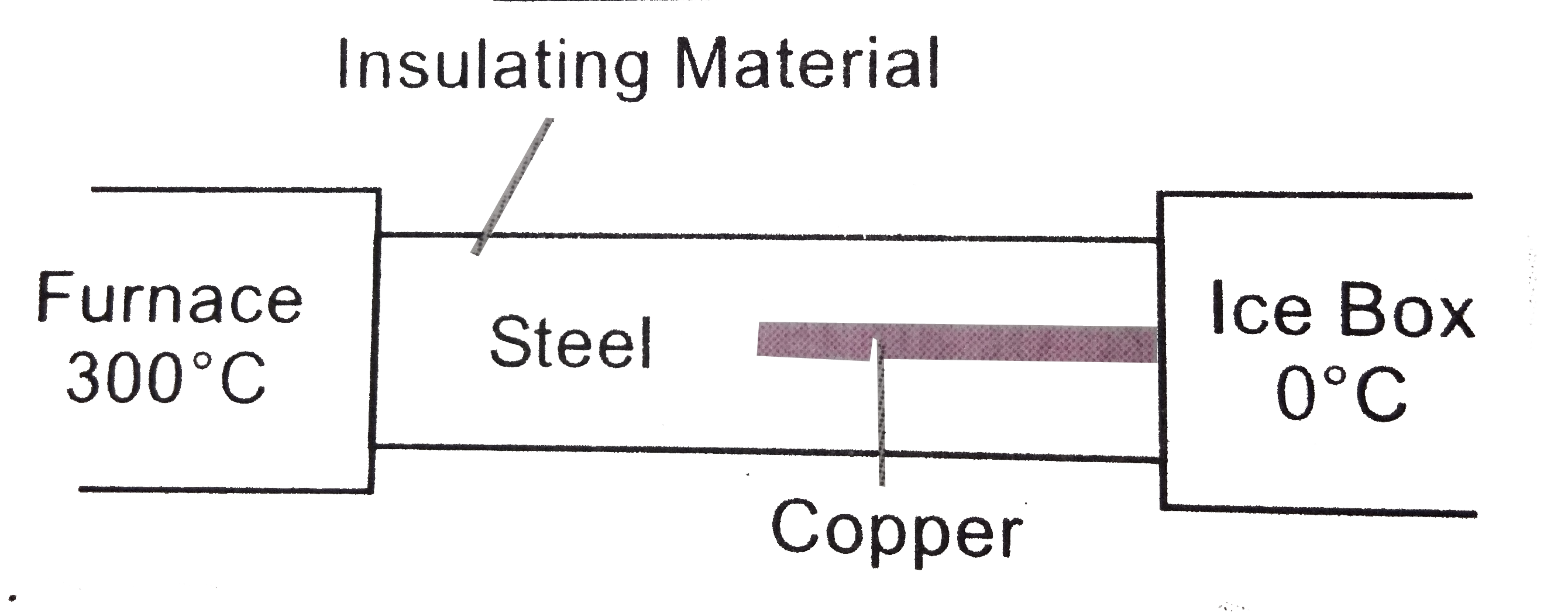
View SolutionWhat is the temperature of the steel -copper junction in the steady state of the system shown in the figure. Length of the steel rod =25cm, length of the copper rod =50cm, temperature of the furance =300∘C, temperature of the other end =0∘C. The area of cross section of the steel rod is twice that of the copper rod. (Thermal conductivity of steel =50Js−1m−1K−1 and of copper =400Js−1m−1K−1)

View Solution
Knowledge Check
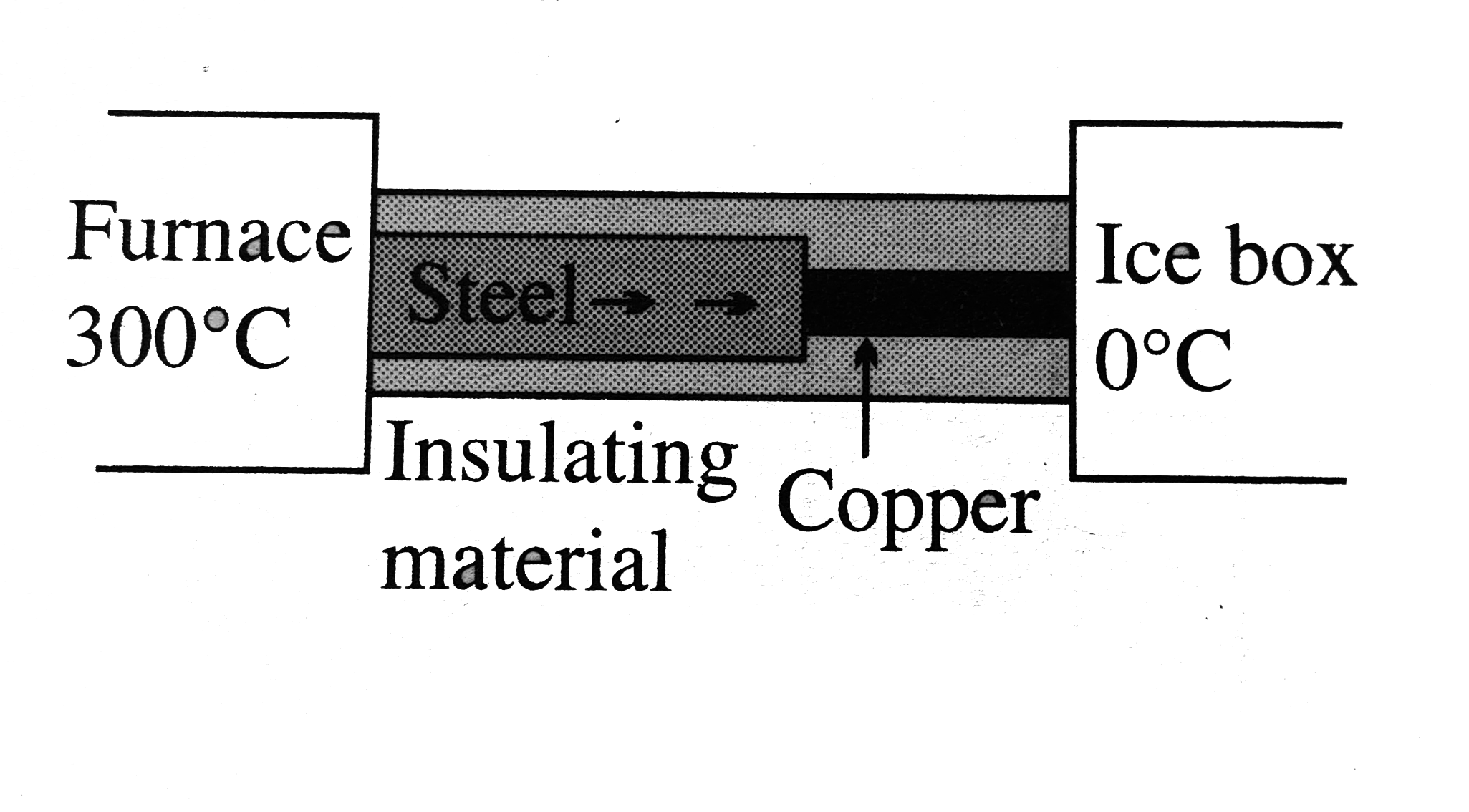
What is the temperature of the steel-copper junction in the steady state of the system shown if Fig? Length of the steel rod =25cm, length of the copper rod=50cm, temperature of the furnace=300∘C, temperature of the other end =0∘C. The area of cross section of the steel rod is twice that of the copper rod. (Thermal conductivity of steel =50J/sm and of copper =400Js/mK).A150∘CB200∘CC100∘CD250∘CA steel tape measures that length of a copper rod as 90.0 cm when both are at 10∘C, the calibration temperature, for the tape. What would the tape read for the length of the rod when both are at 30∘C. Given αsteel=1.2×10−5 per.∘CandαCu=1.7×10−5per.∘C
A90.01 cmB89.90 cmC90. 22 cmD89. 80 cmA rod of 1m length and area of cross-section 1cm2 is connected across two heat reservoirs at temperature 100∘C and 0∘C as shown. The heat flow per second through the rod in steady state will be [Thermal conductivity of material of rod =0.09kilocalm−1s−1('∘C)]
 A9×10−4kilocal/secB9kilocal/secC0.09kilocal/secD9×10−6kilocal/sec
A9×10−4kilocal/secB9kilocal/secC0.09kilocal/secD9×10−6kilocal/sec
Find the thermal resistance of an aluminium rod of length 20cm and area of cross section 1cm2 . The heat current is along the length of the rod. Thermal conductivity of aluminium =200Wm−1K−1 .
View SolutionAn aluminium rod and a copper rod of equal length 1.0m and cross-sectional area. 1cm2 are welded together as shown in figure. One end is kept at a temperature of 20∘C and the other at 60∘C Calculate the amount of heat taken out per second from the hot end. thermal conuctivity of aluminium =200Wm−1^(@)C^(-1)andofcopper=390Wm^(-1)^(∘)C−1 .

View SolutionFigure shows a copper rod joined to a steel rod. The rods have equal length and and the equal cross sectional area. The free end of the copper rod is kept at 0∘C and that of the steel rod is kept at 100∘C . Find the temperature at the junction of the rods. conductivity of copper =390WM−1^(@)C^(-1)andtˆofsteel=46Wm^(-1)(∘)C−1 .

View SolutionA bar of copper of length 75cm and a abr of steel of length 125cm are joined together end to end. Both are of circular cross section with diameter 2cm. The free ends of the copper and the steel bors are maintained at 100∘C and 0^(@)Crespectively.Thecurvedsurfaceofthebors.arethermally∈sa––ted.Wˆisthetemperatureofthecoppe−steeljunction?Wˆistheamountofheattransmiedperunittimeacrossthejunction?Thermalconductivityofcopperis386Js^(-1)m^(-1)^(∘)C−1 and that of steel is 46Js^(-1)m^(-1)(@)C^(-1)
View SolutionThe ends of copper rod of length 1m and area of cross section 1cm2 are maintained at 0∘C and 100∘C. At the centre of rod, there is a source of heat of 32W. The thermal conductivity of copper is 400W/mK

View Solution