What will happen to the emission rate if the temperature of a black body is increased from 200 K to 400 K?
To determine how the emission rate of a black body changes when its temperature is increased from 200 K to 400 K, we can use Stefan-Boltzmann Law, which states that the emission rate (E) of a black body is proportional to the fourth power of its absolute temperature (T).
1. Understanding the Stefan-Boltzmann Law:
The law states that the emission rate E is given by:
E=σT4
where σ is the Stefan-Boltzmann constant.
2. Initial and Final Temperatures:
Let the initial temperature T1=200K and the final temperature T2=400K.
3. Calculating Initial Emission Rate:
Using the Stefan-Boltzmann Law, the initial emission rate E1 at T1 is:
E1=σ(T1)4=σ(200)4
4. Calculating Final Emission Rate:
The final emission rate E2 at T2 is:
E2=σ(T2)4=σ(400)4
5. Finding the Ratio of Emission Rates:
To find how the emission rates compare, we take the ratio E2E1:
E2E1=σ(400)4σ(200)4=(400)4(200)4
6. Simplifying the Ratio:
Since σ cancels out, we simplify the ratio:
E2E1=(400200)4=24=16
7. Conclusion:
Therefore, the emission rate increases by a factor of 16 when the temperature of the black body is increased from 200 K to 400 K.
Final Answer:
The emission rate will increase by 16 times.
The law states that the emission rate E is given by:
E=σT4
where σ is the Stefan-Boltzmann constant.
Let the initial temperature T1=200K and the final temperature T2=400K.
Using the Stefan-Boltzmann Law, the initial emission rate E1 at T1 is:
E1=σ(T1)4=σ(200)4
The final emission rate E2 at T2 is:
E2=σ(T2)4=σ(400)4
To find how the emission rates compare, we take the ratio E2E1:
E2E1=σ(400)4σ(200)4=(400)4(200)4
Since σ cancels out, we simplify the ratio:
E2E1=(400200)4=24=16
Therefore, the emission rate increases by a factor of 16 when the temperature of the black body is increased from 200 K to 400 K.
The emission rate will increase by 16 times.
Topper's Solved these Questions
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - REVISION EXERCISE (ADDITIONAL QUESTION)
3 Videos
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - REVISION EXERCISE (FILL IN THE BLANKS)
10 Videos
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - HIGH ORDER THINKING SKILLS AND ADVANCED LEVEL
10 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Chapter Practice Test (for Board Examination)
16 Videos
- UNITS AND MEASUREMENTBOOK - MODERN PUBLICATIONCHAPTER - UNITS AND MEASUREMENTEXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
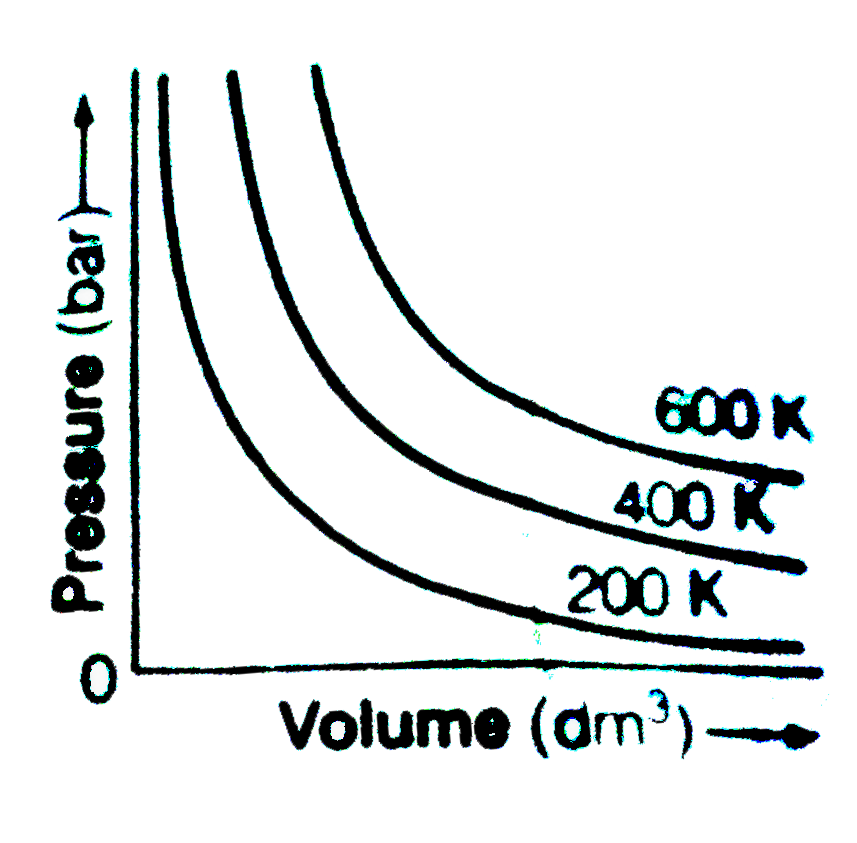
The variation of pressure with volume of the gas at different temperatures can be graphically represented as shown in figure. On the basis of this graph answer the following question.
(i) How will the volume of a gas change if its pressure is increased at constant temperature ?
(ii) At a constant pressure, how will the volume of a gas change if the temperature is increased from 200 K to 400 K ?
View SolutionWhat is the energy of activation of a reaction is its rate doubles when the temperature is raised from 290 K to 300 K ?
View Solution
Knowledge Check
The temperature of a black body is gradually increased. The colour of the body will change from
AWhite - green - redBRed - yellow - blueCRed - violet - yellowDYellow - green - redBy 10 K increase in temperature, the rate of reaction becomes double. How many times the rate of reaction will be if the temperature is increased from 303 K to 353 K ?
A32B6C8D4The activation energy of a reaction is 9.0kcal/mol.
The increase in the rate consatnt when its temperature is increased from 298K to 308K isA0.63B0.5C1D0.1
What is the activation energy (KJ/mol) for a reaction if its rate constant doubles when the temperature is raised from 300 K to 400 K ? (R=8.314 J mol−1K−1)
View SolutionThe activation energy of a reaction is 9.0kcal/mol.
The increase in the rate consatnt when its temperature is increased from 298K to 308K is
View SolutionWhat is the energy of activation of a reaction is its rate doubles when the temperature is raised from 290 K to 300 K ?
View SolutionWhat is the energy of activation of a reaction if its rate doubles when the temperature is raised from 290 K to 300 K?
View SolutionThe ratio of the wavelengths of emission corresponding to the maximum emission in the spectrum of a black body heated to temperatures 1000K and 2000K respectively is
View Solution
