When a body of mass 2.0 kg is suspended by a spring, the spring is stretched. If the body is pulled down slightly and released, it coscillated up and down. What force is applied on the body by the spring when it passes through the mean position ? (g=9.8Nkg−1)
To solve the problem, we need to determine the force applied on the body by the spring when it passes through the mean position during its oscillation.
1. Identify the Mass and Gravitational Force:
- The mass of the body (m) is given as 2.0 kg.
- The acceleration due to gravity (g) is given as 9.8 N/kg.
- The weight (W) of the body can be calculated using the formula:
W=m⋅g=2.0kg⋅9.8N/kg=19.6N
2. Understanding the Mean Position:
- The mean position in oscillation is where the spring force and the weight of the body are balanced.
- At the mean position, the net force acting on the body is zero, which means that the spring force (F_spring) equals the weight of the body (W).
3. Applying Hooke's Law:
- According to Hooke's Law, the force exerted by the spring (F_spring) is proportional to the displacement (x) from the mean position:
Fspring=k⋅x
- However, at the mean position, the spring force is equal to the weight of the body:
Fspring=W
4. Conclusion:
- Since the spring force at the mean position equals the weight of the body, we can conclude:
Fspring=19.6N
- Therefore, the force applied on the body by the spring when it passes through the mean position is 19.6 N.
Final Answer:
The force applied on the body by the spring when it passes through the mean position is 19.6 N.
- The mass of the body (m) is given as 2.0 kg.
- The acceleration due to gravity (g) is given as 9.8 N/kg.
- The weight (W) of the body can be calculated using the formula:
W=m⋅g=2.0kg⋅9.8N/kg=19.6N
- The mean position in oscillation is where the spring force and the weight of the body are balanced.
- At the mean position, the net force acting on the body is zero, which means that the spring force (F_spring) equals the weight of the body (W).
- According to Hooke's Law, the force exerted by the spring (F_spring) is proportional to the displacement (x) from the mean position:
Fspring=k⋅x
- However, at the mean position, the spring force is equal to the weight of the body:
Fspring=W
- Since the spring force at the mean position equals the weight of the body, we can conclude:
Fspring=19.6N
- Therefore, the force applied on the body by the spring when it passes through the mean position is 19.6 N.
The force applied on the body by the spring when it passes through the mean position is 19.6 N.
Topper's Solved these Questions
- OSCILLATIONS AND WAVESBOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Short Answer Questions
104 Videos
- OSCILLATIONS AND WAVESBOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Long Answer Questions
33 Videos
- OSCILLATIONS AND WAVESBOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - conceptual problems
84 Videos
- MATHEMATICAL TOOLSBOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks
5 Videos
- PHYSICAL WORLD AND MEASUREMENTBOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Competiton Focus Jee Medical Entrance
18 Videos
Similar Questions
A spring has a natural length of 50 cm and a force constant of 2.0×103 Nm−1. A body of mass 10 kg is suspended from it and the spring is stretched. If the body is pulled down to a length of 58 cm and released, it executes simple harmonic motion. The net force on the body when it is at its lowermost position of its oscillation is (10x) newton. Find value of x. (Take g=10m/s2)
View SolutionWhen a body of mass M is hung from a spring, the spring extends by 1 em. If the body of mass 2M be hung from the same spring, the extension of spring will be
View Solution
Knowledge Check
When a mass m is hung on a spring, the spring stretched by 6 cm. If the loaded spring is pulled downward a little and released, then the period of vibration of the system will be
A0.27 sB0.35 sC0.49 sD0.64 sA body of mass m is suspended from three springs as shown in figure. If mass m is displaced slightly then time period of oscillation is
 A2π√m3KB2π√3m2KC2π√2m3KD2π√3Km
A2π√m3KB2π√3m2KC2π√2m3KD2π√3KmA body of mass 5 kg is suspended by a spring balance on an inclined plane as shown in figure. The spring balance measure
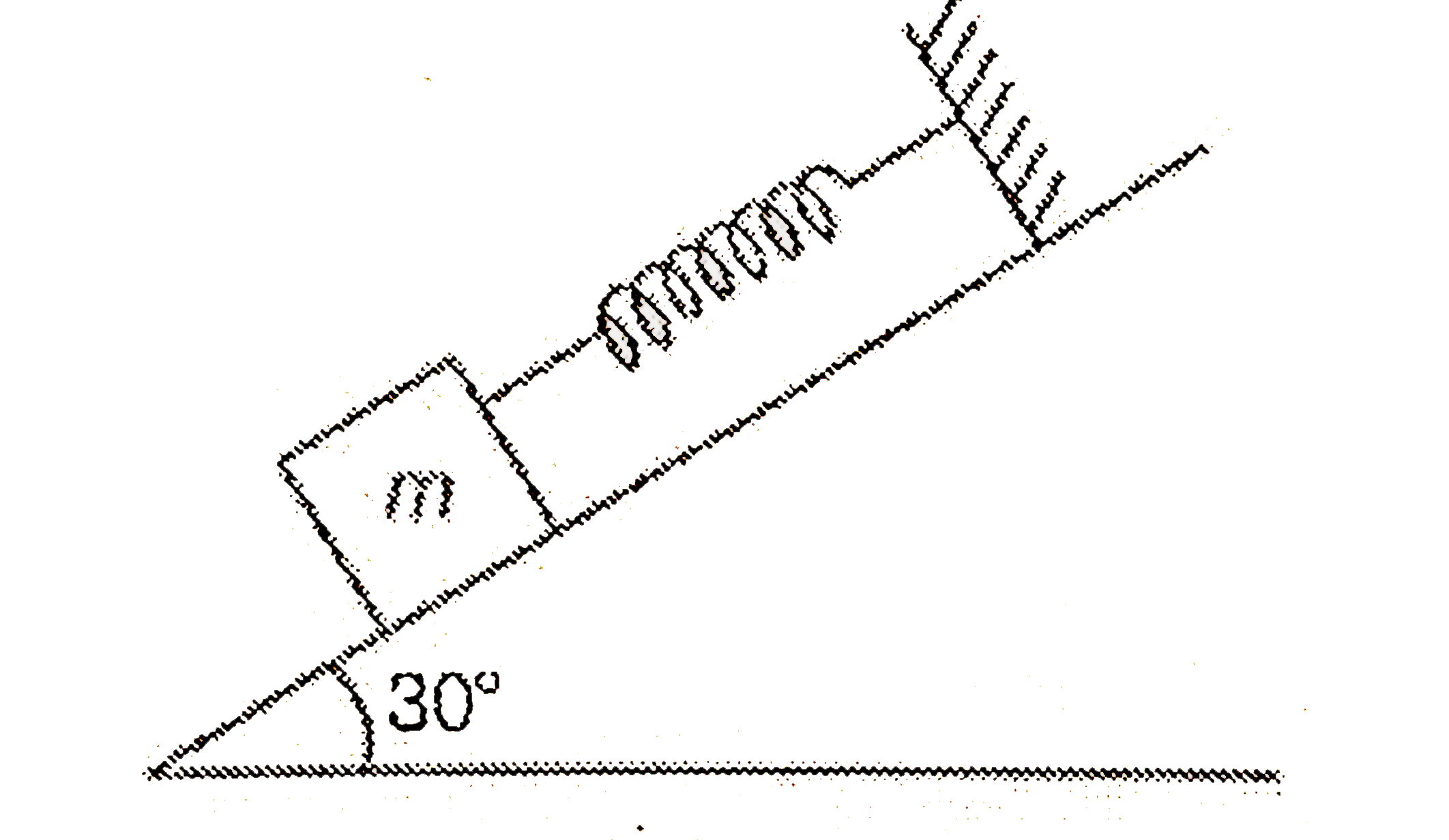 A50 NB25 NC500 ND10 N
A50 NB25 NC500 ND10 N
A body of mass 5 kg is suspended by a spring balance on an inclined plane as shown in figure. The spring balance measure
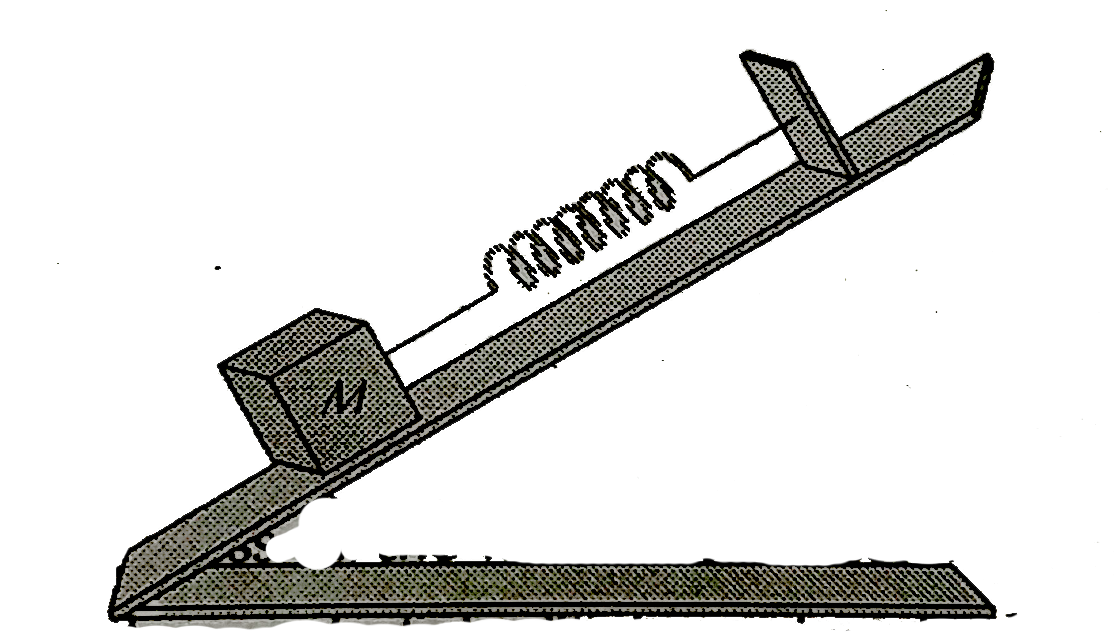
View SolutionA mass of 1 kg is hanged through a spring. The spring is stretched through 1cm. What is the energy stared in spring?
View SolutionA mass of 1kg is hanged through a spring. The spring is stretched through 1cm. What is the energy stared in spring?
View SolutionA body of mass 50 kg is suspended using a spring balance inside a lift at rest. If the lift startts falling freely, the reading of the spring balance is
View SolutionA body of mass 1 kg suspended by a light vertical spring of spring constant 100 N/m executes SHM. Its angular frequency and frequency are
View Solution
