When two resistances connected in series and parallel their equivalent resistances are 15Ω and 5615Ω respectively. Find the individual resistances. Solution in Tamil
Rs=R1+R2=15Ω …(1)
Rp=R1R2R1+R2=5615Ω …(2)
From equation (1) substituting for R1+R2 in equation (2)
R1R215=5615Ω ∴R1R2=56
R2=5615Ω ...(3)
Substituting for R2 in equation (1) from equation (3)
R1+5615=15 then, R21+56R1=15
R21+56=15R1⇒R21−15R1+56=0
The above equation can be solved using factorisation.
R21−8R1−7R1+56=0
R1(R1−8)−7(R1−8)=0⇒(R1−8)(R1−7)=0
If (R1=8Ω), using in equation (1)
8+R2=15⇒R2=15−8=7Ω
R2=7Ω i.e, (when R1=8Ω,R2=7Ω)
If (R1=7Ω), substituting in equation (1)
7+R2=15⇒R2=8Ω, i.e, (when R1=8Ω,R2=7Ω)
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - FULL MARKSCHAPTER - CURRENT ELECTRICITYEXERCISE - TEXTBOOK EVALUATION (Choose the correct answer)
15 Videos
- CURRENT ELECTRICITYBOOK - FULL MARKSCHAPTER - CURRENT ELECTRICITYEXERCISE - TEXTBOOK EVALUATION (Short Answer Questions)
21 Videos
- COMMUNICATION SYSTEMSBOOK - FULL MARKSCHAPTER - COMMUNICATION SYSTEMSEXERCISE - ADDITIONAL QUESTIONS (Additional problems)
3 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - FULL MARKSCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - ADDITIONAL QUESTIONS -( ADDITIONAL NUMERICAL PROBLEMS : )
10 Videos
Similar Questions
The following graph shows current versus voltage values of some unknown conductor. What is the resistance of this conductor?
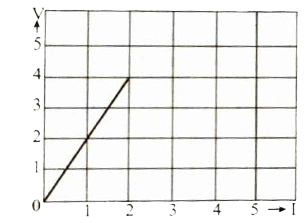
View SolutionWhy current is a scalar?
View SolutionA radio can tune into any station in the 7.5 MHz to 12 MHz band. What is the corresponding wavelength band ?
View SolutionIf a light of wavelength 4950 Å is viewed as a continuous flow of photons, what is the energy of each photon in eV ? (Given h=6.6×10−34Js, c =3×108ms−1)
View SolutionThe resistance of two conductors in series is 40Ω and their resistance becomes 6.4Ω, when connected in parallel. Find the resistance of individual conductors.
View SolutionThe Total resistance when connected in series in 9Ω and when connected in parallel is 2Ω The value of two resistance are
View SolutionThe resistance of two conductors in series is 40Ω and their resistance becomes 7.5Ω, when coneected in parallel. Find the resistance of the individual conductors.
View SolutionThe resistance of two conductors in series is 18 Omega and the resistance becomes 4 Omega when connected in parallel. Find the resistance of individual conductors.
View SolutionThe equivalent resistance of two coils connected in series and in parallel are 12Ω and 53Ω respectively. Calculate the value of each resistance.
View SolutionTwo resistance 60.36 Omega and 30.09 Omega are connected in parallel. The equivalent resistance is
View SolutionThe equivalent resistance of two resistors connected in series is 6 Omega and their parallel equivalent resistance is 4/3 Omega . What are the values of resistances ?
View SolutionWhen resistors 1 and 2 are connected in series, the equivalent resistance is 20.0 Omega. When they are connected in parallel, the equivalent resistance is 3.75 Omega. What are (a) the smaller resistance and (b) the larger resistance of these two resistors?
View SolutionThe resultant resistance of two resistance in series is 50 Ω and it is 12 Ω , when they are in parallel. The individual resistances are
View SolutionThe resultant resistance of two resistance in series is 50Ωand it is 12Ω, when they are in parallel. The individual resistances are
View SolutionFind the equivalent of resistances of the individual resistance connected in series?
View Solution
