With the usual notations , check if the following equation St=u+12a(2t−1) is dimensionally correct or not.
To check if the equation St=u+12a(2t−1) is dimensionally correct, we will analyze the dimensions of each term in the equation.
Step 1: Identify the dimensions of each variable
1. Displacement St: The dimension of displacement is denoted as (St)=L (length).
2. Initial velocity u: The dimension of velocity is given by (u)=LT−1 (length per time).
3. Acceleration a: The dimension of acceleration is given by (a)=LT−2 (length per time squared).
4. Time t: The dimension of time is denoted as (t)=T.
Step 2: Analyze the term 12a(2t−1)
1. The term (2t−1) can be simplified. Since 2 is a dimensionless constant, the dimension of (2t) is the same as that of t, which is (t)=T. The constant 1 is also dimensionless, so we can ignore it for dimensional analysis.
2. Therefore, the dimension of (2t−1) is (2t)=T.
Step 3: Calculate the dimensions of the term a(2t−1)
1. Now we multiply the dimensions of a and (2t−1):
(a(2t−1))=(a)⋅(2t−1)=(LT−2)⋅(T)=LT−1
Step 4: Combine the terms in the equation
1. Now we can rewrite the equation:
St=u+12a(2t−1)
The dimensions of each term are:
- (St)=L
- (u)=LT−1
- (12a(2t−1))=LT−1
Step 5: Check for dimensional consistency
1. The left-hand side St has the dimension L, while both terms on the right-hand side u and 12a(2t−1) have the dimension LT−1.
2. Since the dimensions on both sides of the equation do not match, the equation is not dimensionally correct.
Conclusion
The equation St=u+12a(2t−1) is not dimensionally correct.
---
2. Initial velocity u: The dimension of velocity is given by (u)=LT−1 (length per time).
3. Acceleration a: The dimension of acceleration is given by (a)=LT−2 (length per time squared).
4. Time t: The dimension of time is denoted as (t)=T.
2. Therefore, the dimension of (2t−1) is (2t)=T.
(a(2t−1))=(a)⋅(2t−1)=(LT−2)⋅(T)=LT−1
St=u+12a(2t−1)
The dimensions of each term are:
- (St)=L
- (u)=LT−1
- (12a(2t−1))=LT−1
2. Since the dimensions on both sides of the equation do not match, the equation is not dimensionally correct.
Topper's Solved these Questions
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Exercise 1.2
6 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Exercise 1.3
17 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
Knowledge Check
Assertion: The given equation x=x0+u0t+12at2 is dimensionsally correct, where x is the distance travelled by a particle in time t , initial position x0 initial velocity u0 and uniform acceleration a is along the direction of motion.
Reason: Dimensional analysis can be used for cheking the dimensional consistency or homogenetly of the equation.Aif both assertion and reason are true reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation fo assertion.CIf assertion is true but reaso is false.DIF both assertion and reason are false.
Check whether the following equation is a solution to one dimensional wave equation. y−4x−7t
View Solution[ML2T−3A−1] is the dimensional formula for
View Solution[ML−1T−2] is the dimensional formula of
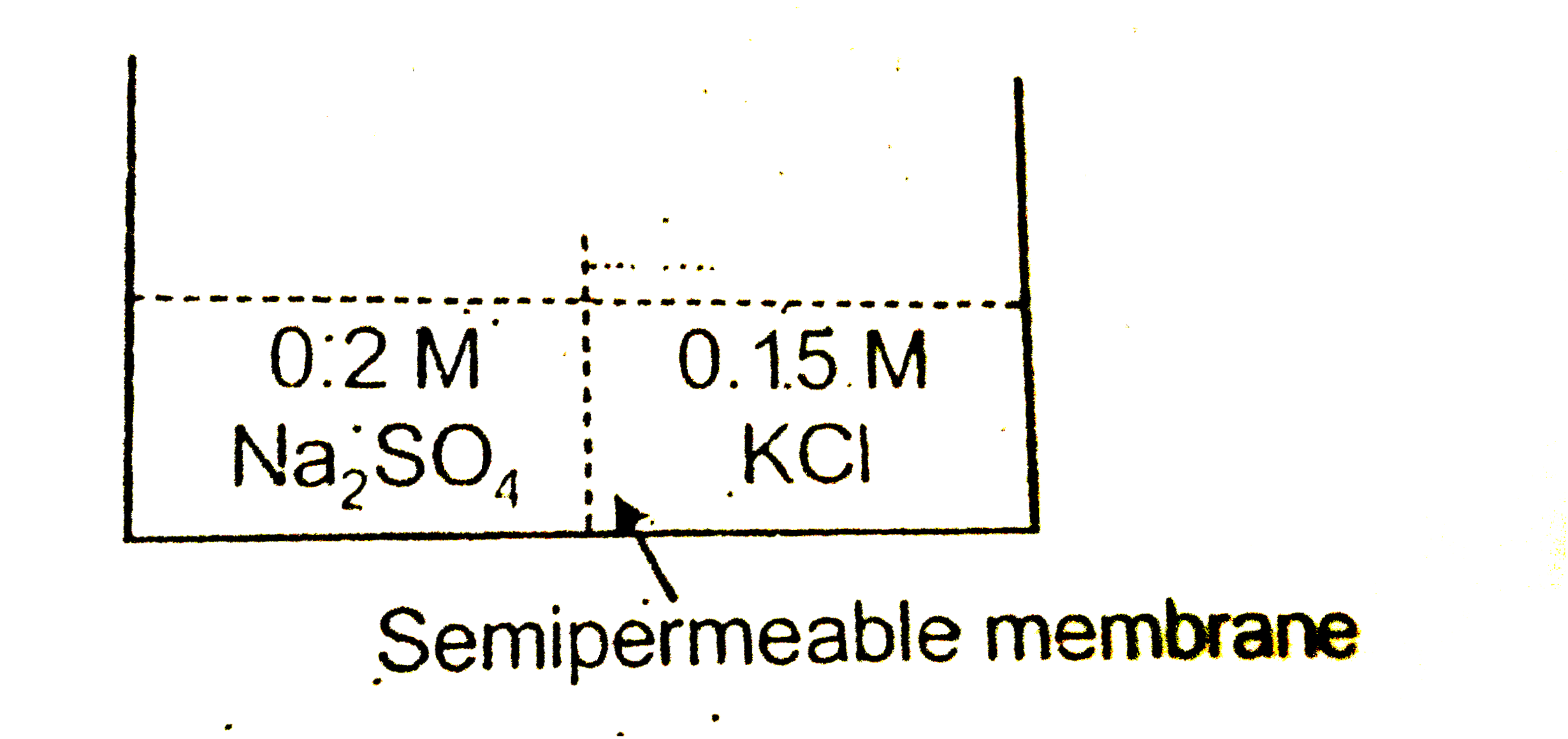
View SolutionConsider the following arrangement and choose the correct options
View SolutionProve the relation, st=u+at−12a.
View SolutionWhich of the following quantity has dimensional formula as (ML−1T−2)?
View SolutionIn the s−t equations (s=10+20t−5t2) match the following
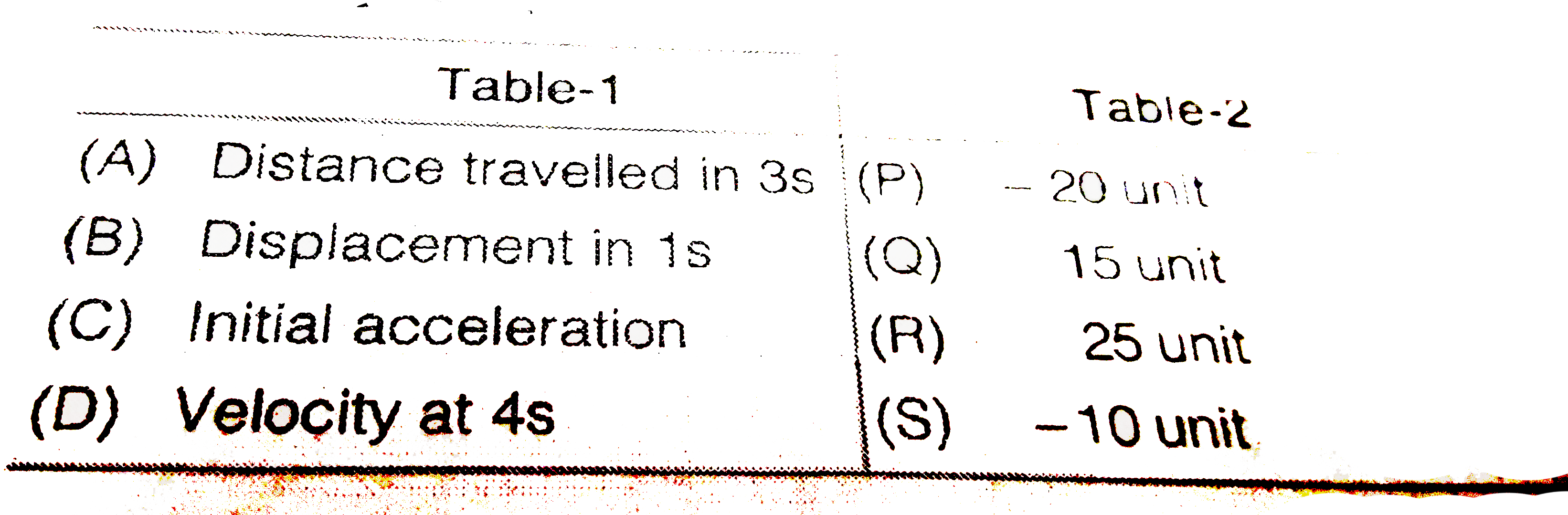
View Solution
