Work function of potassium metal is 2.30 eV . When light of frequency 8×1014 Hz is incident on the metal surface, photoemission of electrons occurs. The stopping potential of the electrons will be equal to
0.1 V
1.0 V
2.3 V
3.3 V
The correct Answer is:B
To solve the problem, we need to calculate the stopping potential of the electrons emitted from potassium metal when light of a certain frequency is incident on it. The stopping potential can be determined using the photoelectric effect equation.
1. Identify the Given Values:
- Work function of potassium, ϕ=2.30eV
- Frequency of incident light, ν=8×1014Hz
2. Calculate the Energy of the Incident Photons:
The energy of the photons can be calculated using the formula:
E=hν
where h (Planck's constant) is approximately 6.63×10−34Js.
Substituting the values:
E=(6.63×10−34Js)×(8×1014Hz)
3. Convert Energy from Joules to Electron Volts:
To convert energy from Joules to electron volts, we use the conversion factor 1eV=1.6×10−19J:
E(in eV)=E(in J)1.6×10−19J/eV
4. Calculate the Maximum Kinetic Energy of the Emitted Electrons:
The maximum kinetic energy (Kmax) of the emitted electrons can be calculated using the photoelectric equation:
Kmax=E−ϕ
where ϕ is the work function.
5. Determine the Stopping Potential:
The stopping potential V0 is related to the maximum kinetic energy by:
Kmax=eV0
Therefore, we can express the stopping potential as:
V0=Kmaxe
6. Substitute the Values and Solve:
After calculating E and Kmax, we can find V0.
- From the calculations, we find:
- E≈3.3eV
- Kmax=3.3eV−2.3eV=1.0eV
- Thus, V0=1.0V
Final Answer:
The stopping potential of the electrons will be equal to 1 V.
---
- Work function of potassium, ϕ=2.30eV
- Frequency of incident light, ν=8×1014Hz
The energy of the photons can be calculated using the formula:
E=hν
where h (Planck's constant) is approximately 6.63×10−34Js.
E=(6.63×10−34Js)×(8×1014Hz)
To convert energy from Joules to electron volts, we use the conversion factor 1eV=1.6×10−19J:
E(in eV)=E(in J)1.6×10−19J/eV
The maximum kinetic energy (Kmax) of the emitted electrons can be calculated using the photoelectric equation:
Kmax=E−ϕ
where ϕ is the work function.
The stopping potential V0 is related to the maximum kinetic energy by:
Kmax=eV0
Therefore, we can express the stopping potential as:
V0=Kmaxe
After calculating E and Kmax, we can find V0.
- E≈3.3eV
- Kmax=3.3eV−2.3eV=1.0eV
- Thus, V0=1.0V
The stopping potential of the electrons will be equal to 1 V.
Topper's Solved these Questions
- DUAL NATURE OF MATTER AND RADIATION BOOK - MTG GUIDECHAPTER - DUAL NATURE OF MATTER AND RADIATION EXERCISE - NEET Cafe Topicwise Practice Questions (PARTICLE NATURE OF LIGHT (THE PHOTON)
17 Videos
- DUAL NATURE OF MATTER AND RADIATION BOOK - MTG GUIDECHAPTER - DUAL NATURE OF MATTER AND RADIATION EXERCISE - NEET Cafe Topicwise Practice Questions (MATTER WAVES AND DE BROGLIE RELATION)
39 Videos
- DUAL NATURE OF MATTER AND RADIATION BOOK - MTG GUIDECHAPTER - DUAL NATURE OF MATTER AND RADIATION EXERCISE - AIPMT/ NEET MCQs
30 Videos
- CURRENT ELECTRICITY BOOK - MTG GUIDECHAPTER - CURRENT ELECTRICITY EXERCISE - MCQs AIPMT / NEET
32 Videos
- ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTSBOOK - MTG GUIDECHAPTER - ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTSEXERCISE - AIPMT/NEET MCQS
31 Videos
Similar Questions
Work function of potassium metal is 2.30 eV . When light of frequency 8×1014 Hz is incident on the metal surface, photoemission of electrons occurs. The stopping potential of the electrons will be equal to
View SolutionThe work function of caesium metal is 2.14 eV. When light of frequency 6×1014Hz is incidennt on the metal surface, photoemission of electrons occus. What is the
(a) maximum kinetic energy of the emitted electrons,
(b) stopping potential, and
(c) maximum speed of the emitted photoelectrons ?
View Solution
Knowledge Check
The work function of a metal is 2.5 eV. Light of wavelength 3600 Å is incident on this metal surface. The velocity of emitted photoelectrons will be
A3.8×106m/sB5.2×104m/sC4×104m/sD6×105m/sThe work function of metal is 1eV. Light of wavelength 3000Å is incident on this metal surface . The velocity of emitted photo - electrons will be
A10m/secB1×103m/secC1×104m/secD1×106m/secLight of frequency 1015 Hz falls on a metal surface of work function 2.5 eV. The stopping potential of photoelectrons in volts is
A1.6B2.5C4.1D6.6
The work function of cesium metal is 2.14 eV .When light of frequency 6×1014 Hz is incident on the metal surface.phtoemission of electrons occurs. What is the :
(a) maximum kinetic energy of the emitted electrons,
(b) stopping potential , and
(c) maximum speed of the emitted photo - electrons ?
View SolutionThe work function of caesium is 2.14 eV. When light of frequency 6×1014Hz is incident on the metal surface, photoemission of electrons occurs. What is the (a) maximum kinetic energy of the emitted electrons. (b) stopping potential and (c) maximum speed of the emitted photoelectrons. given , h=6.63×10−34Js,1eV=1.6×10−19J,c=3×108m/s.
View SolutionThe given graph shows the variation of KE with frequency of incident radiations for two surfaces A and B.

(a) Which of the metals has greater work function ? For which of the metals will stopping potential be more for the same frequency of incident radiation ?
(b) The work function caesium metal is 2.14 eV. When light of frequency 6×1014 Hz. incident on the metal surface , what is the maximum KE of the photoelectrons and stopping potential ?
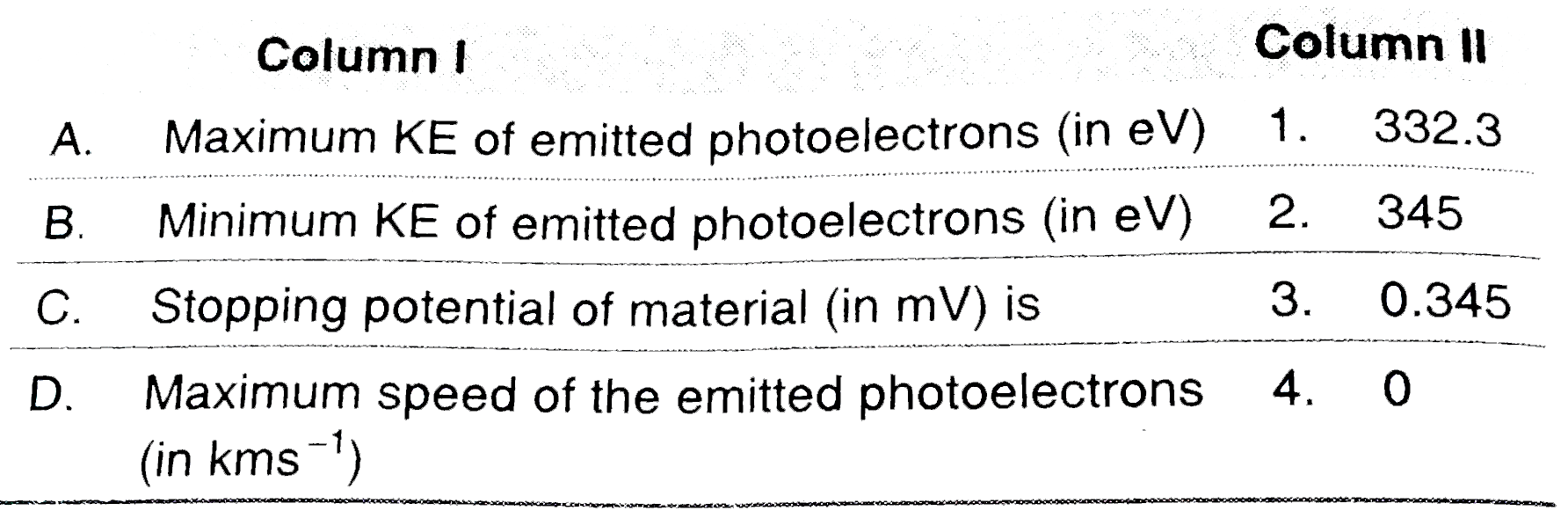
View SolutionWork function for caesium metal is 2.14 eV. Let a beam of light of frequency 6×1014Hz incident over the metal surface.
Now, match the following columns and choose the correct option from code given.
View SolutionA photon of frequency 5×1014Hz is incident on the surface of a metal. What is the energy of the incident photon in eV?
View Solution
