$x - t$ equation of a particle executing SHM is
$x = A cos (ω t - 45^{\circ})$
Find the point from where particle starts its journey and the direction of its initial velocity.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Given, $x = A cos (ω t - 45^{\circ})$
$v = \frac{d x}{d t} = - ω A sin (ω t - 45^{\circ})$
Putting $t = 0$ in both equation we have,
$x = + \frac{A}{\sqrt{2}}$ and $v = + \frac{ω A}{\sqrt{2}}$
So, the particle starts from $x = + \frac{A}{\sqrt{2}}$ with velocity in positive direction.

|

Updated on:21/07/2023

Class 11 PHYSICS OSCILLATIONS

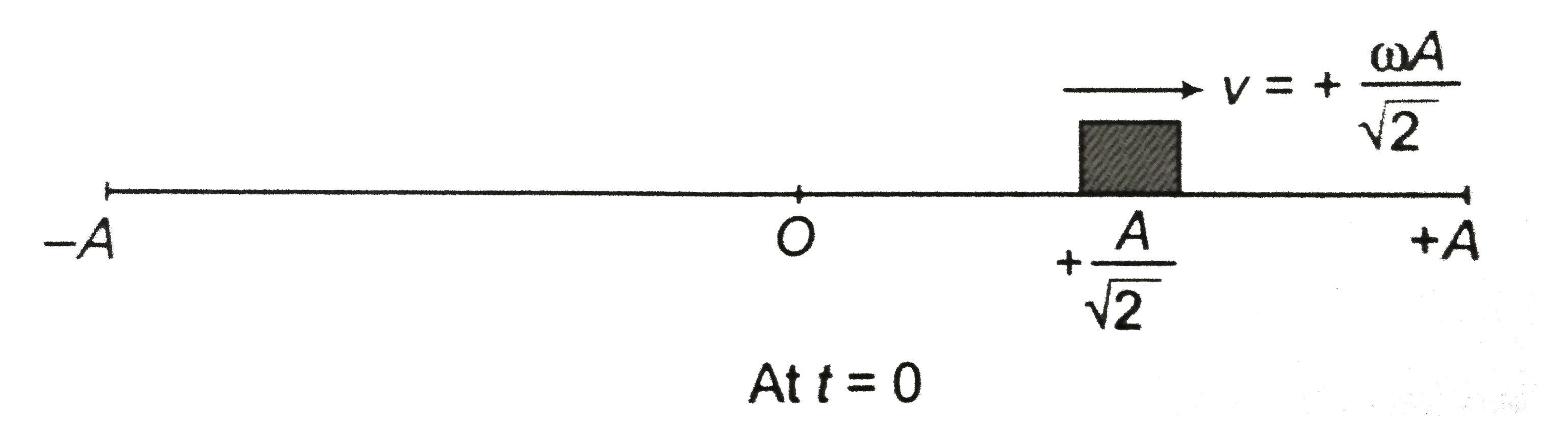
Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The displecemen-time equation of a particle execitting $S H M$ is $x = A sin (ω t + ϕ)$ At time $t = 0$ position of are position is $x = A / 2$ and it is moving along negative $x - d i r e c t i o n$ .Then the angle $ϕ$ can be
A ${[\frac{1}{k_{1}} + \frac{1}{k_{2}}]}^{1}$
B $(4 t - \frac{π}{6})$
C $\frac{1}{2 π} {(\frac{k_{1} + k_{2}}{M})}^{1 / 2}$
D $\frac{1}{2 π} {(\frac{k_{1} - k_{2}}{M})}^{1 / 2}$
Question 2 - Select One
Displacement-time equation of a particle executing SHM is $x = A sin (ω t + \frac{π}{6})$ Time taken by the particle to go directly from $x = - \frac{A}{2} to x = + \frac{A}{2}$
A $\frac{π}{3 ω}$
B $\frac{π}{2 ω}$
C $\frac{π}{ω}$
D $\frac{π}{ω}$
Question 3 - Select One
Displacement of a particle executing SHM s $x = 10 (cos π t + sin π t)$ . Its maximum speed is
A $5 π m / s$
B $10 π m / s$
C $10 \sqrt{2} π m / s$
D $5 \sqrt{2} π m / s$

DC PANDEY-SIMPLE HARMONIC MOTION-Example Type 1

x - t equation of a particle executing SHM is x = Acos (omega t - 45...
03:21

Home
Ask Doubt
Profile