144 cartons of Coke Cans and 90 cartons of Pepsi Cans are to be stacked in a Canteen. If each stack is of the same height and is to contain cartons of the same drink, what would be the greatest number of cartons each stack would have?
To solve the problem of finding the greatest number of cartons each stack would have, we need to determine the highest common factor (HCF) of the two numbers: 144 (cartons of Coke) and 90 (cartons of Pepsi).
1. Find the Prime Factorization of 144:
- Divide 144 by 2:
- 144 ÷ 2 = 72
- 72 ÷ 2 = 36
- 36 ÷ 2 = 18
- 18 ÷ 2 = 9
- 9 ÷ 3 = 3
- 3 ÷ 3 = 1
- Therefore, the prime factorization of 144 is:
144=24×32
2. Find the Prime Factorization of 90:
- Divide 90 by 2:
- 90 ÷ 2 = 45
- 45 ÷ 3 = 15
- 15 ÷ 3 = 5
- 5 ÷ 5 = 1
- Therefore, the prime factorization of 90 is:
90=21×32×51
3. Identify the Common Factors:
- From the prime factorizations:
- For 144: 24×32
- For 90: 21×32×51
- The common prime factors are:
- For 2: the minimum power is 21
- For 3: the minimum power is 32
4. Calculate the HCF:
- Multiply the common factors:
HCF=21×32=2×9=18
5. Conclusion:
- The greatest number of cartons each stack would have is 18.
- Divide 144 by 2:
- 144 ÷ 2 = 72
- 72 ÷ 2 = 36
- 36 ÷ 2 = 18
- 18 ÷ 2 = 9
- 9 ÷ 3 = 3
- 3 ÷ 3 = 1
- Therefore, the prime factorization of 144 is:
144=24×32
- Divide 90 by 2:
- 90 ÷ 2 = 45
- 45 ÷ 3 = 15
- 15 ÷ 3 = 5
- 5 ÷ 5 = 1
- Therefore, the prime factorization of 90 is:
90=21×32×51
- From the prime factorizations:
- For 144: 24×32
- For 90: 21×32×51
- The common prime factors are:
- For 2: the minimum power is 21
- For 3: the minimum power is 32
- Multiply the common factors:
HCF=21×32=2×9=18
- The greatest number of cartons each stack would have is 18.
Topper's Solved these Questions
Similar Questions
Two sets of English and social science books containing 336 and 96 respectively in a library have to be stacked in such a way that an the books are stored topic wise and the height of each stack is the same. Assuming that the books are of the same thickness, determine the number of stacks. What are the characteristics of library?
View SolutionA sweetseller has 420 kaju barfis and 130 badam barfis. She wants to stack them in such a way that each stack has the same number, and they take up the least area of the tray. What is the maximum number of barfis that can be placed in each stack for this purpose.
View Solution
Knowledge Check
84 Maths books, 90 Physics books and 120 Chemistry books have to be stacked topic wise. How many books will be their in each stack so that each stack will have the same height too?
A12B18C6D21The specific heats of several metals are given in the table. If the same number of Joules were applied to the same mass of each metal, which metal would show the greatest temperature change?
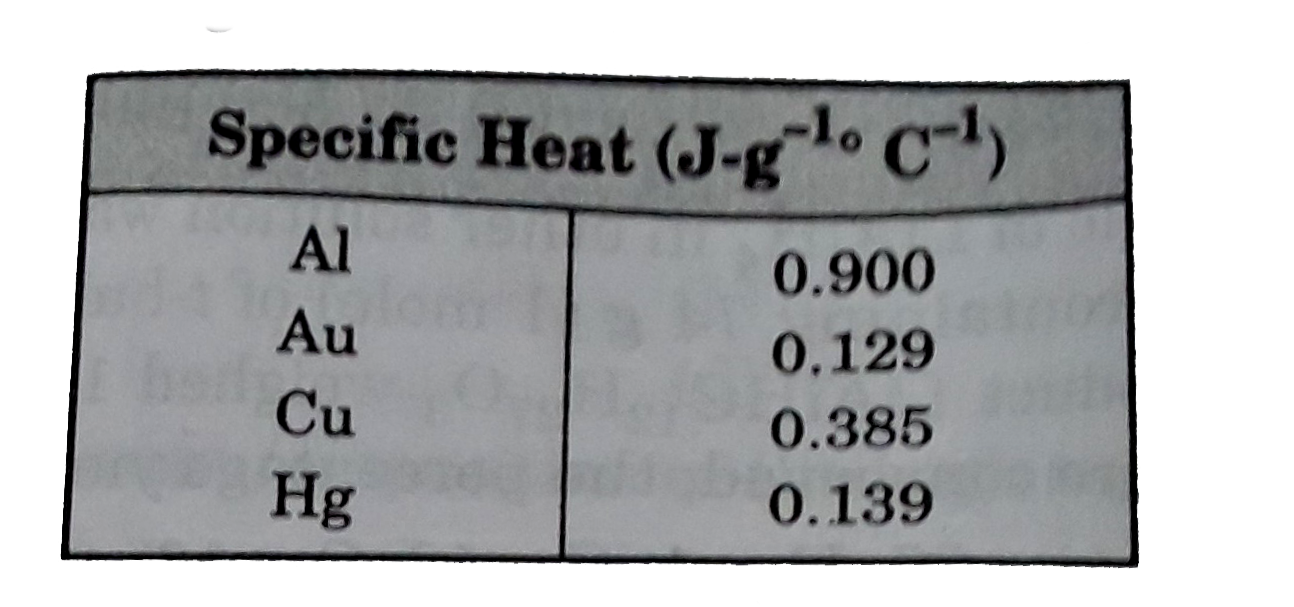 AAlBAvCCuDHg
AAlBAvCCuDHgA milk vendor has 21 lt of cow milk, 42 lt of toned milk and 63 lt of double toned milk. If he wants so pack them in cans so that each can contains same lt of milk and does not want to mix any two kinds of milk in a can, then the least number of cans required is
A3B6C9D12
A sweet seller has 420 kaju barfis and 130 badam barfis. She wants to stack them in such a way that each stack has the same number and they take up the least area of the tray. What is the maximum number of barfis that can be placed in each stack for this purpose?
View SolutionA bookseller has 420 science stream books and 130 art stream books. He wants to stack them in such a way that each stack has the take same number and they take up the least area of the surface (a) What is the maximum number of science stream books that can be placed in e stack for this purpose? (b) which mathematical concept used to solve the problem? (c) If the bookseller makes a stack, then which kinds of quality are shown by the bookseller?
View Solution1152 bars of soap can be packed in 8 cartons of the same size. How many such cartons will be required to pack 3888 bars?
View Solution84 Maths books, 90 Physics books and 120 Chemistry books have to be stacked topic wise. How many books will be their in each stack so that each stack will have the same height too?
View SolutionA,B and C start runing a race from the same point P in the same direction A runs around a path which is an equilant triangle PQX and B runs around a square path PQYZ and C runs on the regular hexagonal path PQRSTU where the one side PQ of each point is common.if all of them complete one equal round at the same time then which of the following is true?
View Solution
