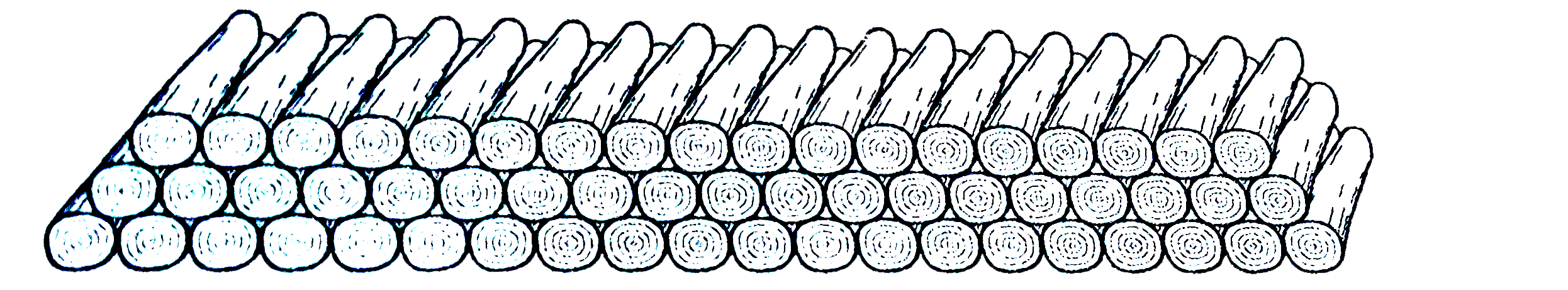
200 logs are stacked in the following manner: 20 logs in the bottom row, 19 in the next row, 18 in the row next to it and so on (see Figure). In how may rows are the 200 logs placed and how many logs are in the top row?
Here, a is the first term, d is a common difference and n is the number of terms.
It can be observed that the number of logs in rows are forming an A.P. 20,19,18,...
For this A.P.,
First terms, a=20
Common difference, d=19−20=−1
Sum of the n terms, Sn=200
We know that sum of n terms of AP is given by the formula Sn=n2[2a+(n−1)d]
200=n2[2×20+(n−1)(−1)]
400=n[40−n+1]
400=n[41−n]
400=41n−n2
n2−41n+400=0
n2−16n−25n+400=0
n(n−16)−25(n−16)=0
(n−16)(n−25)=0
Either (n−16)=0or(n−25)=0
∴ n=16 or n=25
The number of logs in nth row will be,
an=a+(n−1)d
a16=20+(16−1)×(−1)
a16=20−15
a16=5
Similarly,
a25=20+(25−1)×(−1)
a25=20−24
a25=−4
Clearly, the number of logs in the 16th row is 5. However, the number of logs in the 25th row is negative 4, which is not possible.
Therefore, 200 logs can be placed in 16 rows. The number of logs in the top (16th) row is 5.
Similar Questions
Three hundred sixty bricks are stacked in the following manner: 30 bricks in the bottom row, 29 in the next row, 28 in the row next to it and so on. In how many rows are the 360 bricks placed and how many bricks are there in the top row?
View Solution200 logs are stacked in such a way that there are 20 logs in the bottom row, 19 in the next row, 18 in the next row and so on. In how many rows, 200 logs are placed and how many logs are there in the top row?
View Solution
Knowledge Check
- Question 1 - Select One
In a row of trees one tree is the 7th from either end of the row. How many trees are there in the row ?
A11B13C15D14 - Question 2 - Select One or More
Apple tree is 16th from either end in a row of trees. How many trees are there in the row?
A31B32C30D33
In a rose garden, there are 32 rose plants in the first row, 29 in the second row, 26 in the third row and so on. There are 5 rose plants in the last row. How many rows of rose plants are there in the rose garden ?
View SolutionIn a flower bed, there are 43 rose plants in the first row, 41 in the second, 39 in the third, and so on. There are 11 rose plants in the last row. How many rows are there in the flower bed?
View SolutionIn a flower bed, there are 51 plants in the first row, 48 plants in the second row, 45 plants in the third row and so on. There are 12 plants in the last row. How many rows are there in the flower bed?
View SolutionIn a flower bed, there are 23 rose plants in the first row, 21 in the second, 19 in the third, and so on. There are 5 rose plants in the last row. How many rows are there in the flower bed?
View SolutionIn a flower bed there are 23 rose plants in the first row, twenty one in the second row, nineteen in the third row and so on. There are five plants in the last row. How many rows are there in the flower bed?
View SolutionIn how many ways can 10 soldiers stand in two rows having 5 soldiers in each row?
View Solution

