A and B are two events, such that P(A)=35andP(B)=23 if A and B are independent then find P(A intersection B)
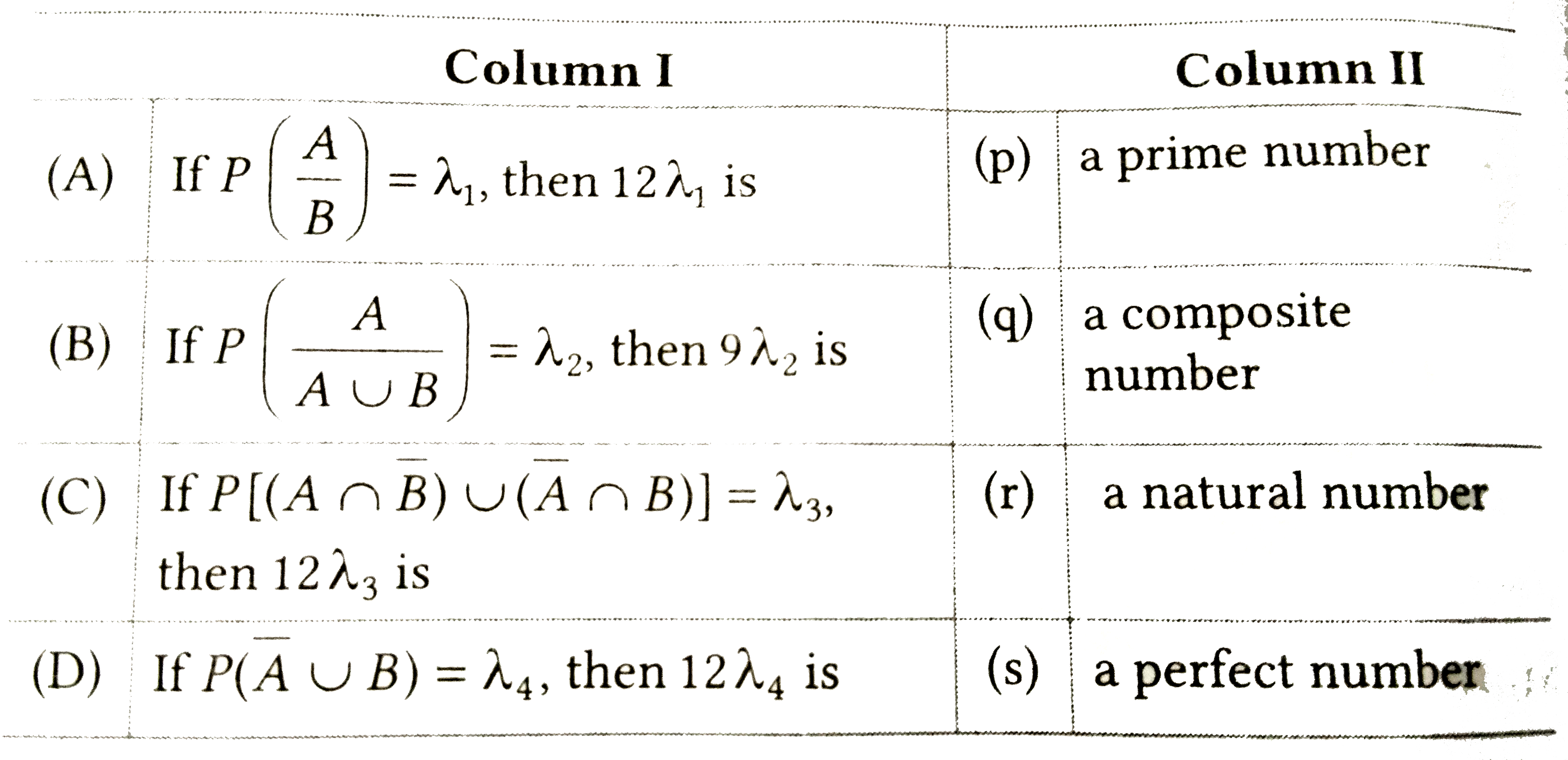
The correct Answer is:A-s;B-p;C-r;D-q
To solve the problem, we need to find the probability of the intersection of two independent events A and B, given their individual probabilities.
Step 1: Understand the given information
- We are given:
- P(A)=35
- P(B)=23
- We know that events A and B are independent.
Step 2: Use the formula for independent events
- For two independent events A and B, the probability of their intersection is given by:
P(A∩B)=P(A)×P(B)
Step 3: Substitute the values into the formula
- Now, we substitute the given probabilities into the formula:
P(A∩B)=P(A)×P(B)=35×23
Step 4: Perform the multiplication
- Multiply the fractions:
P(A∩B)=3×25×3=615
Step 5: Simplify the fraction
- Simplifying 615:
P(A∩B)=25
Final Answer:
- Therefore, the probability of the intersection of events A and B is:
P(A∩B)=25
- We are given:
- P(A)=35
- P(B)=23
- We know that events A and B are independent.
- For two independent events A and B, the probability of their intersection is given by:
P(A∩B)=P(A)×P(B)
- Now, we substitute the given probabilities into the formula:
P(A∩B)=P(A)×P(B)=35×23
- Multiply the fractions:
P(A∩B)=3×25×3=615
- Simplifying 615:
P(A∩B)=25
- Therefore, the probability of the intersection of events A and B is:
P(A∩B)=25
Topper's Solved these Questions
- PROBABILITYBOOK - ARIHANT MATHS ENGLISHCHAPTER - PROBABILITYEXERCISE - Exercise (Statement I And Ii Type Questions)
11 Videos
- PROBABILITYBOOK - ARIHANT MATHS ENGLISHCHAPTER - PROBABILITYEXERCISE - Exercise (Subjective Type Questions)
15 Videos
- PROBABILITYBOOK - ARIHANT MATHS ENGLISHCHAPTER - PROBABILITYEXERCISE - Exercise (Single Integer Answer Type Questions)
10 Videos
- PERMUTATIONS AND COMBINATIONS BOOK - ARIHANT MATHS ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
28 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHS ENGLISHCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
51 Videos
Similar Questions
If A and B are two events such that P(A) = 3/4 and P(B) = 5/8, then
View SolutionIf P(A)=25 and P(B)=13 and A and B are independent events, then find P(A∩B).
View SolutionIf A and B are two independent events, such that P(A)=13andP(B)=14.
View SolutionIf A and B are two events such that 2 P(A)=P(B)=513f∈dP(A∩B) .
View SolutionLet A and B be two events such that
P(A)=12,P(B)=pandP(A∪B)=35
find 'p' if A and B are independent events.
View SolutionA and B are two events such that P(A)=35,P(B)=310 and P(A∪B)=12
Find whether A and B are independent or not.
View SolutionIf A and B are two events such that 2P(A)=P(B)=513 and P(AB)=25, find P(A∪B).
View SolutionLet A and B be two events such that
P(A)=12,P(B)=pandP(A∪B)=35
find 'p' if A and b are independent events.
View Solutionif A and B be two events such that P(A)=14,P(B)=13 and P(A∪B)=12, show that A and B are independent events.
View SolutionIf A and B be two events such that P(A)=1/4, P(B)=1/3 and P(A∪B)=1/2 show that A and B are independent events.
View Solution
