is a rectangle formed by joining the points and and are the mid-points of sides and respectively. Is the quadrilateral a square? a rectangle? or a rhombus? Justify your answer.
Given vertices of a rectangle are A(-1, -1), B(-1, 4), C(5, 4) and D(5, -1).
Mid-point of AB is
Mid-point of BC is
Mid-point of CD is
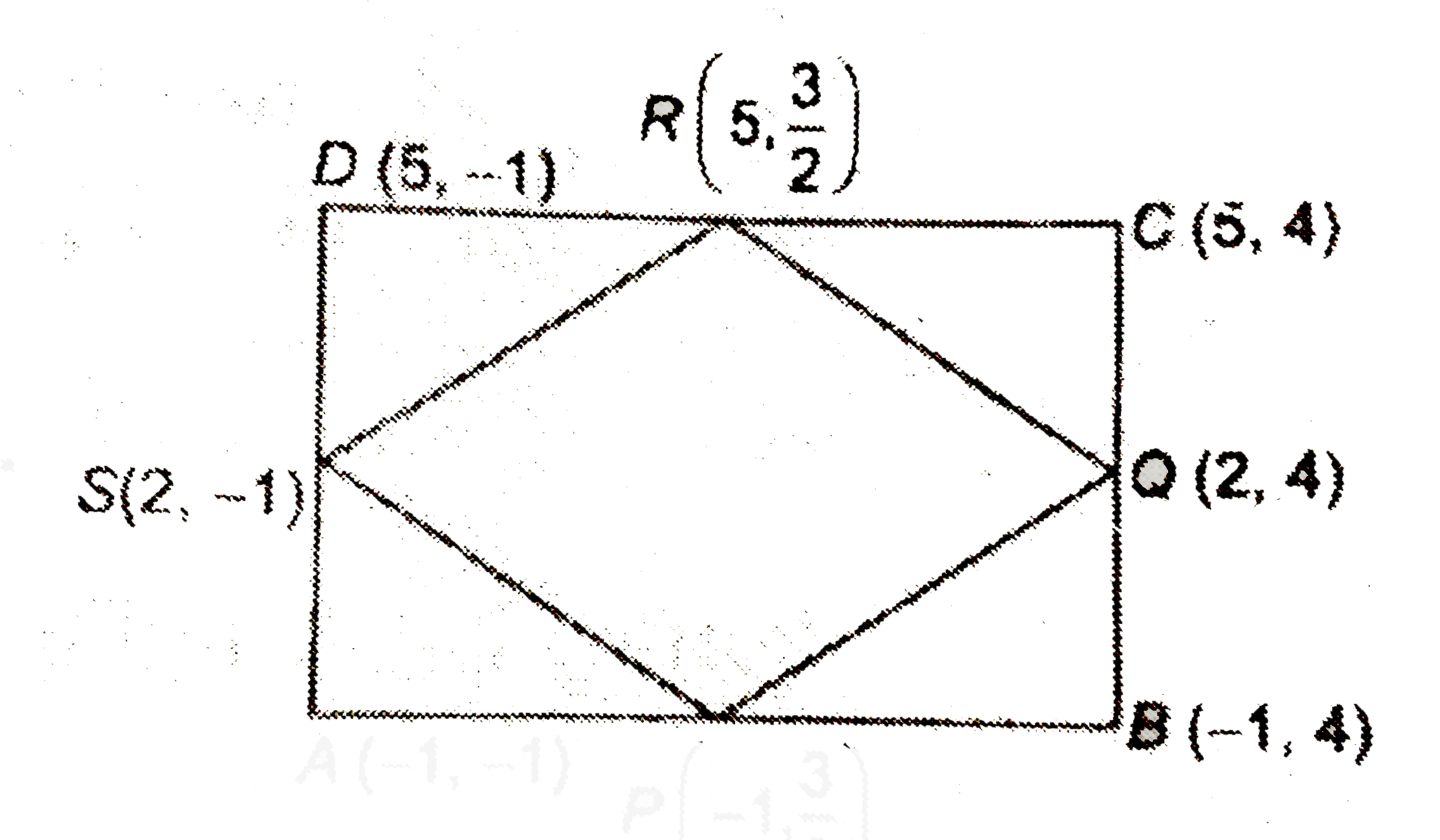
Mid-point of AD is .
Now ,
Now,
and
Since, all the sides are equal but diagonals are not equal.
is rhombus.
Topper's Solved these Questions
- CO-ORDINATE GEOMETRYBOOK - NAGEEN PRAKASHANCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Exercise 7a
20 Videos
- CO-ORDINATE GEOMETRYBOOK - NAGEEN PRAKASHANCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Exercise 7b
18 Videos
- CO-ORDINATE GEOMETRYBOOK - NAGEEN PRAKASHANCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Miscellaneous Examples
8 Videos
- CIRCLESBOOK - NAGEEN PRAKASHANCHAPTER - CIRCLESEXERCISE - Revision Exercise Long Answer Questions
3 Videos
- CONSTRUCTIONSBOOK - NAGEEN PRAKASHANCHAPTER - CONSTRUCTIONSEXERCISE - PROBLEMS FROM NCERT/ EXEMPLAR
10 Videos
Similar Questions
ABCD is a rectangle formed by the points A(1, 1),B(1, 4),C(5, 4) and D(5, 1). P, Q, R and S are the midpoints of AB, BC, CD and DA respectively. Is the quadrilateral PQRS a square? A rectangle? or a rhombus? Justify your answer.
View SolutionABCD is a rhombus and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
View SolutionABCD is rectangle formed by the points A(-1, -1), B(-1, 4), C(5, 4) and D(5, -1). If P, Q, R and S be the midpoint of AB, BC, CD and DA respectively, show that PQRS is a rhombus.
View SolutionABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
View SolutionABCD is a rhombus and P,Q,R,S are the mid-points of AB,BC,CD,DA respectively. Prove that PQRS is a rectangle.
View SolutionIn an equilateral triangle ABC, D, E, F are the mid-points of AB, BC and AC respectively.The quadrilateral BEFD is are a
(A) Square
(B) Parallelogram
(C) Rectangle
(D) Rhombus
View SolutionIn Fig. 32, ABCD is a rectangle of length AB=40 cm and breadth BC=25 cm. If P,Q,R,S be the mid-points of the sides AB, BC, CD and DA respectively, find the area of the shaded region.
View SolutionShow that the points A(2,-2), B(8, 4), C(5,7) and D(-1, 1) are the angular points of a rectangle.
View SolutionIn a ABC,EandF are the mid-points of ACandAB respectively. The altitude AP to BC intersects FE at Q Prove that AQ=QP
View SolutionIf D,E,F are the mid-points of the sides BC,CAandAB respectively of a triangle ABC, prove by vector method that AreaofDEF=14(areaofABC).
View Solution
