A chord of the circle $x^{2} + y^{2} - 4 x - 6 y = 0$ passing through the origin subtends an angle arctan(7/4) at the point where the circle meets positive y-axis. Equation of the chord is

Text Solution

Verified by Experts

Chord OA subtends an angle $θ = {tan}^{- 1} (\frac{7}{4})$ at point B on y-axis.
From the geometrical properties of the circle, we know that
$∠ A O B = ∠ A O T$ , where OT is tangent at origin.

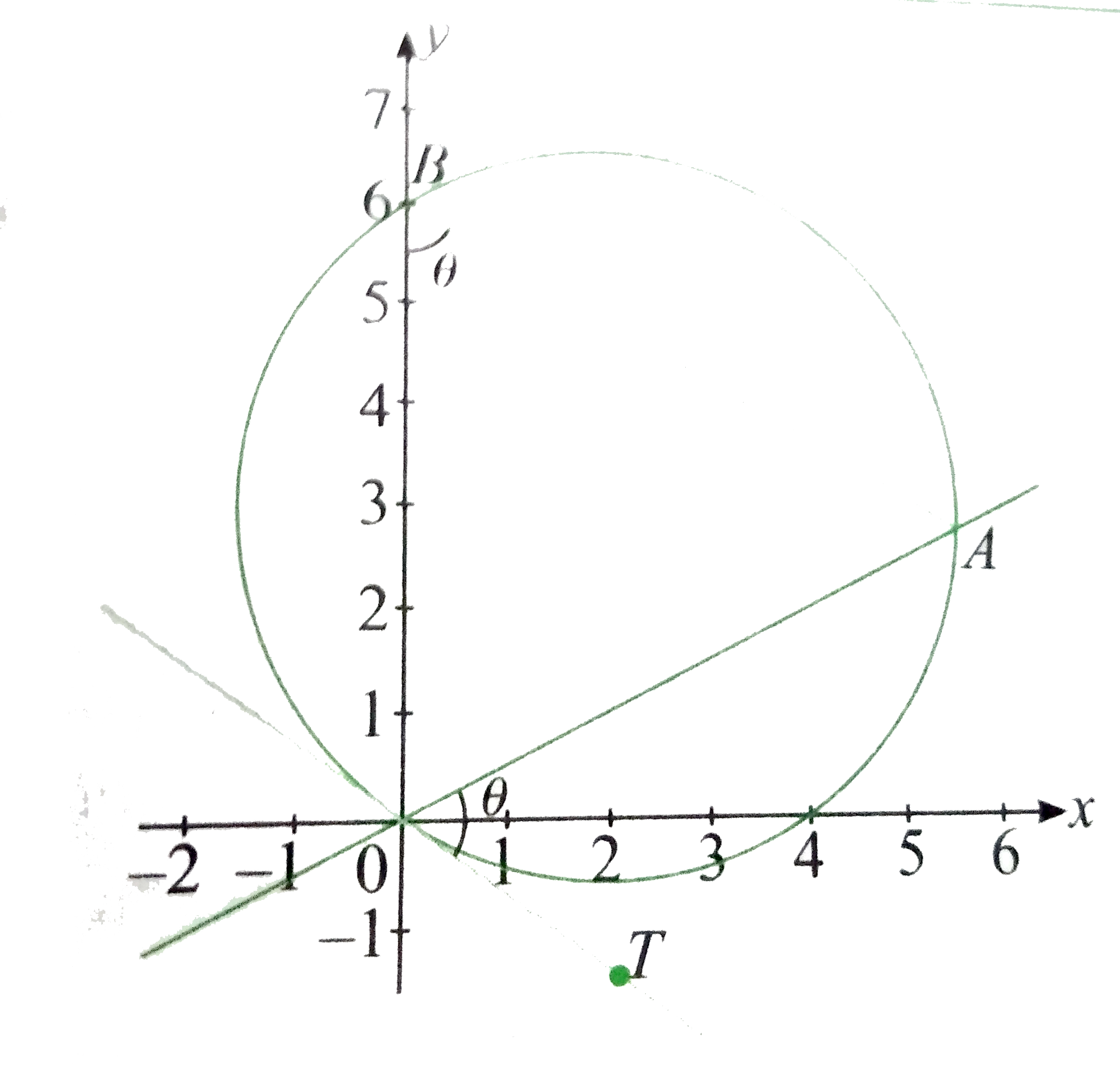
Equation of tangent at (0,0) is
$- 2 (x + 0) - 3 (y + 0) = 0$
or $2 x + 3 y = 0$
Let the slope of OA be m.
$∴ \frac{7}{4} = ∣ ∣ ∣ ∣ \frac{m + \frac{2}{3}}{1 - \frac{2}{3} m} ∣ ∣ ∣ ∣$
$\Rightarrow \frac{7}{4} = ∣ ∣ ∣ \frac{3 m + 2}{3 - 2 m} ∣ ∣ ∣$
$\Rightarrow 21 - 14 m = 12 m + 8$ (Taking positive sign)
$\Rightarrow 26 m = 13$
$\Rightarrow m = 1 / 2$
Therefore , the equation of chord $O A$ is $x - 2 y = 0$ .

The common chord of the circles $x^{2} + y^{2} - 4 x - 4 y = 0$ and $x^{2} + y^{2} - 16 = 0$ subtends at the origin an angle equal to
A $30^{\circ}$
B $45^{\circ}$
C $60^{\circ}$
D $90^{\circ}$
The common chord of the circles $x^{2} + y^{2} - 4 x - 4 y = 0$ and $2 x^{2} + 2 y^{2} = 32$ subtends at the origin an angle equal to
A $\frac{π}{2}$
B $\frac{π}{4}$
C $\frac{π}{6}$
D $\frac{π}{3}$
If a circle is concentric with the circle $x^{2} + y^{2} - 4 x - 6 y + 9 = 0$ and passes through the point (-4,-5) then its equation is
A $x^{2} + y^{2} + 4 x + 6 y - 87 = 0$
B $x^{2} + y^{2} + 4 x + 6 y + 87 = 0$
C $x^{2} + y^{2} - 4 x - 6 y - 87 = 0$
D $x^{2} + y^{2} - 4 x - 6 y + 87 = 0$