A circle passes through the points (0,0) and (0, 1) and also touches the circie x 2 + y 2 = 16 The radius of the circle is

A

1

B

2

C

3

D

4

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:B

Let S ≡ x 2 + y 2 = 16
At point (0, 0 )
S ≡ 0 + 0 - 16 = - 16 < 0
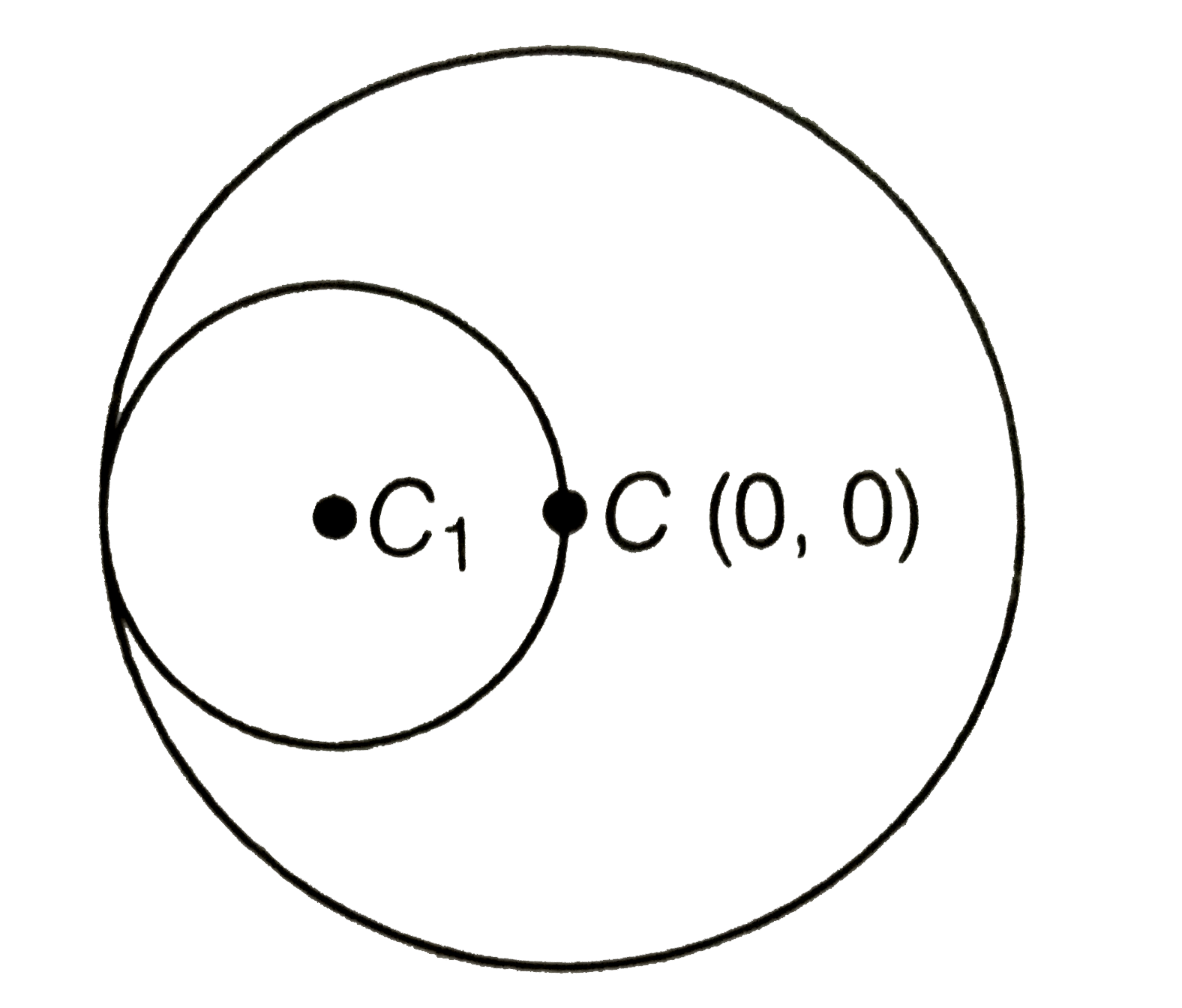
ocr_image
At point ( 0 , 1 )
S ≡ 0 + 1 - 16 = - 15 < 0
Thus, both points lie inside the circle.
It means required circle touch inside the given circle.
So , the centre and radius of given circle are C ( 0 , 0 ) and r = 4 .
Since, the required circle is passing through centre (0, 0)
∴ Diameter of required circle = 4
and radius of required circle = 4 2 = 2

Updated on:21/07/2023
|
Class 12MATHSQUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Statement-1: The centre of the circle passing through the points (0, 0), (1, 0) and touching the circle C:x2+y2=9 lies inside the circle.
    Statement-2: If a circle C1 passes through the centre of the circle C2 and also touches the circle, the radius of the circle C2 is twice the radius of circle C1

    AStatement-1 is True, Statement-2 is True, Statement-2 is a correct explanation for Statement-1.
    BStatement-1 is True, Statement-2 is not a correct explanation for Statement-1.
    CStatement-1 is True, Statement-2 is False.
    DStatement-1 is False, Statement-2 is True.

  • The centre of a circle passing through the points (0, 0), (1, 0) and touching the circle x2+y2=9, is

    A(32,12)
    B(12,32)
    C(12,12)
    D(12,−21/2)

  • Centre of a circle passing through point (0,1) and touching the curve y=x2 at (2,4) is

    A(165,5310)
    B(−165,5310)
    C(−165,−5310)
    D(165,−5310)