A circular flower bed is surrounded by a path 4m wide. The diameter of the flower bed is 66m. What is the area of this path ? (π=3.14)
To find the area of the path surrounding the circular flower bed, we can follow these steps:
Step 1: Calculate the radius of the inner circle (flower bed)
The diameter of the flower bed is given as 66 m. The radius is half of the diameter.
Radius of inner circle=Diameter2=66m2=33m
Step 2: Calculate the radius of the outer circle (flower bed + path)
The path surrounding the flower bed is 4 m wide. Therefore, we add this width to the radius of the inner circle.
Radius of outer circle=Radius of inner circle+Width of path=33m+4m=37m
Step 3: Calculate the area of the outer circle
The area of a circle is given by the formula Area=πr2.
Area of outer circle=π×(37m)2=3.14×1369m2=4290.06m2
Step 4: Calculate the area of the inner circle
Using the same area formula for the inner circle:
Area of inner circle=π×(33m)2=3.14×1089m2=3427.26m2
Step 5: Calculate the area of the path
The area of the path is the difference between the area of the outer circle and the area of the inner circle.
Area of path=Area of outer circle−Area of inner circle=4290.06m2−3427.26m2=862.8m2
Final Result
The area of the path is 862.8m2.
---
The diameter of the flower bed is given as 66 m. The radius is half of the diameter.
Radius of inner circle=Diameter2=66m2=33m
The path surrounding the flower bed is 4 m wide. Therefore, we add this width to the radius of the inner circle.
Radius of outer circle=Radius of inner circle+Width of path=33m+4m=37m
The area of a circle is given by the formula Area=πr2.
Area of outer circle=π×(37m)2=3.14×1369m2=4290.06m2
Using the same area formula for the inner circle:
Area of inner circle=π×(33m)2=3.14×1089m2=3427.26m2
The area of the path is the difference between the area of the outer circle and the area of the inner circle.
Area of path=Area of outer circle−Area of inner circle=4290.06m2−3427.26m2=862.8m2
The area of the path is 862.8m2.
Topper's Solved these Questions
- PERIMETER AND AREABOOK - NCERTCHAPTER - PERIMETER AND AREAEXERCISE - EXERCISE 11.4
8 Videos
- PERIMETER AND AREABOOK - NCERTCHAPTER - PERIMETER AND AREAEXERCISE - SOLVED EXAMPLES
22 Videos
- PERIMETER AND AREABOOK - NCERTCHAPTER - PERIMETER AND AREAEXERCISE - EXERCISE 11.2
8 Videos
- LINES AND ANGLESBOOK - NCERTCHAPTER - LINES AND ANGLESEXERCISE - SOLVED EXAMPLES
1 Videos
- RATIONAL NUMBERSBOOK - NCERTCHAPTER - RATIONAL NUMBERSEXERCISE - SOLVED EXAMPLES
6 Videos
Similar Questions
A circular flower bed is surrounded by a path 4m wide. The diameter of the flower bed is 66m. What is the area of this path ? (π=3.14)
View SolutionA circular garden is surrounded by 3 m wide path. The circumference of the garden excluding the path is 88 m.
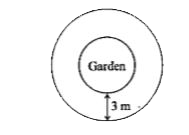
What is the area of the path surrounding the garden?
View Solution
Knowledge Check
A circular grass plot 40 m in radius is surrounded by a path. If the area of the grass plot is twice the area of the path, the width of the path in metres would be
A40(1+√23)B40(1−√23)C40(√32−1)D40(√32+1)
A square lawn is surrounded by a path 2.5 m wide. If the area of the path is 165 m2 , find the area of the lawn.
View SolutionA square lawn is surrounded by a path 2.5 m wide. If the area of the path is 165m2 find the area of the lawn.
View SolutionA lawn 30m long and 16m wide is surrounded by a path 2m wide. What is the area of the path
View SolutionA circular water fountain 6.6 m in diameter is surrounded by a path of width 1.5 m. The area of this path in square metres is-
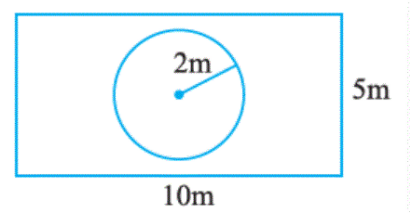
View SolutionThe adjoining figure represents a rectangular lawn with a circular flower bed in the middle. Find:
(i) the area of the whole land
(ii) the area of the flower bed
(iii) the area of the lawn excluding the area of the flower bed
(iv) the circumference of the flower bed.
View SolutionA rectangular grassy lawn is 18 m by 12 m. It has a gravel path 1.5 m wide all around it on the outside. What is the area of the path .
View SolutionA square lawn with side 100 m long has a circular flower bed in the centre. If the area of the lawn, excluding the flower bed, is 8614 m2, the radius of the circular flower bed is (a) 21 m (b) 31 m (c) 41 m (d) None of these
View Solution
