A coin is tossed 5 times. If X is the number of heads observed, fid the probability distribution of X.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:N/a

Here $n = 3$
Probability of getting a head on one coin
$p = \frac{1}{2}$
$∴ q =$ probability of not getting a head $= 1 - p$
$\Rightarrow q = 1 - \frac{1}{2} = \frac{1}{2}$
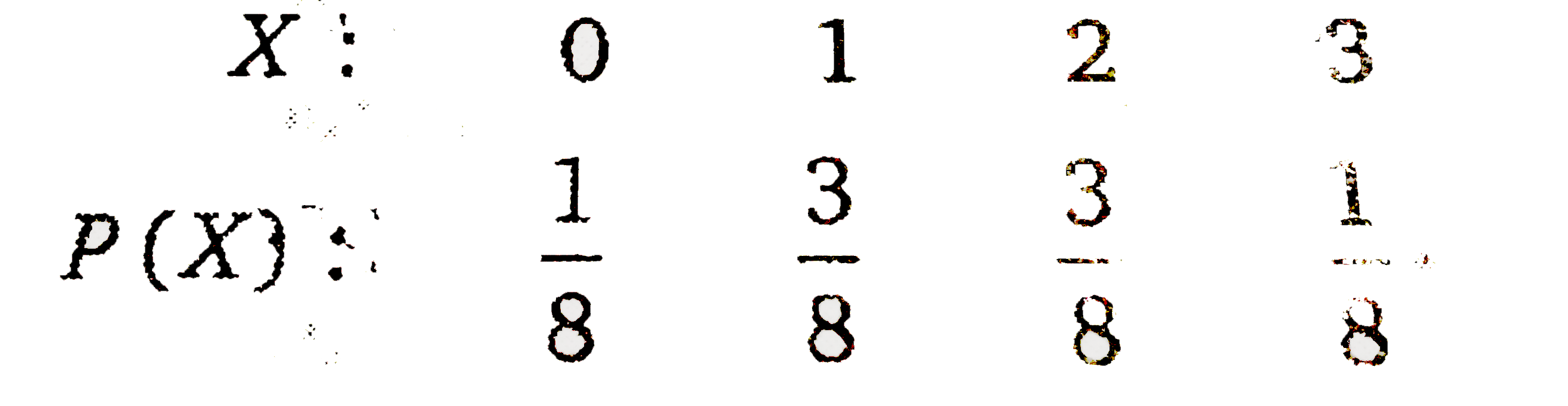
$P (X = 0) = .^{3} C_{0} p^{0} . q^{3} = 1 \times 1 \times {(\frac{1}{2})}^{3} = \frac{1}{8}$
$P (X = 1) = .^{3} C_{1} . p^{1} . q^{2} = 3 \times \frac{1}{2} \times {(\frac{1}{2})}^{2} = \frac{3}{8}$
$P (X = 2) = .^{3} C_{2} . p^{2} . q^{1} = 3 \times {(\frac{1}{2})}^{2} \times \frac{1}{2} = \frac{3}{8}$
$P (X = 3) =^{3} C_{3} . p^{3} . q^{0} = 1 \times {(\frac{1}{2})}^{3} = \frac{1}{8}$
Therefore probability distribution is:

|

Updated on:21/07/2023

Class 12 MATHS PROBABILITY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A fair coin is tossed 4 times. If X denotes the number of heads obtained, then the formula for p.m.f. of X is
A $f (x) = {\begin{matrix} \frac{1}{16}^{4} C_{x}, x = 0, 1, 2, 3, 4 0, otherwise \end{matrix}$
B $f (x) = {\begin{matrix} \frac{1}{8}^{4} C_{x}, x = 0, 1, 2, 3, 4 0, otherwise \end{matrix}$
C $f (x) = {\begin{matrix} \frac{1}{4}^{4} C_{x}, x = 0, 1, 2, 3, 4 0, otherwise \end{matrix}$
D $f (x) = {\begin{matrix} \frac{1}{2}^{4} C_{x}, x = 0, 1, 2, 3, 4 0, otherwise \end{matrix}$
Question 2 - Select One
A unbaised coin is tossed n times. If the probability of getting 5 heads is equal to the probability of getting 6 heads then probability of getting 3 heads is
A $^{11} C_{5} {(\frac{1}{2})}^{5}$
B $^{11} C_{6} {(\frac{1}{2})}^{6}$
C $^{11} C_{3} {(\frac{1}{2})}^{11}$
D $\frac{11}{1024}$

NAGEEN PRAKASHAN-PROBABIILITY-Miscellaneous Exercise

Home
Ask Doubt
Profile