A diagonal of rhombus $A B C D$ is member of both the families of lines $(x + y - 1) + λ (2 x + 3 y - 2) = 0$ and rhombus is (3, 2). If the area of the rhombus is $12 \sqrt{5}$ sq. units, then find the remaining vertices of the rhombus.

Video Solution

Text Solution

Verified by Experts

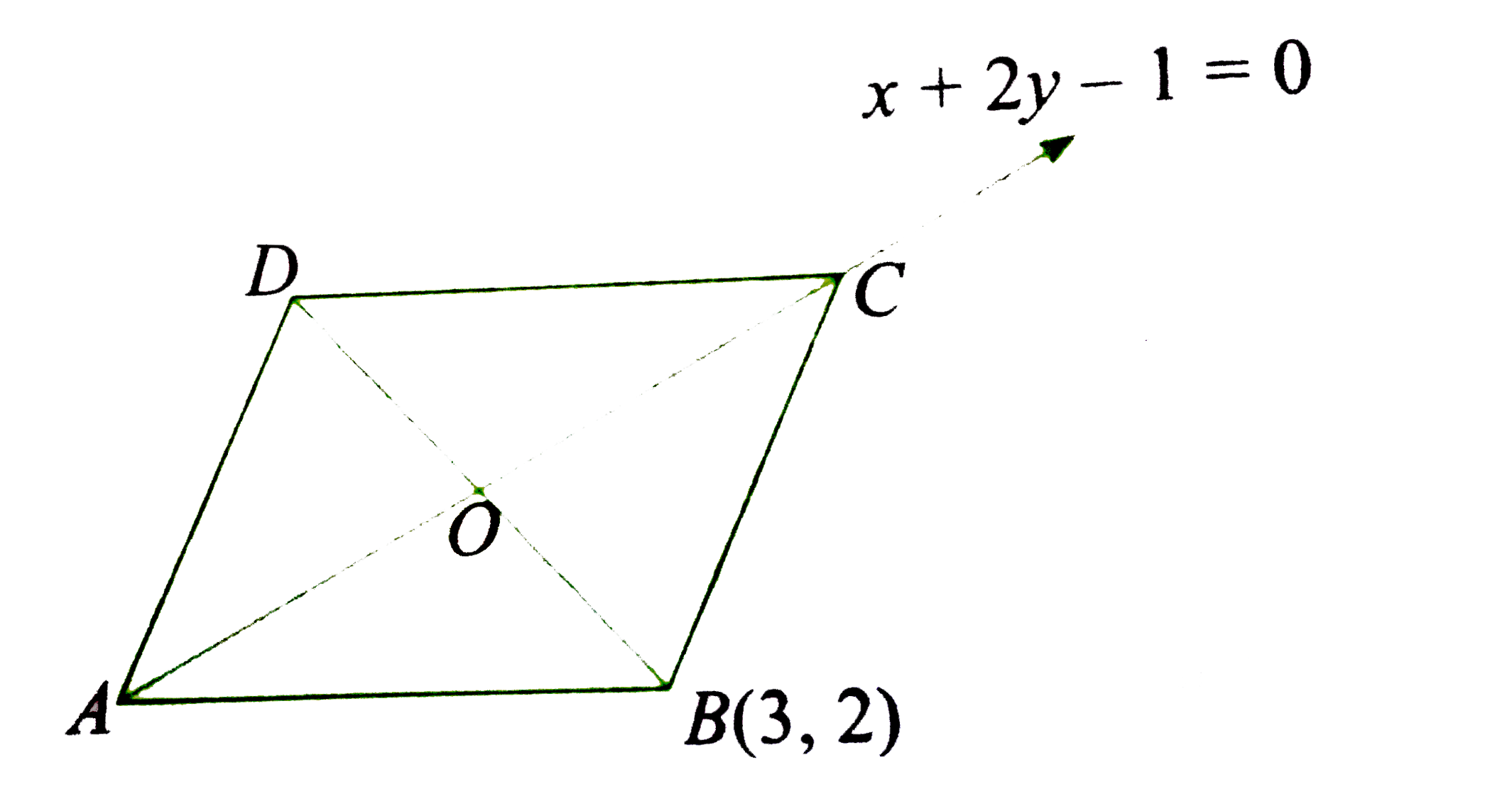
Since diagonal is member of both the given families of line, it will pass through the points (1,0) and (-1,1) which are points where given family of lines are concurrent.
So, equation of diagonal AC is x+2y-1=0.
Clearly, point (3,2) does not satisfy this diagonal.
$∴ Let B \equiv (3, 2) .$
Diagonal BD is perpendicular to AC.
So, equation of diagonal BD is 2x-y-4=0.
Point of intersection of diagonal AC and BD is O $(\frac{9}{5}, \frac{- 2}{5}) .$
Since O is midpoint of BD, D $\equiv (\frac{3}{5}, - \frac{14}{5}) .$
$Now, O D = \frac{6}{\sqrt{5}} . S o, B D = \frac{12}{\sqrt{5}} = d_{1}$
$Area of rhombus = \frac{1}{2} d_{1} \times d_{2} = 12 \sqrt{5} (where d_{2} is length of diagonal AC)$
$∴ d_{2} = 10 units$
This is the length of diagonal AC.
Slope of AC $= - \frac{1}{2} = tan θ, where θ$ is inclination of AC with x-axis.
Since OA=OC=5, using parametic from of straight line, we have
$A, C \equiv (\frac{9}{5} \pm 5 cos θ, - \frac{2}{5} \pm 5 sin θ)$
$\equiv (\frac{9}{5} \pm 5 (- \frac{2}{\sqrt{5}}), - \frac{2}{5} \pm 5 (\frac{1}{\sqrt{5}}))$
$\equiv (\frac{9}{5} - 2 \sqrt{5}, - \frac{2}{5} + \sqrt{5}), (\frac{9}{5} + 2 \sqrt{5}, - \frac{2}{5} - \sqrt{5})$

Updated on:21/07/2023