A doctor is called to see a sick child. The doctor knows (prior to the visit) that 90% of the sick children in that neighbourhood are sick with the flu, denoted by F, while 10% are sick with the measles, denoted by M. A well-known symptom of measles is a rash, denoted by R. The probability having a rash for a child sick with the measles is 0.95. however, occasionally children with the flu also develop a rash, with conditional probability 0.08. upon examination the child, the doctor finds a rash. The what is the probability that the child has the measles? 91/165 b. 90/163 c. 82/161 d. 95/167
91/165
90/163
82/161
95/167
The correct Answer is:D
To solve the problem, we will use Bayes' theorem, which allows us to find the probability of an event based on prior knowledge of conditions related to the event.
1. Define Events:
- Let M be the event that the child has measles.
- Let F be the event that the child has the flu.
- Let R be the event that the child has a rash.
2. Given Probabilities:
- P(M)=0.10 (Probability that the child has measles)
- P(F)=0.90 (Probability that the child has the flu)
- P(R|M)=0.95 (Probability of having a rash given measles)
- P(R|F)=0.08 (Probability of having a rash given flu)
3. Use Bayes' Theorem:
We want to find P(M|R), the probability that the child has measles given that the child has a rash. According to Bayes' theorem:
P(M|R)=P(R|M)⋅P(M)P(R)
4. Calculate P(R):
To find P(R), we use the law of total probability:
P(R)=P(R|M)⋅P(M)+P(R|F)⋅P(F)
Substituting the known values:
P(R)=(0.95⋅0.10)+(0.08⋅0.90)
P(R)=0.095+0.072=0.167
5. Substitute into Bayes' Theorem:
Now substitute P(R) back into Bayes' theorem:
P(M|R)=0.95⋅0.100.167
P(M|R)=0.0950.167
6. Calculate the Final Probability:
Now, we can calculate 0.0950.167:
P(M|R)=95167
Conclusion:
The probability that the child has measles given that the child has a rash is 95167.
- Let M be the event that the child has measles.
- Let F be the event that the child has the flu.
- Let R be the event that the child has a rash.
- P(M)=0.10 (Probability that the child has measles)
- P(F)=0.90 (Probability that the child has the flu)
- P(R|M)=0.95 (Probability of having a rash given measles)
- P(R|F)=0.08 (Probability of having a rash given flu)
We want to find P(M|R), the probability that the child has measles given that the child has a rash. According to Bayes' theorem:
To find P(R), we use the law of total probability:
P(R)=0.095+0.072=0.167
Now substitute P(R) back into Bayes' theorem:
P(M|R)=0.0950.167
Now, we can calculate 0.0950.167:
The probability that the child has measles given that the child has a rash is 95167.
Topper's Solved these Questions
- PROBABILITY IIBOOK - CENGAGE ENGLISHCHAPTER - PROBABILITY IIEXERCISE - MULTIPLE CHOICE ANSWER TYPE
17 Videos
- PROBABILITY IIBOOK - CENGAGE ENGLISHCHAPTER - PROBABILITY IIEXERCISE - LINKED COMPREHENSION TYPE
43 Videos
- PROBABILITY IIBOOK - CENGAGE ENGLISHCHAPTER - PROBABILITY IIEXERCISE - CONCEPT APPCICATION EXERCISE 14.6
5 Videos
- PROBABILITY I BOOK - CENGAGE ENGLISHCHAPTER - PROBABILITY I EXERCISE - JEE Advanced
7 Videos
- PROGRESSION AND SERIESBOOK - CENGAGE ENGLISHCHAPTER - PROGRESSION AND SERIESEXERCISE - ARCHIVES (MATRIX MATCH TYPE )
1 Videos
Similar Questions
Knowledge Check
A family has three children.Assuming that the probability of having a boy is 0.5, what is the probability that at least one child is a boy?
A0.875B0.67C0.5D0.375The vector of sleeping sickness' is :
Aa) house flyBb) sand flyCc) mosquitoDd) tsetse fly
How many times must a man toss a fair com so that the probability of having at least one head is more than 90%?
View SolutionThe probability that a person visiting a zoo will see the girafee is 0.72, the probability that he will see the bears is 0.84 and the probability that he will see both is 0.52.
View SolutionWrite the causative agent and the most important symptom of the following disease:
Measles
View SolutionA couple has two children. Find the probability that both the children are (i) males, if it is known that at least one of the children is male. (ii) females, if it is known that the elder child is a female.
View SolutionA couple has two children. Find the probability that both the children are (i) males, if it si known that at least one of the children is male. (ii) females, if it is known that the elder child is a female.
View SolutionThe domain of the function f(x) is denoted by Df
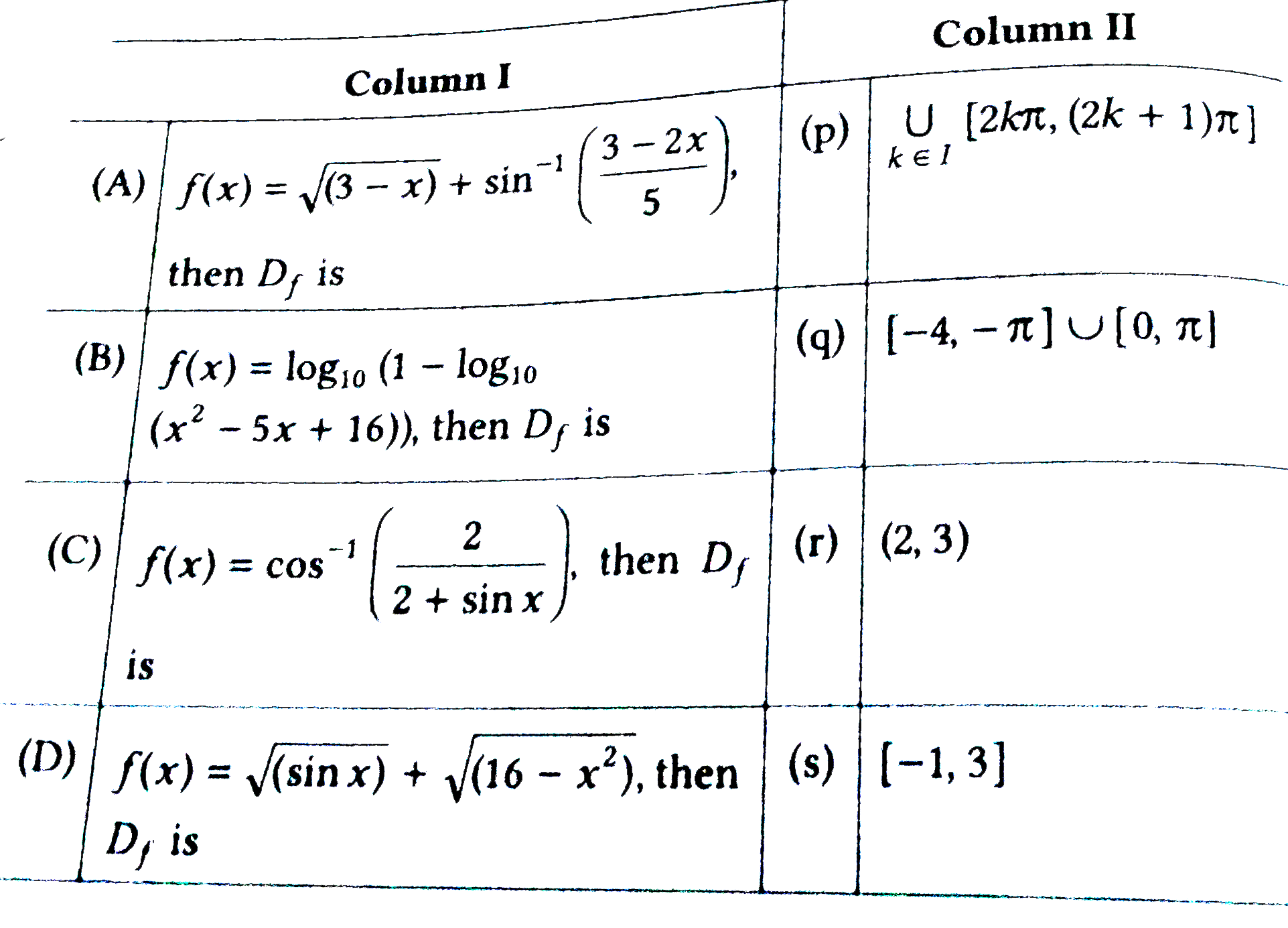
View Solution
