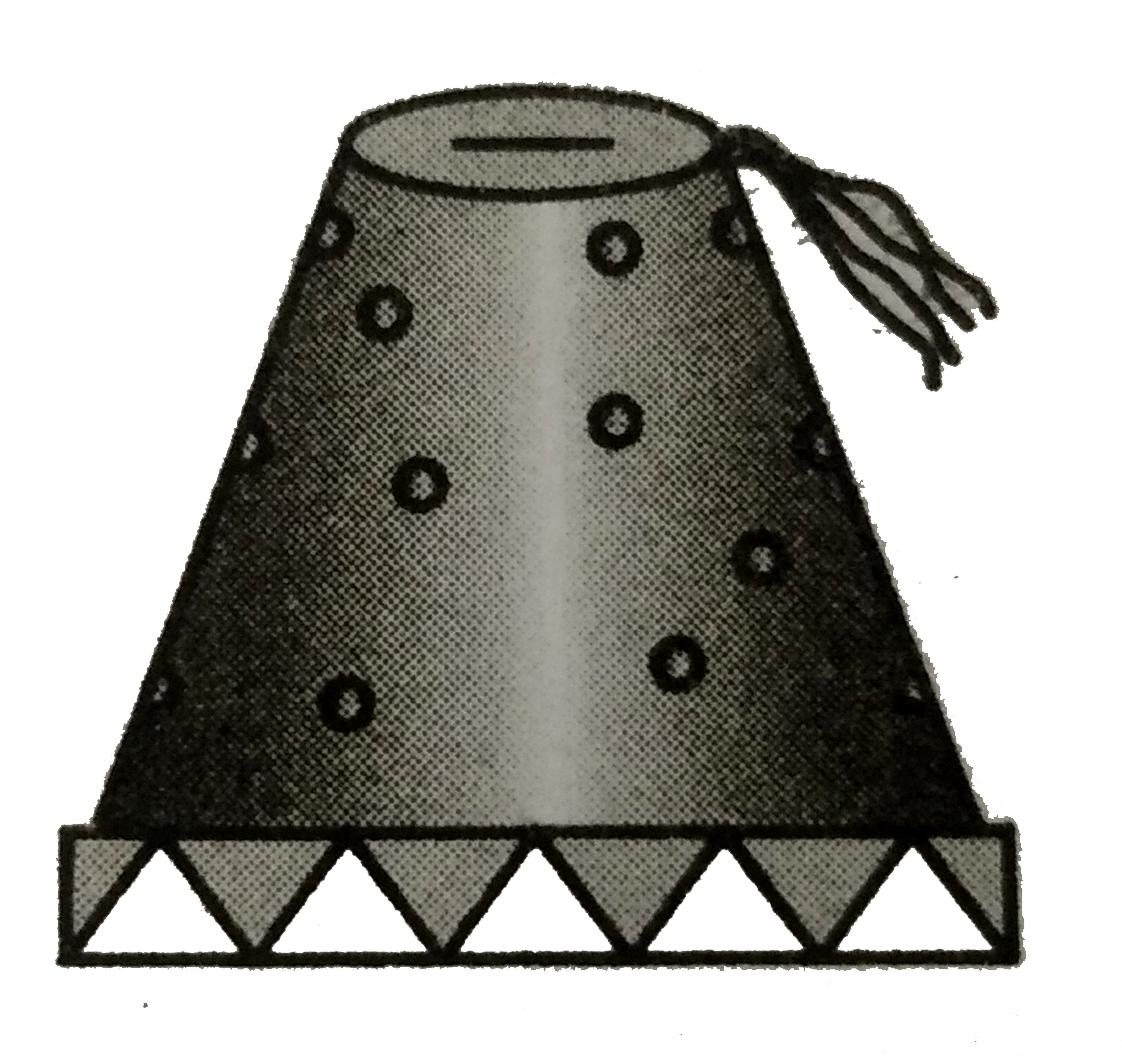
A Fez , the cap used by the Turks is shaped like the frustum of a cone (see fig ) . If its radius on the open side is 10cm , radius at the upper base is 4cm and its slant height is 15cm , find the area of material used for making it. Solution in Kannada
The correct Answer is:
Step by step video, text & image solution for A Fez , the cap used by the Turks is shaped like the frustum of a cone (see fig ) . If its radius on the open side is 10cm , radius at the upper base is 4cm and its slant height is 15cm , find the area of material used for making it. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SURFACE AREAS AND VOLUMES BOOK - SUBHASH PUBLICATIONCHAPTER - SURFACE AREAS AND VOLUMES EXERCISE - EXERCISE 15.5
4 Videos
- SURFACE AREAS AND VOLUMES BOOK - SUBHASH PUBLICATIONCHAPTER - SURFACE AREAS AND VOLUMES EXERCISE - EXERCISE 15.3
9 Videos
- STATISTICS BOOK - SUBHASH PUBLICATIONCHAPTER - STATISTICS EXERCISE - EXERCISE 13.4
3 Videos
- TRIANGLES BOOK - SUBHASH PUBLICATIONCHAPTER - TRIANGLES EXERCISE - EXERCISE 2.6 (OPTIONAL)
15 Videos
Similar Questions
A copper wire, 3mm in diameter , is wound about a cylinder whose length is 12cm, and diameter 10cm, so as to cover the curved surface of the cylinder . Find the length and mass of the wire, assuming the density of copper to be 8.88gpercm3
View SolutionA metallic sphere of radius 4.2cm is melted and recast into the shape of a cylinder of radius 6cm. Find the height of the cylinder.
View SolutionThe following distribution gives the daily income of 50 workers of a factory.
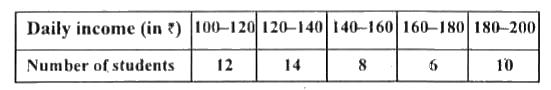
Convert the distribution above to a less than type cumulative frequency distribution, and draw its ogive.
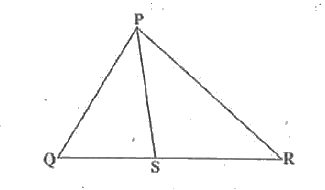
View SolutionIn Fig PS is the bisector of ∠QPR of ΔPQR . Prove that QSSR=PQPR
View SolutionA fez, the cap used by the Turks, is shaped like the frustum of a cone. If its radius on the open side is 10 cm, radius at the upper base is 4 cm and its slant height is 15 cm, find the area of material used for making it.
View SolutionThe height of a cone is 15 cm. If its volume is 1570 cm3, find the radius of the base.
View SolutionA fez, the headgear cap used by the trucks is shaped like the frustum of a cone. If its radius on the open side is 10 cm, radius at the upper base is 4 cm and its slant height is 15 cm, find the area of material used for making it.
View Solutionएक तुर्की टोपी शंकु के एक छन्ने के आकर की है (देखिए अकृतिये) । यदि इसके खुले सिरे की त्रिज्या 10 सेमी है, ऊपरी सिरे की त्रिज्या 4 सेमी है और टोपी की तियक ऊंचाई 15 सेमी है, तो इसके बनाने में प्रयुक्त पदार्थ का क्षेत्रफल ज्ञात कीजिए ।

View Solutionएक तुर्की टोपी शंकु के एक छिन्नक के आकार की है | यदि इसके खुले सिरे की त्रिज्या 10 सेमी है, ऊपरी सिरे की त्रिज्या 4 सेमी है और टोपी की तिर्यक ऊँचाई 15 सेमी है तो इसके बनाने में प्रयुक्त पदार्थ का क्षेत्रफल ज्ञात कीजिए |
View Solutionएक तुर्की टोपी शंकु के एक छिन्नक के आकर की है। यदि इसके खुले सिरे की त्रिज्या 10cm है, ऊपरी सिरे की त्रिज्या 4cm है और टोपी की तिर्यक उचाई 15cm हैम तो इसके बनाने में प्रयुक्त पर्दार्थ का क्षेत्रफल ज्ञात कीजिएः
View Solutionएक तुर्की टोपी शंकु के एक छिन्नक के आकार की है (देखिए आकृति ) । यदि इसके खुले सिरे की त्रिज्या 10 सेमी है , ऊपरी सिरे की त्रिज्या 4 सेमी है । और टोपी की तिर्यक ऊंचाई 15 सेमी है , तो इसके बनाने में प्रयुक्त पदार्थ का क्षेत्रफल ज्ञात कीजिए ।
View SolutionHeight of the cone =3m and radius of its base =4m then the slant height of the cone will be -
View SolutionThe slant height of a frustum of a cone is 4cm and the perimeters of its circular ends are 18cm and 6cm . Find the curved surface areas of the frustum.
View SolutionA fex, the cap used by the Turks, is shaped like the frustum of a cone. If its radius on the open side is 10cm, radius at the upper base is 4cm and its slant height is 15cm, find the area of material used for making it.
View SolutionA fez, the cap used by the Turks, is shaped like the frustum of a cone. If its radius on the open side is 10 cm, radius at the upper base is 4 cm and its slant height is 15 cm, find the area of material used for making it.
View Solution
