A firm manufactures two types of products, A and B, and sells them at a profil of 3 per unit to type B product and 5 perunit of type A product. Both product is processed on two machines M1and M2 One unit of type A requires one minute of processing time on M1 and two minutes of processing time on M2i whereas one unit of type B requires one minute of processing time on M1 and one minute on M Machines sells them at a profit of 5 per unit of type A and M, and M, are respectively available for at most 5 hours and 6 hours in a day. Find out how many units of each type of product the firn should produce a day in order to maximize the profit. Solve the problem graphically [CHSE 2000]
Let x units of A and y units of B be produced in order to have a maximum profit.
Then, clearly x≥0andy≥0. x unit of A and y units of B will take (x+y) minutes on M1.
∴x+y≤300.
x units of A and y units of B will take (2x+y) minutes on M2.∴2x+y≤360.
Let Z be the profit function. Then Z=5x+3y.
We have to maximize Z=5x+3y, subject to the constraints
x≥0,y≥0,x+y≤300and2x+y≤360.
Now, x+y=300⇒x300+y300=1.
This line meets the axes in (300,0)and(0,3000).
Joining these points, we get line x+y=300.
Since (0,0) satisfies the inequation x+y≤300, the region below the line x+y=300 containing O(0,0) represents x+y≤300.
Again, 2x+y=360⇒x180+y360=1.
This line meets the axis in (180,0)and(0,360).
Joining these points, we get the line 2x+y=360.
Since (0,0) satisfies 2x+y≤360, the region below the line 2x+y=360 containing (0,0) represents 2x+y≤360.
Also x=0 is the y-axis and y=0 is the x-axis.
On drawing these lines and shading the feasible region, we obtain a figure, given below.
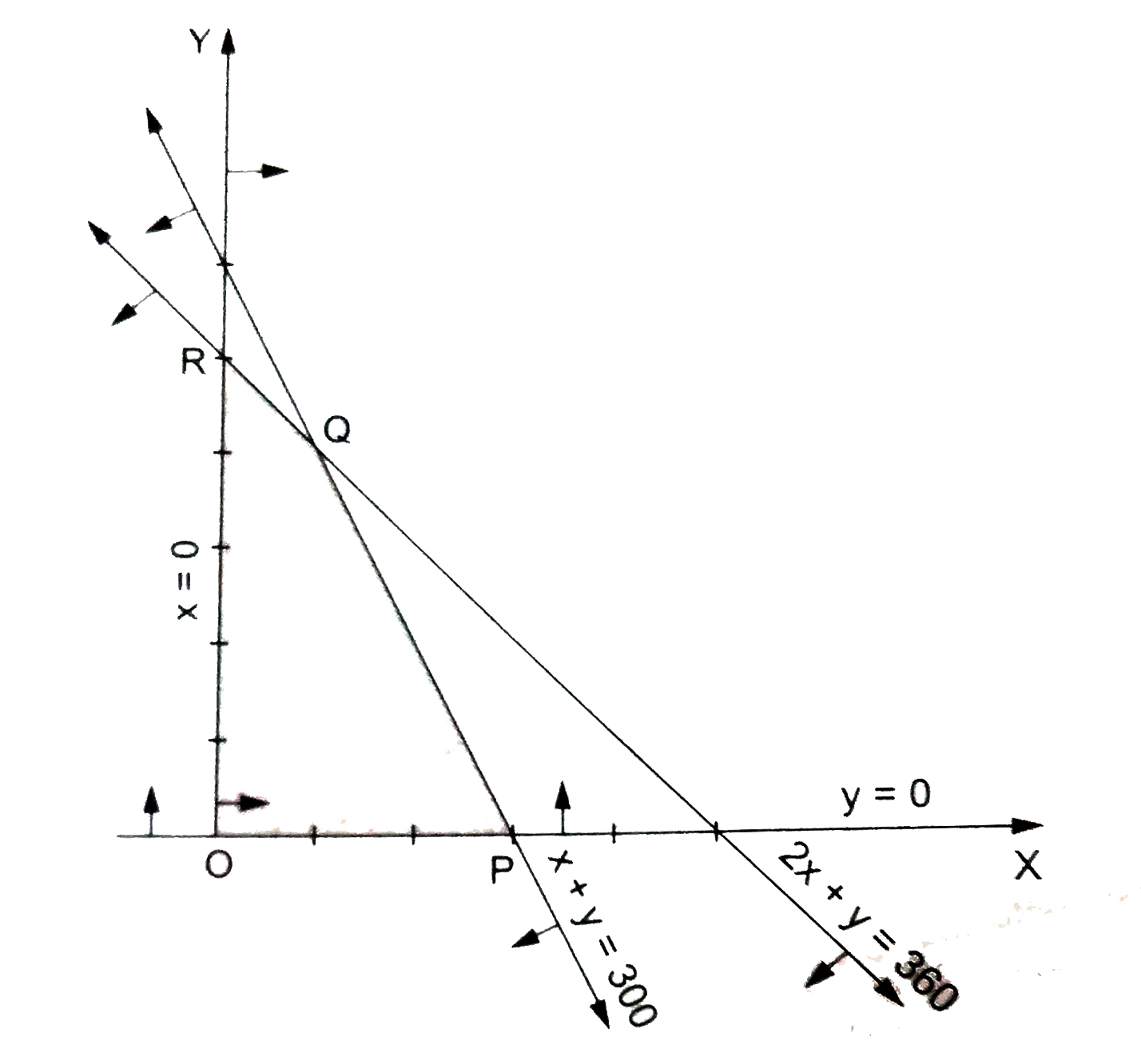
On solving x+y=300and2x+y=360, we get the point Q(60,240). ∴ the vertices of the feasible region are
O(0,0),P(180,0),Q(60,240)andR(0,300).
Values of Z=5x+3yatO,P,Q,R are 0,900,1020,900 respectively.
∴ Z is maximum when x=60andy=240.
Topper's Solved these Questions
- LINEAR PROGRAMMINGBOOK - RS AGGARWALCHAPTER - LINEAR PROGRAMMINGEXERCISE - Exprcise 33A
11 Videos
- LINEAR PROGRAMMINGBOOK - RS AGGARWALCHAPTER - LINEAR PROGRAMMINGEXERCISE - Exprcise 33B
38 Videos
- LINEAR DIFFERENTIAL EQUATIONS BOOK - RS AGGARWALCHAPTER - LINEAR DIFFERENTIAL EQUATIONS EXERCISE - Objective Questions
27 Videos
- MATRICESBOOK - RS AGGARWALCHAPTER - MATRICESEXERCISE - Exercise 5F
21 Videos
Similar Questions
A firm manufactures two types of products, A and B, and sells them at a profit of Rs.5 per unit of type A and Rs.3 per unit of type B.Each product is processed on two machines, M1andM2. One unit of type A requires one minute of processing time on M1 and one minute on M2. Machines M1andM2. are respectively available for at most 5 hours and 6 hours in a day. Find out how many units of each type of product the fiem should produce a day in order to maximize the profit. Solve the problem graphically.
View SolutionA firm manufactures two products A and B on which the profit earned per unit are Rs. 3 and Rs. 4 respectively. Each product is processed on two machines M1 and M2. Product A requires one minute of processing time on M1 and two minutes on M2 while B requires one minute on M1 and one minute on M2. Machine M1 is available for not more than 7 hours 30 minutes, while machine M2 is available for 10 hrs during any working day. Find the number of units of products A and B to manufactured to get maximum profit.
View Solution
Knowledge Check
A company produces three products. The products are processed on 3 different machines. The time required to manufacture one unit of each the three products and the daily capacity of three machines are given in the table below :

How many units of product 1 can be produced in one day?AA. 160BB. 205CC. 225DD. 64
Name two types of butter product on large scale.
View SolutionWhich type of respiration produces water as one of the products ?
View SolutionA company manufactures two types of novelty souvenirs made of plywood. Souvenirs of type A require 5 minutes each for cutting and 10 minutes each for assembling. Souvenirs of type B require 8 minutes each for cutting and 8 minutes each for assembling. There are 3 hours 20 minutes available for cutting and 4 hours for assembling. The profit is Rs 5 each for type A and Rs 6 each for type B souvenirs. How many souvenirs of each type should the company manufacture m order to maximise the profit?
View SolutionA company manufacturers two types of novelty suouvenirs made of plywood. Souvenirs of type A require 5 minutes each for cutting and 10 minutes each for assembling. Souvenirs of type B requires 8 minutes each for cutting and 8 minutes each for assembling. There are 3 hours 20 minutes available for cutting and 4 hours for assembling. The profit is Rs. 5 each for type A and Rs. 6 each for type B souvenirs. How many souvenirs of each type should the company manufacture in order to maximise the profit?
View SolutionA manufacturer makes two models A and B of a product. Each model is processed by two machines. To complete one unit of model. A, machines I and II must work 1 hours and 3 hours respectively. To complete one unit of model B, machine I and II must work 2 hours and 1 hour respectively. Machine I may not operate for more than 8 hours per day, and machine II for not more than 9 hours per day. If profits on model A and B per unit are Rs. 300 and Rs. 350, then how many units of each model should be produced , per day, to maximize the profits ?
View SolutionA company manufactures two types of toys A and B. Type A requires 5 minutes each for cutting and 10 minutes each for assembling. Type B requires 8 minutes each for cutting and 8 minutes each for assembling. There are 3 hours available for cutting and 4 hours available for assembling in a day. He earns a profit of Rs.50 each on type A and Rs.60 each on type. B. How many toys of each type should the company manufacture in a day to maximize the profit ?
View SolutionA copany manufactures two types of toys, A and B. Type A requires 5 minutes each for cutting and 10 minutes each for assembling. Type B requires 8 minutes each for cutting and 8 minutes each for assembling. There are 3 hours available for cutting and 4 hours available for assembling in a day. The profit is Rs.50 each on type A and Rs.60 each on type B. How many toys of each type should the company manufacture in a day to maximize the profit?
View Solution
