Question

A furniture dealer deals in only items - tables and chairs. He has $₹15,000$ to invest and a space to store atmost $60$ pieces. A table costs him $₹750$ and chair $₹150$ . Suppose he makes x tables any y chairs
The graphical solution of the inequations representing given data is

Options

A.
B.
C.
D. None of these

Answer

(c )

Explanation

To solve the problem step by step, we need to set up the inequalities based on the given information about the furniture dealer's constraints.

Step 1: Define Variables
Let:
- \( x \) = number of tables
- \( y \) = number of chairs

Step 2: Set Up the Inequalities
1. Investment Constraint: The dealer has ₹ 15,000 to invest. The cost of each table is ₹ 750 and each chair is ₹ 150. Therefore, the inequality representing the investment constraint is:
\( 750x + 150y \leq 15000 \)
Dividing the entire inequality by 150 to simplify:
\( 5x + y \leq 100 \)

2. Storage Constraint: The dealer can store at most 60 pieces of furniture (tables and chairs combined). Therefore, the inequality representing the storage constraint is:
\( x + y \leq 60 \)

3. Non-negativity Constraints: Since the dealer cannot produce a negative number of tables or chairs, we have:
\( x \geq 0 \quad \text{and} \quad y \geq 0 \)

Step 3: Graph the Inequalities
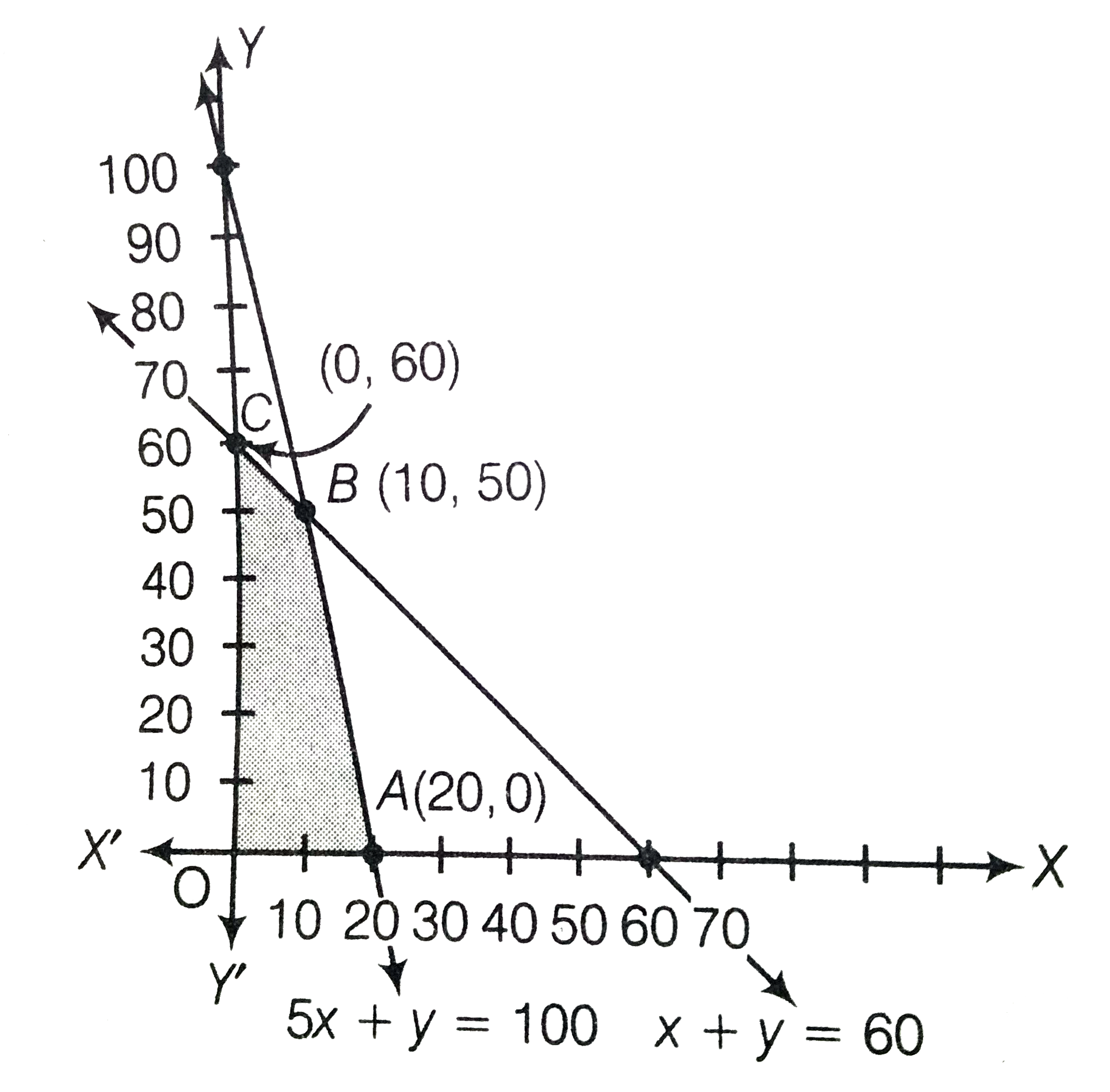
To graph the inequalities, we need to find the intercepts and plot the lines.

1. For the first inequality \( 5x + y = 100 \):
- When \( x = 0 \):
\( y = 100 \quad \text{(Point: (0, 100))} \)
- When \( y = 0 \):
\( 5x = 100 \implies x = 20 \quad \text{(Point: (20, 0))} \)

2. For the second inequality \( x + y = 60 \):
- When \( x = 0 \):
\( y = 60 \quad \text{(Point: (0, 60))} \)
- When \( y = 0 \):
\( x = 60 \quad \text{(Point: (60, 0))} \)

Step 4: Identify the Feasible Region
The feasible region is where all the inequalities overlap. This region is bounded by the lines \( 5x + y = 100 \) and \( x + y = 60 \), and it must also satisfy \( x \geq 0 \) and \( y \geq 0 \).

Step 5: Find the Intersection Point
To find the intersection of the lines \( 5x + y = 100 \) and \( x + y = 60 \), we can solve these equations simultaneously.

1. From \( x + y = 60 \), we can express \( y \):
\( y = 60 - x \)

2. Substitute \( y \) into the first equation:
\( 5x + (60 - x) = 100 \)
Simplifying:
\( 5x + 60 - x = 100 \implies 4x + 60 = 100 \implies 4x = 40 \implies x = 10 \)

3. Substitute \( x = 10 \) back into \( y = 60 - x \):
\( y = 60 - 10 = 50 \)

Step 6: Conclusion
Thus, the solution to the problem is:
- Number of tables \( x = 10 \)
- Number of chairs \( y = 50 \)

Summary
The graphical solution shows that the dealer can purchase a maximum of 10 tables and 50 chairs while adhering to the constraints of investment and storage.