A horse is placed for grazing inside a rectangular field 70m by 52m and is tethered to one corner by a rope 21m long. On how much area can it graze?
Given: rectangular field 70 m by 52 m and rope 21 m long
Area of grass grazed by horse =θ3600× πr2
[# X_RD_15_E01_35]
=90∘360∘×227× 21× 21
=6932=346.5cm2
Topper's Solved these Questions
Similar Questions
A horse is placed for grazing inside a rectangular field 70 m by 52 m and is tethered to one corner by a rope 21 m long. On how much area can it graze?
View SolutionA horse is placed for grazing inside a rectangular field 40 m by 36 m and is tethered to one corner by a rope 14 m long. Over how much area can it graze? (Take π=22/7) .
View Solution
Knowledge Check
A horse is tethered to one corner of a rectangular grassy field 40 m by 24 m with a rope 14 m long. Over how much area of the field can it graze?
A154 m2B308 m2C150 m2DNone of theseAt each corner of a triangular field of sides 26m, 28m and 30m, a cow is tethered by a rope of length 7m. The area (in m2) ungrazed by the cows is
A336B259C154D77One side of a rectangular field is 9 m and one of its diagonal is 20 m. Find the area of the field.
A9√319sqmB7√319sqmC2√319sqmD5√319sqm
A horse is tethered to one corner of a rectangular field, 60 m by 40 m, by a rope 14 m long. On how much area can the horse graze?
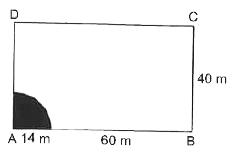
View SolutionA horse is tethered to one corner of a rectangular field of dimensions 70 m × 52 m, by a rope of length 21 m. How much area of the field can it graze?
View SolutionA horse is tethered to a corner of a rectangular field 80 m by 50 m by a rope of length 7 m . Find the area over which it can graze . Also find the area beyond his reach .
View SolutionOne side of a rectangular field is 15 m and one of its diagonals is 17 m. Find the area of the field.
View SolutionA horse is tied at the corner of a rectangular field whose length is 20 m and width is 16 m, with a rope whose length is 14 m. Find the area which the horse can graze: (a) 144 sq. m (b) 154 sq. m (c) 156 sq. m (d) 164 sq. m
View Solution
