A horse is tethered to one corner of a rectangular field of dimensions 70 m × 52 m, by a rope of length 21 m. How much area of the field can it graze?
The correct Answer is:346.5m2
To solve the problem of how much area a horse can graze when tethered to one corner of a rectangular field, we can follow these steps:
1. Understand the Problem:
The horse is tethered at one corner of a rectangular field with dimensions 70 m by 52 m, and the length of the rope is 21 m. The area that the horse can graze will be a quarter of a circle (a quadrant) with a radius equal to the length of the rope.
2. Identify the Radius:
The radius r of the grazing area is equal to the length of the rope, which is 21 m.
3. Calculate the Area of the Quadrant:
The area A of a full circle is given by the formula:
A=πr2
Since the horse can only graze in a quarter of the circle (the quadrant), we will take one-fourth of the area of the full circle:
Area of the quadrant=14πr2
4. Substituting the Values:
Substitute r=21 m into the formula:
Area of the quadrant=14π(21)2
5. Calculating 212:
212=441
So,
Area of the quadrant=14π×441
6. Using π≈227:
Area of the quadrant=14×227×441
7. Calculating the Area:
First, calculate 4414:
4414=110.25
Now, multiply by 227:
Area=110.25×227
=110.25×3.142857≈346.5 m2
8. Final Answer:
The area that the horse can graze is approximately 346.5 m2.
Summary:
The area grazed by the horse is 346.5 m2.
The horse is tethered at one corner of a rectangular field with dimensions 70 m by 52 m, and the length of the rope is 21 m. The area that the horse can graze will be a quarter of a circle (a quadrant) with a radius equal to the length of the rope.
The radius r of the grazing area is equal to the length of the rope, which is 21 m.
The area A of a full circle is given by the formula:
A=πr2
Since the horse can only graze in a quarter of the circle (the quadrant), we will take one-fourth of the area of the full circle:
Area of the quadrant=14πr2
Substitute r=21 m into the formula:
Area of the quadrant=14π(21)2
212=441
So,
Area of the quadrant=14π×441
Area of the quadrant=14×227×441
First, calculate 4414:
4414=110.25
Now, multiply by 227:
Area=110.25×227
=110.25×3.142857≈346.5 m2
The area that the horse can graze is approximately 346.5 m2.
The area grazed by the horse is 346.5 m2.
Topper's Solved these Questions
- C.B.S.E 2020 CLASS -X (OUTSIDE DELHI)BOOK - OSWAL PUBLICATIONCHAPTER - C.B.S.E 2020 CLASS -X (OUTSIDE DELHI)EXERCISE - OUTSIDE DELHI SET -III ( SECTION- D )
2 Videos
- C.B.S.E 2020 CLASS -X (OUTSIDE DELHI)BOOK - OSWAL PUBLICATIONCHAPTER - C.B.S.E 2020 CLASS -X (OUTSIDE DELHI)EXERCISE - OUTSIDE DELHI SET -III ( SECTION- B )
2 Videos
- C.B.S.E 2020 CLASS -X (DELHI)BOOK - OSWAL PUBLICATIONCHAPTER - C.B.S.E 2020 CLASS -X (DELHI)EXERCISE - DELHI SET -III ( SECTION- D )
1 Videos
- CIRCLES BOOK - OSWAL PUBLICATIONCHAPTER - CIRCLES EXERCISE - SELF ASSESSMENT
5 Videos
Similar Questions
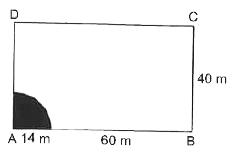
A horse is tethered to one corner of a rectangular field, 60 m by 40 m, by a rope 14 m long. On how much area can the horse graze?
View SolutionA horse is tethered to a corner of a rectangular field 80 m by 50 m by a rope of length 7 m . Find the area over which it can graze . Also find the area beyond his reach .
View Solution
Knowledge Check
A horse is tethered to one corner of a rectangular grassy field 40 m by 24 m with a rope 14 m long. Over how much area of the field can it graze?
A154 m2B308 m2C150 m2DNone of theseA goat is tied to one corner of a field of dimensions 16 m × 10m with a rope 7 m long. Find the area of the field that the goat can graze.
The following are the steps involved in solving the above problem. Arrange them in sequential order.
(A) Required area = 38.5 m2
(B) Area of the field that the goat can graze = Area of the sector of radius 7 m and a sector angle of 90∘
(C ) 90∘360×227×(7)2AABCBBCACBACDCBAA cow is tied on the corner of a rectangular field of size 30m×20m by a 14m long rope. The area of the region, that she can graze is (use π=227) :
A350m2B196m2C154m2D22m2
A cow is tied with a rope of length 14m at the corner of a rectangular field of dimensions 20m×16m . Find the area of the field in which the cow can graze.
View SolutionA horse is tied at the corner of a rectangular field whose length is 20 m and width is 16 m, with a rope whose length is 14 m. Find the area which the horse can graze: (a) 144 sq. m (b) 154 sq. m (c) 156 sq. m (d) 164 sq. m
View SolutionA horse is placed for grazing inside a rectangular field 40 m by 36 m and is tethered to one corner by a rope 14 m long. Over how much area can it graze? (Take π=22/7) .
View SolutionA horse is placed for grazing inside a rectangular field 70 m by 52 m and is tethered to one corner by a rope 21 m long. On how much area can it graze?
View SolutionAt each corner of a triangular field of sides 26 m, 28 m and 30 m, a cow is tethered by a rope of, length 7 m. The area (in m2) ungrazed by the cows is
View Solution
