A ladder has rungs 25 cm apart, (see Figure). The rungs decrease uniformly in length from 45 cm at the bottom to 25 cm at the top. If the top and the bottom rungs are 212m apart, what is the length of the wood required for the rungs?
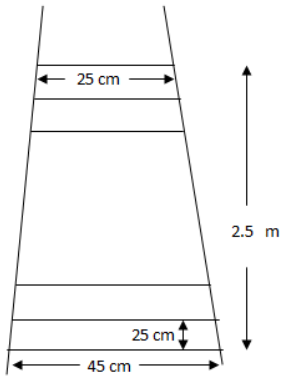
Length of rugs is in AP
n=250/25=10
therefor, number of rugs=10
S=r1+r2+...+r10
S10=102(a+l)
S10=5(45+25)
S10=350cm
Topper's Solved these Questions
- BOOK - NCERTCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - Exercise 5.14videos
ARITHMETIC PROGRESSIONS
- BOOK - NCERTCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - Exercise 5.320videos
ARITHMETIC PROGRESSIONS
- BOOK - NCERTCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - Solved Examples16videos
ARITHMETIC PROGRESSIONS
- BOOK - NCERTCHAPTER - AREAS RELATED TO CIRCLESEXERCISE - SOLVED EXAMPLES6videos
AREAS RELATED TO CIRCLES
- BOOK - NCERTCHAPTER - CIRCLESEXERCISE - SOLVED EXAMPLES3videos
CIRCLES
Similar Questions
Knowledge Check
- Question 1 - Select One
The length of the common chord of two circles of radii 15 and 20, whose centres are 25 units apart, is
A24B25C15D20 - Question 2 - Select One
Two sides of a triangle are of length 4 cm and 2.5 cm. The length of the third side of the triangle cannot be
A6 cmB6.5 cmC5.5 cmD6.3 cm
A washing tub in the shape of a frustum of a cone has height 21 cm. The radii of the circular top and bottom are 20 cm and 15 cm respectively. What is the capacity of the tub in litres?
View SolutionA bucket is of height 25 cm. Its top and bottom radii are 20 cm and 10 cm respectively. Its capacity in litres is
View SolutionA block of wood has a mass of 25g. When a 5g metal piece with a volume of 2cm3 is attached to the bottom of the block, the wood barely floats in water. What is the volume V of the wood?
View SolutionA ladder is place against a wall such that its foot is 2.5 m distance from the wall, and its top reaches a window 6m above the ground. Find the length of the ladder.
View SolutionThe area of a rectangular sheet is 750 cm2. If the length of the sheet is 25 cm, then what is its width ?
View SolutionFigure shows an iron ball suspended by thread of negligible mass from an upright cylinder that floats partially submerged in water. The cylinder has a height of 6.00 cm, a face area of 12.0cm2 on the top and bottom, and a density of 0.25g/cm2 , and 1.00 cm of its height is above the water surface. What is the radius of the iron ball?
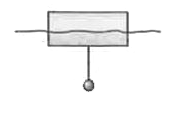 View Solution
View Solution

