A lending library has a fixed charge for the first three days and an additional charge for each day thereafter. Aarushi paid Rs. 27 for a book kept for seven days. If fixed charges are Rs. x and per day charges are Rs. y, then which of the following linear equations represents the given information?
4x + y = 27
x + 4y = 27
x + 7y = 27
x + y + 7 = 27
The correct Answer is:B
To solve the problem, we need to derive a linear equation based on the information given about the charges in the library. Let's break it down step by step.
Step 1: Understand the Charges
Aarushi paid a total of Rs. 27 for keeping a book for 7 days. The charges consist of:
- A fixed charge for the first 3 days.
- An additional charge for each day after the first 3 days.
Step 2: Define Variables
Let:
- x = Fixed charge for the first 3 days.
- y = Charge per day for each day after the first 3 days.
Step 3: Calculate the Number of Days Charged
Aarushi kept the book for 7 days. The first 3 days are covered by the fixed charge, so the additional days charged will be:
- Total days kept = 7
- Days covered by fixed charge = 3
- Additional days = 7−3=4
Step 4: Write the Total Charge Equation
The total charge paid by Aarushi can be expressed as:
Total Charge=Fixed Charge+Charge for Additional Days
Substituting the values, we have:
27=x+(4⋅y)
This simplifies to:
x+4y=27
Conclusion
The linear equation that represents the given information is:
x+4y=27
Aarushi paid a total of Rs. 27 for keeping a book for 7 days. The charges consist of:
- A fixed charge for the first 3 days.
- An additional charge for each day after the first 3 days.
Let:
- x = Fixed charge for the first 3 days.
- y = Charge per day for each day after the first 3 days.
Aarushi kept the book for 7 days. The first 3 days are covered by the fixed charge, so the additional days charged will be:
- Total days kept = 7
- Days covered by fixed charge = 3
- Additional days = 7−3=4
The total charge paid by Aarushi can be expressed as:
Total Charge=Fixed Charge+Charge for Additional Days
Substituting the values, we have:
27=x+(4⋅y)
This simplifies to:
x+4y=27
The linear equation that represents the given information is:
x+4y=27
Topper's Solved these Questions
- IMO QUESTION PAPER SET A 2019BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - IMO QUESTION PAPER SET A 2019EXERCISE - Achievers Section
5 Videos
- IMO QUESTION PAPER SET A 2019BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - IMO QUESTION PAPER SET A 2019EXERCISE - Achievers Section
5 Videos
- IMO QUESTION PAPER SET A 2017BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - IMO QUESTION PAPER SET A 2017EXERCISE - ARCHIEVERS SECTION
5 Videos
- IMO QUESTION PAPER SET B 2019BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - IMO QUESTION PAPER SET B 2019EXERCISE - ACHIEVERS SECTION
5 Videos
Similar Questions
A lending library has a fixed charge for the first three days and an additional chargefor each day thereafter. Aarushi paid Rs 27 for a book kept for seven days. If fixed charges are Rs x and per day charges are Rs y. Write the linear equation representing the abvoe information.
View SolutionA lending library has a fixed charge for the first three days and an additional charge for each day thereafter. Saritha paid Rs 27 for a book kept for seven days, while Susy paid Rs 21 for the book she kept for five days. Find the fixed charge and the charge for each extra day.
View SolutionReading book in a library has a fixed charge for the first three days and an additional charge for each day thereafter. Shristi paid ₹ 27 for a book kept for seven days. While Bunty paid ₹ 21 for the book kept for five days.
(i) Find the fixed charge.
(ii) Find how much additional charge Shristi and Bunty paid.
(iii) Which mathematical concept is used in this problem ?
(iv) Which value does it depict ?
View SolutionA shopkeeper gives books on rent for reading. She takes a fixed charge for the first two days and an additional charge for each day thereafter. Latika paid Rs. 22 for a book kept for six days, while Anand paid Rs. 16 for the book kept for four days. Find the fixed charges and the charge for each extra day.
View Solution
A book store shopkeeper gives books on rent for reading. He has variety of books in his store related to fiction, story books, quiz books etc. He takes a fixed charges for the first two days and an additional charges for each day thereafter. Radhika paid Rs 22 for a book and kept for six days, while Reshma paid Rs 16 when she kept for 4 days. Let the fixed charges be represented by Rs x and charges for each days be represented by Rs y.
Represent algebraically the situation of amount paid by Radhika.
View Solution
A book store shopkeeper gives books on rent for reading. He has variety of books in his store related to fiction, story books, quiz books etc. He takes a fixed charges for the first two days and an additional charges for each day thereafter. Radhika paid Rs 22 for a book and kept for six days, while Reshma paid Rs 16 when she kept for 4 days. Let the fixed charges be represented by Rs x and charges for each days be represented by Rs y.
Represent algebraically the situation of amount paid by Reshma
View SolutionJane has Rs. X with her. The amount with Colin is Rs. 15, which Rs. 2 more than that with Jane.
Which of the following equations correctly represents the given information?
View SolutionIt is common that Governments revise travel fares from time to time based on various factors such as inflation ( a general increase in prices and fall in the purchasing value of money) on different types of vehicles like auto, Rickshaws, taxis, Radio cab etc. The auto charges in a city comprise of a fixed charge together with the charge for the distance covered. Study the following situations
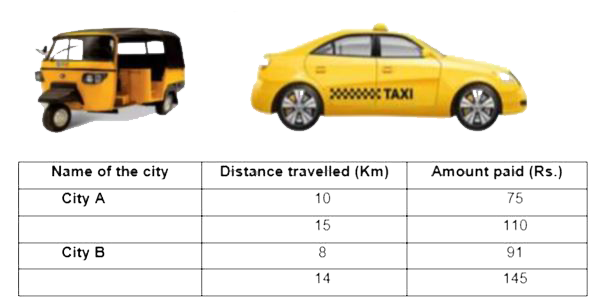
Situation 1: In city A, for a journey of 10 km, the charge paid is Rs 75 and for a journey of 15 km, the charge paid is Rs 110.
Situation 2: In a city B, for a journey of 8km, the charge paid is Rs91 and for a journey of 14km, the charge paid is Rs 145.
If the fixed charges of auto rickshaw be Rs x and the running charges be Rs y km/hr, the pair of linear equations representing the situation is
View SolutionA certain amount is charged for 1 km for a scooter fare and lesser amount for each additional km. If one day Aliya paid Rs. 24 for 5 km and on another day she paid Rs. 35.40 for 8 km. Find the fare for the 1st km and for the additional kms.
View SolutionThe parking charges of a car in a private parking is Rs 20 for the first hour and Rs 10 for subsequent hours.Taking total parking charges to be y & total parking time as x hours form a linear equation.Write it in standard form.
View Solution
