A line L:y=mx+3 meets y-axis at E(0,3) and the arc of the parabola y 2 =16x,0≤y≤6 at the point F(x 0 ,y 0 ). The tangent to the parabola at F(x 0 ,y 0 ) intersects the y-axis at G(0,y 1 ). The slope m of the line L is chosen such that the area of the △EFG has a local maximum. Match List 1 with List 2 List 1 List 2 A. m= 1. 2 1 B. Maximum area of ΔEFG is 2. 4 C. y 0 = 3. 2 D. y 1 = 4. 1Solution in Tamil
The correct Answer is:a→s;b→p;c→q;d→r
a→s,b→p,c→q,d→r
Let point F on the parabola be (4t2,8t).
Tangent at this point is ty=x+4t2
It meets the y-axis at (0,4t).
Then the area of triangle
EFG is A(t)=2t2(3−4t)=6t−8t3
Differentiating w.r.t, we get
A'(t)=12t−24t2
For A'=0, t=1/2, which is a maxima. So, point F is (1,4).
slope of EF = 1
∴ m=1orA(t)∣max.=12 sq. units
y0=4
andy1=2
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - PARABOLAEXERCISE - Single Correct Answer Type46videos
PARABOLA
- BOOK - CENGAGECHAPTER - PARABOLAEXERCISE - Multiple Correct Answers Type10videos
PARABOLA
- BOOK - CENGAGECHAPTER - PARABOLAEXERCISE - JEE Main Previous Year8videos
PARABOLA
- BOOK - CENGAGECHAPTER - PAIR OF STRAIGHT LINESEXERCISE - Exercise (Numerical)5videos
PAIR OF STRAIGHT LINES
- BOOK - CENGAGECHAPTER - PERMUTATION AND COMBINATIONEXERCISE - Question Bank4videos
PERMUTATION AND COMBINATION
Similar Questions
The equation x2−2xy+y2+3x+2=0 represents
03:45View SolutionA family of curve S is given by S≡x2+2xy+y2−4x(1−λ)−4y(1+λ)+4, then S=0 represents
06:51View SolutionIf the two tangents drawn from a point P to the parabola y2=4x are at right angles then the locus of P is
05:32View SolutionThe distance between the lines (x+7y)2+4√2(x+7y)−42=0 is_____________
04:45View SolutionIf the number of ways in which a selection of 100 balls can be made out of 100 identical red balls, 100 identical blue balls and 100 identical white balls is
04:23View SolutionFind the value of m for which the lines mx+(2m+3)y+m+6=0 and mx+(2m+1)x+(m−6)y+9=0 intersect at a; point on y-axis .
02:53View SolutionA line L : y = mx + 3 meets y-axis at E (0, 3) and the arc of the parabola y^2 = 16x 0leyle6 at the point art F(x_0,y_0). The tangent to the parabola at F(X_0,Y_0) intersects the y-axis at G(0,y). The slope m of the line L is chosen such that the area of the triangle EFG has a local maximum P) m= Q) = Maximum area of triangle EFG is (R) y_0= (S) y_1=
04:42View SolutionStatement 1: The line x-2y=2 meets the parabola,y^(2)+2x=0 only at the points (-2,2) statement 2: The line y=mx-(1)/(2m)(m!=0) is tangent to the parabola,y^(2)=-2x at the point (-(1)/(2m^(2)),-(1)/(m))
07:49View SolutionFind the equation of the parabola whose focus is at (0, 0) and vertex is at the intersection of the line x+y=1andx−y=3.
04:41View Solutionयदि रेखा x+y=1 परवलय y2−y+x=0 को स्पर्श करती है, तो स्पर्श बिंदु के निर्देशांक होंगे
10:33View SolutionArea bounded by parabola x2=y, the Y-axis and the lines y = 0, y = 4 is
View Solutionm का मान निकालें ताकि निम्नलिखित रेखाएँ y-अक्ष पर एक बिंदु पर प्रतिच्छेद करें।
mx+(2m+3)y+m+6=0 तथा (2m+1)x+(m−1)y+(m−9)=007:11View Solutionline L:y=mx+3 meets y–axis at E(0, 3) and the are of the parabola y2=16x,0≤y≤6 at the point F(x0,y0). The tangent to the parabola at F(x0,y0) intersects the y-axis at G(0,y1). The slope m of the L is chosen such that the area of the triangle EFG has a local maximum.
Match List I with List II and select the correct answer using the code given below the lists :
06:29View SolutionIf the line x+y=1 is a tangent to the parabola y2−y+x=0, then the point of contact is-
05:23View Solutionएक रेखा L:y=mx+3,y अक्ष के बिन्दु E(0, 3) तथा परवलय के चाप y2=16x,0≤y≤6 के बिन्दु F(x0,y0) पर मिलती है। परवलय की बिन्दु F(x0,y0) पर स्पर्शी -अक्ष को बिन्दु G(0,y1) पर काटती है रेखा L की प्रवणता m ऐसी चुनी जाती है कि △EFG के क्षेत्रफल का एक स्थानीय अधिकतम है।
सूची I सूची II से सुमेलित कीजिए तथा सूचियों के नीचे दिए गए कोड का प्रयोग करके सही उत्तर चुनिए।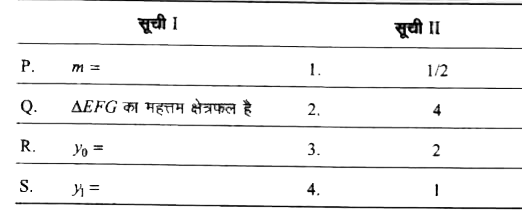
04:30View SolutionThe angle between tangents to the parabola y2=4x at the points where it intersects with the line x-y -1 =0 is
02:56View Solution