Question

A man observes a car from the top of a tower, which is moving towards the tower with a uniform speed. If the angle of depression of the car change from ${30}^{\circ }\text{and}{45}^{\circ }$ in 12 minutes, find the time taken by the car now toreach the tower.

Explanation

To solve the problem step by step, we will use trigonometric principles and the information given in the question.

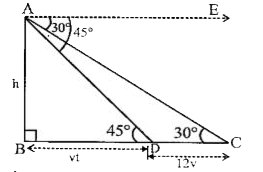
1. Understanding the Problem:
- We have a tower and a car moving towards it.
- The angle of depression changes from \(30^\circ\) to \(45^\circ\) in 12 minutes.

2. Setting Up the Diagram:
- Let the height of the tower be \(h\).
- Let the distance of the car from the base of the tower when the angle of depression is \(30^\circ\) be \(x + y\).
- Let the distance of the car from the base of the tower when the angle of depression is \(45^\circ\) be \(y\).

3. Using Trigonometric Ratios:
- From the triangle formed when the angle of depression is \(45^\circ\):
\( \tan(45^\circ) = \frac{h}{y} \implies h = y \quad \text{(since } \tan(45^\circ) = 1\text{)} \)
- From the triangle formed when the angle of depression is \(30^\circ\):
\( \tan(30^\circ) = \frac{h}{x + y} \implies \frac{1}{\sqrt{3}} = \frac{h}{x + y} \)
Substituting \(h = y\) into this equation:
\( \frac{1}{\sqrt{3}} = \frac{y}{x + y} \)

4. Cross-Multiplying:
- Cross-multiplying gives:
\( x + y = \sqrt{3}y \)
- Rearranging this gives:
\( x = \sqrt{3}y - y = (\sqrt{3} - 1)y \)

5. Finding the Time Taken by the Car:
- The car covers the distance \(x\) in 12 minutes. Therefore, the speed of the car can be expressed as:
\( \text{Speed} = \frac{x}{12} = \frac{(\sqrt{3} - 1)y}{12} \)
- Now, we need to find the time taken to cover the distance \(y\):
\( \text{Time} = \frac{y}{\text{Speed}} = \frac{y}{\frac{(\sqrt{3} - 1)y}{12}} = \frac{12}{\sqrt{3} - 1} \)

6. Rationalizing the Denominator:
- To simplify \(\frac{12}{\sqrt{3} - 1}\), we multiply the numerator and denominator by the conjugate \((\sqrt{3} + 1)\):
\( \frac{12(\sqrt{3} + 1)}{(\sqrt{3} - 1)(\sqrt{3} + 1)} = \frac{12(\sqrt{3} + 1)}{3 - 1} = \frac{12(\sqrt{3} + 1)}{2} = 6(\sqrt{3} + 1) \)

7. Calculating the Final Time:
- The time taken by the car to cover distance \(y\) is:
\( 6(\sqrt{3} + 1) \text{ minutes} \)
- Approximating \(\sqrt{3} \approx 1.732\):
\( 6(1.732 + 1) \approx 6(2.732) \approx 16.392 \text{ minutes} \)

8. Conclusion:
- The total time taken by the car to reach the tower from the point where the angle of depression was \(45^\circ\) is approximately \(16.39\) minutes.