Question

A man on the top of a vertical tower observes a car moving at a uniform
speed coming directly towards it. If it takes 12 minutes for the angle of
depression to change from ${30}^0\to {45}^0,$
how soon after this will the car reach the tower? Give your answer to
the nearest second.

Explanation

To solve the problem step by step, we can follow these steps:

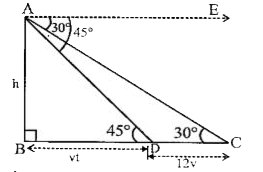
Step 1: Understand the Problem
We have a vertical tower of height \( H \). A man at the top observes a car moving towards the tower. The angle of depression changes from \( 30^\circ \) to \( 45^\circ \) in 12 minutes.

Step 2: Set Up the Geometry
- Let \( A \) be the position of the car when the angle of depression is \( 30^\circ \).
- Let \( B \) be the position of the car when the angle of depression is \( 45^\circ \).
- Let \( C \) be the base of the tower.

Step 3: Use Trigonometry to Find Distances
Using the angles of depression:
1. From point \( A \) (angle \( 30^\circ \)):
\( \tan(30^\circ) = \frac{H}{AC} \implies AC = \frac{H}{\tan(30^\circ)} = H \sqrt{3} \)

2. From point \( B \) (angle \( 45^\circ \)):
\( \tan(45^\circ) = \frac{H}{BC} \implies BC = H \)

Step 4: Calculate the Distance \( AB \)
The distance \( AB \) (the distance the car travels from \( A \) to \( B \)):
\( AB = AC - BC = H\sqrt{3} - H = H(\sqrt{3} - 1) \)

Step 5: Calculate the Speed of the Car
The car takes 12 minutes to travel from \( A \) to \( B \). Converting 12 minutes to seconds:
\( 12 \text{ minutes} = 12 \times 60 = 720 \text{ seconds} \)

Let the speed of the car be \( V \) (in meters per second). Then:
\( AB = V \times 720 \)
Thus,
\( H(\sqrt{3} - 1) = V \times 720 \)

Step 6: Find the Time to Travel from \( B \) to \( C \)
Now, we need to find the time \( T \) it takes for the car to travel from \( B \) to \( C \):
\( BC = H \)
Using the speed \( V \):
\( T = \frac{BC}{V} = \frac{H}{V} \)

Step 7: Substitute \( H \) in Terms of \( V \)
From the earlier equation:
\( H = \frac{V \times 720}{\sqrt{3} - 1} \)
Substituting this into the time equation:
\( T = \frac{\frac{V \times 720}{\sqrt{3} - 1}}{V} = \frac{720}{\sqrt{3} - 1} \)

Step 8: Calculate \( T \)
Now we can compute \( T \):
\( T = \frac{720}{\sqrt{3} - 1} \)
Calculating \( \sqrt{3} \approx 1.732 \):
\( T \approx \frac{720}{1.732 - 1} = \frac{720}{0.732} \approx 983.60 \text{ seconds} \)

Step 9: Final Answer
Thus, the time taken for the car to reach the tower after the angle of depression changes from \( 30^\circ \) to \( 45^\circ \) is approximately 984 seconds (to the nearest second).

---