A psychologist selected a random sample of 20 students. He grouped them in 10 pairs so that the students in each pair have nearly equal scores in an intelligence test. In each pair, one student was taught by method A and the other by method B and examined after the course. The marks obtained by them after the course are as follows.
PairsMethod AMethod B134382364332839434455404363050734528283993240104242
Calculate spearman's Rank correlation
To calculate Spearman's Rank Correlation Coefficient for the given data, we will follow these steps:
Step 1: Organize the Data
We have the following pairs of scores for Method A and Method B:
| Pairs | Method A | Method B |
|-------|----------|----------|
| 1 | 34 | 38 |
| 2 | 36 | 43 |
| 3 | 28 | 39 |
| 4 | 34 | 45 |
| 5 | 40 | 43 |
| 6 | 30 | 50 |
| 7 | 34 | 52 |
| 8 | 28 | 39 |
| 9 | 32 | 40 |
| 10 | 42 | 42 |
Step 2: Assign Ranks to Method A and Method B
- Rank Method A:
- 42 → 1
- 40 → 2
- 36 → 3
- 34 (average of ranks 4, 5, 6) → 4.5
- 34 (already assigned) → 4.5
- 34 (already assigned) → 4.5
- 32 → 7
- 30 → 8
- 28 (average of ranks 9, 10) → 9.5
- 28 (already assigned) → 9.5
| Method A | Rank A |
|----------|--------|
| 34 | 4.5 |
| 36 | 3 |
| 28 | 9.5 |
| 34 | 4.5 |
| 40 | 2 |
| 30 | 8 |
| 34 | 4.5 |
| 28 | 9.5 |
| 32 | 7 |
| 42 | 1 |
- Rank Method B:
- 52 → 1
- 50 → 2
- 45 → 3
- 43 (average of ranks 4, 5) → 4.5
- 43 (already assigned) → 4.5
- 39 (average of ranks 6, 7) → 6.5
- 39 (already assigned) → 6.5
- 40 → 8
- 38 → 9
- 42 → 10
| Method B | Rank B |
|----------|--------|
| 38 | 9 |
| 43 | 4.5 |
| 39 | 6.5 |
| 45 | 3 |
| 43 | 4.5 |
| 50 | 2 |
| 52 | 1 |
| 39 | 6.5 |
| 40 | 8 |
| 42 | 10 |
Step 3: Calculate the Differences (D) and D²
Now, we calculate the difference D=Rank A−Rank B and D2.
| Rank A | Rank B | D | D² |
|--------|--------|--------|--------|
| 4.5 | 9 | -4.5 | 20.25 |
| 3 | 4.5 | -1.5 | 2.25 |
| 9.5 | 6.5 | 3 | 9 |
| 4.5 | 3 | 1.5 | 2.25 |
| 2 | 4.5 | -2.5 | 6.25 |
| 8 | 2 | 6 | 36 |
| 4.5 | 1 | 3.5 | 12.25 |
| 9.5 | 6.5 | 3 | 9 |
| 7 | 8 | -1 | 1 |
| 1 | 10 | -9 | 81 |
Step 4: Sum of D²
Now we sum up D²:
∑D²=20.25+2.25+9+2.25+6.25+36+12.25+9+1+81=179.5
Step 5: Apply the Spearman’s Rank Correlation Coefficient Formula
The formula for Spearman's Rank Correlation Coefficient r is:
r=1−6∑D2n(n2−1)
Where n is the number of pairs (in this case, n=10).
Substituting the values:
r=1−6×179.510(102−1)=1−1077990=1−1.09=−0.09
Final Answer
The Spearman's Rank Correlation Coefficient r is approximately -0.09.
We have the following pairs of scores for Method A and Method B:
|-------|----------|----------|
| 1 | 34 | 38 |
| 2 | 36 | 43 |
| 3 | 28 | 39 |
| 4 | 34 | 45 |
| 5 | 40 | 43 |
| 6 | 30 | 50 |
| 7 | 34 | 52 |
| 8 | 28 | 39 |
| 9 | 32 | 40 |
| 10 | 42 | 42 |
- Rank Method A:
- 42 → 1
- 40 → 2
- 36 → 3
- 34 (average of ranks 4, 5, 6) → 4.5
- 34 (already assigned) → 4.5
- 34 (already assigned) → 4.5
- 32 → 7
- 30 → 8
- 28 (average of ranks 9, 10) → 9.5
- 28 (already assigned) → 9.5
|----------|--------|
| 34 | 4.5 |
| 36 | 3 |
| 28 | 9.5 |
| 34 | 4.5 |
| 40 | 2 |
| 30 | 8 |
| 34 | 4.5 |
| 28 | 9.5 |
| 32 | 7 |
| 42 | 1 |
- 52 → 1
- 50 → 2
- 45 → 3
- 43 (average of ranks 4, 5) → 4.5
- 43 (already assigned) → 4.5
- 39 (average of ranks 6, 7) → 6.5
- 39 (already assigned) → 6.5
- 40 → 8
- 38 → 9
- 42 → 10
|----------|--------|
| 38 | 9 |
| 43 | 4.5 |
| 39 | 6.5 |
| 45 | 3 |
| 43 | 4.5 |
| 50 | 2 |
| 52 | 1 |
| 39 | 6.5 |
| 40 | 8 |
| 42 | 10 |
Now, we calculate the difference D=Rank A−Rank B and D2.
|--------|--------|--------|--------|
| 4.5 | 9 | -4.5 | 20.25 |
| 3 | 4.5 | -1.5 | 2.25 |
| 9.5 | 6.5 | 3 | 9 |
| 4.5 | 3 | 1.5 | 2.25 |
| 2 | 4.5 | -2.5 | 6.25 |
| 8 | 2 | 6 | 36 |
| 4.5 | 1 | 3.5 | 12.25 |
| 9.5 | 6.5 | 3 | 9 |
| 7 | 8 | -1 | 1 |
| 1 | 10 | -9 | 81 |
Now we sum up D²:
The formula for Spearman's Rank Correlation Coefficient r is:
The Spearman's Rank Correlation Coefficient r is approximately -0.09.
Topper's Solved these Questions
- SELF ASSESSMENT PAPER 2 BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 2 EXERCISE - SECTION B QUESTIONS
10 Videos
- SELF ASSESSMENT PAPER 1BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 1EXERCISE - SECTION C
10 Videos
- SELF ASSESSMENT PAPER 3BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 3EXERCISE - SECTION C
10 Videos
Similar Questions
The eccentricity of the ellipse x236+y225=1 is
View SolutionThe wholesale price index (o price relative) of tomatoes in 2018 compared to 2016 is 150. If the cost of tomatoes was ₹ 15 per kg in 2016, calculate the cost in 2018.
View SolutionFor the data 1,2,3,4,5,6,7, Q1+Q3 is
View SolutionA pair of students is selected at random from a probability class.The probability that the pair selected will const of one male and one female student is (10)/(19) .Find the maximum number of students the class can contain.
View SolutionA Tennis tournament is to be played by 10 pairs of students and each pair is to play with every other pair one set.If four sets are played each day then the number of days should be allowed for the tournament is
View SolutionFollowing are the marks of 37 students: {:("Marks",4,6,8,10,12,14),("No. of Students",5,7,6,8,6,5):} Calculate mean marks, using Short-cut Method.
View SolutionFollowing are the marks of 37 students: {:("Marks",4,6,8,10,12,14),("No. of Students",5,7,6,8,6,5):} Calculate mean marks, using Short-cut Method.
View SolutionCalculate median marks of a class of 38 student by using lower limit method.
View Solutionकितनी विधियों द्वारा छात्र 9 में से 5 पाठ्यक्रम का चयन कर सकते है । जबकि सभी छात्रों के लिए 2 पाठ्यक्रम अनिवार्य है ?
View Solutionकल्पित माध्य विधि द्वारा 140 विद्यार्थियों द्वारा सांख्यिकी में प्राप्त ाक़ों का माध्य ज्ञात कीजिए:

View Solutionनिम्नलिखित वर्गीकृत आँकड़ों से किसी कूक्षा के विद्यार्थियों की परीक्षा के प्राप्तांकों के माध्य की गणना पद विचलन विधि द्वारा कीजिए।
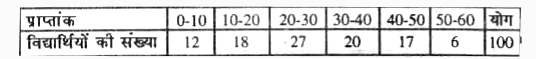
View SolutionThe stock and the seam are paired in the paired pen method.
View SolutionThe following table shows the marks scored by 140 students in an examination of a certain paper: Marks: 0-10 10-20 20-30 30-40 40-50 No. of students: 20 24 40 36 20 Calculate the average marks by using the assumed mean deviation method.
View Solutionनिम्न में से किस जोड़े को डॉक्टर. एक से अधिक बच्चे की सलाह नहीं देगा?
View SolutionThe marks of 8 students in an examination in Statistics and Mathematics are given below

Find Spearman's rank Correlation coefficient.
View Solution
