Question

A retired person has Rs 70,000 to invest and two types of bonds are available in the in the market investment. First type of bond yields an annual income of 8% on the amount investment invested and second type of bond yields 10% per annum. As per norms, he has to invest minimum in the first type and not more than 30,000 in the second type. How should investment, so as to get maximum returns, after one year of investment?

Explanation

Let bonds A be at `8%` and bonds B be at `10%.`
Suppose he plans to invest Rs x in bonds A and Rs y in bonds B.
Then, clearly `x+y=70000." "…(i)`
He invest minimum of Rs10000 in bonds A.
`therefore x ge10000." "…(ii)`
Also, he invests not more than Rs.30000 in bonds B.
`thereforeyle30000" "...(iii)`
Let z be the annual return on these investment. Then,
`z=(8x)/(100)+(10y)/(100)impliesz=0.08+0.1y." "...(iv)`
Thus we have to maximize z, subject to the conditions
`{:(x+y=70000),(xge10000),(y le30000):}}`
Now, `x+y=70000implies(x)/(70000)+(y)/(70000)=1.`
This line meets the axes at `A(70000,0) and B(0,70000).`
Plot these points and join them to obtain the line `x+y=70000.`
Clealry, (0,0) satisfies `x+yle70000.`
So, the region below AB represents `x+yle70000.`
` xge10000` is the region parallel to the y-axis and to the right of it beyond the line x=10000.
Thus, the region to the right of line CD represents `x ge10000.`
`yle30000` is the region parallel to the x-axis and above it, but below the line `y=30000.`
Thus, the regio below the line EF and above the x-axis represents `y le30000.`

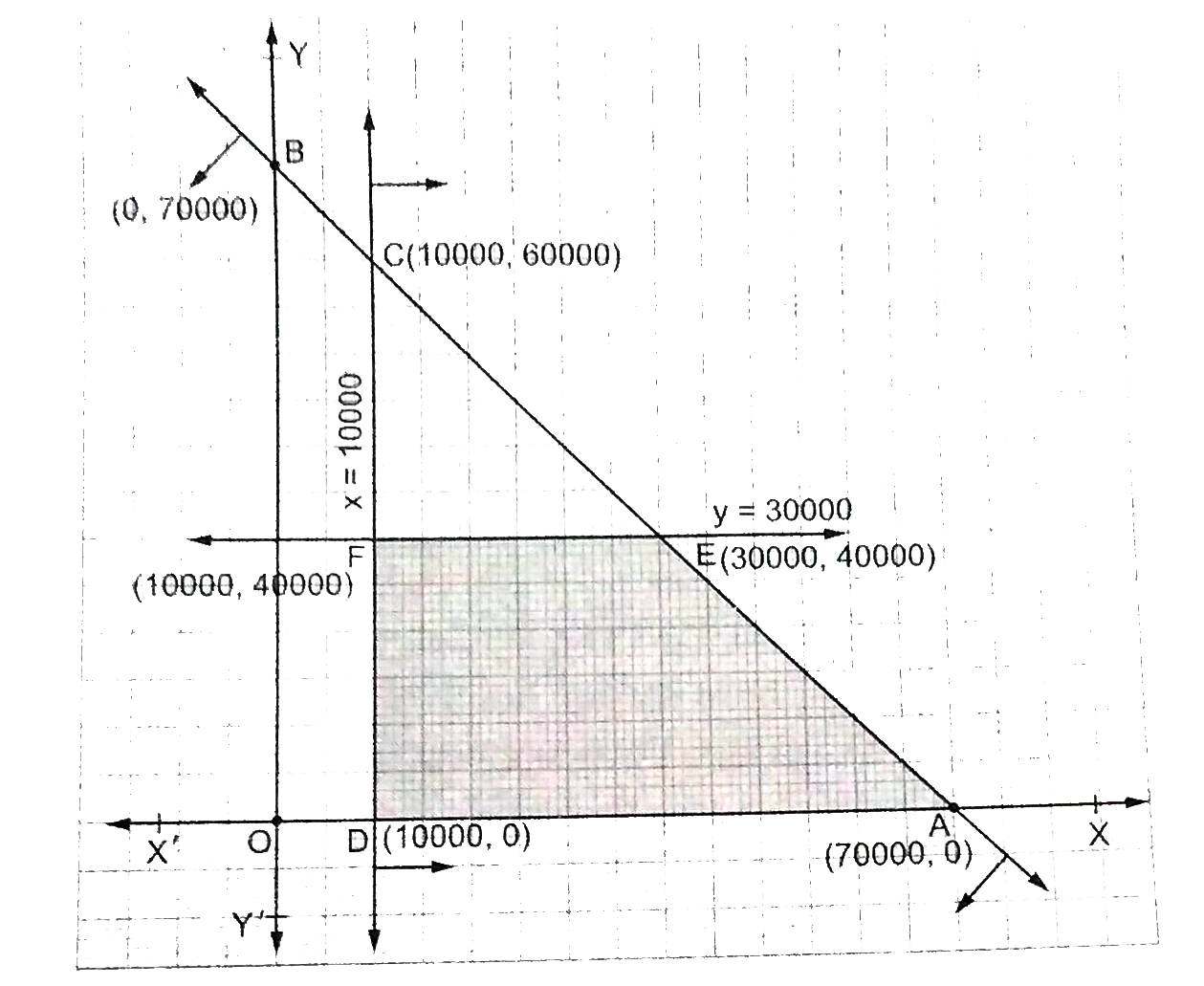
Thus, the corner points of the feasible region are
`D(1000,0)A(70000,0),E(30000,40000) andF(10000,40000).`
Value of annual return `z=0.08x+0.1y:`
(i) At `D(10000,.0)"it is z"=(0.08xx10000+0.1xx0)=800.`
(ii) At `A(70000,0)"it is z"=(0.08xx70000+0.1xx0)=5600.`
(iii) At `E(30000,40000)"it is z"(0.08xx30000+0.1xx40000)=6400.`
(iv) At `F(10000,4000)"it is z"=(0.08xx10000+0.1xx40000)=4800.`
So, in order to get a maximum annual return, he should invest Ra.30000 in bond A and Rs. 40000 in bond B.