A right circular cone with radius R and height H contains a liquid which evaporates at a rate proportional to its surface area in contact with air (proportionality constant k is positive). Suppose that r(t) is the radius of the liquid cone at time t. The time after which the cone is empty is
Let at time t ,r and h be the radius and height of cone of water respectively. Thus, at time t, surface and area of liquid in contact with air =πr2

Now according to question −dVdt∝πr2 Therefore ["-" sign shows that V decreases with time]
Or dVdt=−kπr2.............(1)
But from the figure, we get [Similar Δs]
rh=Rh or h=rHR
or 13ddt[r2rHR]=−kr2 (from 1)
or drdt=−kRH
or r=−kRHt+C (interating)
Now, at t=0, r=R
∴R=0+C or C=R
∴r=−kRtH+R
Now, let the time at which cone is empty be T. Then at T,r=0 (no liquid is left).
∴0=−kRTH+R or T=H/k
Topper's Solved these Questions
- DIFFERENTIAL EQUATIONSBOOK - CENGAGECHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Exercise (Single)
74 Videos
- DIFFERENTIAL EQUATIONSBOOK - CENGAGECHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Exercise (Multiple)
17 Videos
- DIFFERENTIAL EQUATIONSBOOK - CENGAGECHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Exercise 10.8
7 Videos
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS BOOK - CENGAGECHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Exercise
337 Videos
- DIFFERENTIATIONBOOK - CENGAGECHAPTER - DIFFERENTIATIONEXERCISE - Numerical Value Type
3 Videos
Similar Questions
A right circular cone with radius R and height H contains a liquid which evaporates at a rate proportional to its surface area in contact with air (proportionality constant k is positive). Suppose that r(t) is the radius of the liquid cone at time t. The radius of water cone at t = 1 is
View SolutionA right circular cone with radius R and height H contains a liquid which evaporates at a rate proportional to its surface area in contact with air (proportionality constant =k>0) . Find the time after which the cone is empty.
View Solution
Knowledge Check
The radius of a circular cone is R and its height is H. The volume of cone is :
AπR2HB23πR2HCπR2H3DπRH2The cone of radius R and height H is hanging inside a liquid of density by means of a string a shwon in the figure.
 ANet force on the cone by the liquid is equal to buoyancy force on the cone.BForce on the bottom surfae of the cone is more than buoyancy force on the cone.CNet force on the slant surface of the cone is more than buoyancy force on the cone.DNet force on the slant surface of the cone is less than buoyancy force on the cone.
ANet force on the cone by the liquid is equal to buoyancy force on the cone.BForce on the bottom surfae of the cone is more than buoyancy force on the cone.CNet force on the slant surface of the cone is more than buoyancy force on the cone.DNet force on the slant surface of the cone is less than buoyancy force on the cone.The radius of a right circular cone is 3 cm and its height is 4 cm. The total surface area of the cone is
A48.4 sq. cmB64.4 sq. cmC96.4 sq. cmD75.4 sq. cm
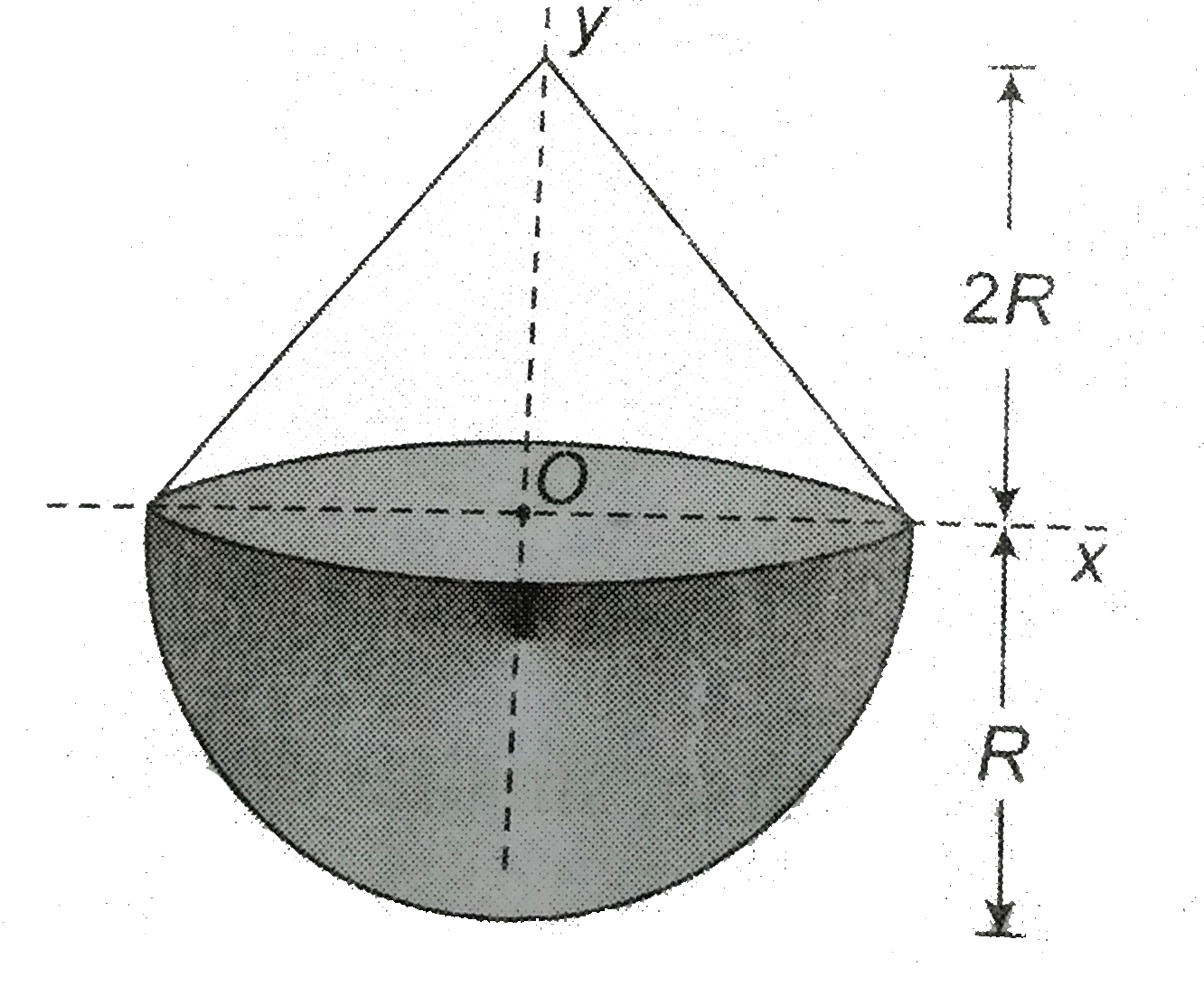
A right circular cone of radius R and height 2R is placed on a hemisphere of radius R. Locate c.m. of the combined mass from O.
View Solutionspherical rain drop evaporates at a rate proportional to its surface area. The differential equation corresponding to the rate of change of the radius of the rain drop if the constant of proportionality is K>0 is
View SolutionA right circular cone has height 8 cm. If the radius of its base is 6 cm, then what is its total surface area ?
View SolutionAn inverted cone of height H, and radius R is pointed at bottom. It is completely filled with a volatile liquid. If the rate of evaporation is directly proportional to the surface area of the liquid in contact with air (constant of proportionality k > 0). Find the time in which whole liquid evaporates.
View SolutionAn inverted frustum (truncated cone) is mounted on a hemisphere of radius R. Height of the frustum is h with an opening of radius r. The vessel is filled with water. If the rate of evaportion of the liquid is proportional (constant of proportionality k>0) to the surface area of water in contact with air, find the time is which the vessel will be amptied.
View Solution
