A room has 3 lamps . From a collection of 10 light bulbs of which 6 are no good , a person selects 3 at random and puts them in a socket . What is the probability , that he will have light ?
The correct Answer is:56
To solve the problem, we need to find the probability that at least one of the selected light bulbs is good. Here's a step-by-step solution:
Step 1: Understand the Problem
We have a total of 10 light bulbs, out of which 6 are defective (no good) and 4 are good. We need to select 3 bulbs at random and determine the probability that at least one of them is good.
Step 2: Calculate the Total Ways to Choose 3 Bulbs
The total number of ways to choose 3 bulbs from 10 is given by the combination formula (nr), where n is the total number of items, and r is the number of items to choose.
Total ways=(103)=10×9×83×2×1=120
Step 3: Calculate the Ways to Choose 3 Defective Bulbs
Next, we calculate the number of ways to choose 3 defective bulbs from the 6 defective ones.
Ways to choose 3 defective bulbs=(63)=6×5×43×2×1=20
Step 4: Calculate the Probability of Choosing 3 Defective Bulbs
The probability of choosing 3 defective bulbs (which means no light) is the ratio of the number of ways to choose 3 defective bulbs to the total ways to choose 3 bulbs.
P(3 defective)=Ways to choose 3 defectiveTotal ways=20120=16
Step 5: Calculate the Probability of Having Light
The probability of having at least one good bulb is the complement of the probability of having all defective bulbs.
P(at least 1 good)=1−P(3 defective)=1−16=56
Final Answer
Thus, the probability that the person will have light is:
56
We have a total of 10 light bulbs, out of which 6 are defective (no good) and 4 are good. We need to select 3 bulbs at random and determine the probability that at least one of them is good.
The total number of ways to choose 3 bulbs from 10 is given by the combination formula (nr), where n is the total number of items, and r is the number of items to choose.
Next, we calculate the number of ways to choose 3 defective bulbs from the 6 defective ones.
The probability of choosing 3 defective bulbs (which means no light) is the ratio of the number of ways to choose 3 defective bulbs to the total ways to choose 3 bulbs.
The probability of having at least one good bulb is the complement of the probability of having all defective bulbs.
Thus, the probability that the person will have light is:
Topper's Solved these Questions
- PROBABILITY BOOK - ICSECHAPTER - PROBABILITY EXERCISE - EXERCISE 22 (E )
24 Videos
- PROBABILITY BOOK - ICSECHAPTER - PROBABILITY EXERCISE - EXERCISE 22 (F)
30 Videos
- PROBABILITY BOOK - ICSECHAPTER - PROBABILITY EXERCISE - EXERCISE 22 (C )
50 Videos
- PRINCIPLE OF MATHEMATICAL INDUCTION BOOK - ICSECHAPTER - PRINCIPLE OF MATHEMATICAL INDUCTION EXERCISE - MULTIPLE CHOICE QUESTIONS
11 Videos
- PROPERTIES OF TRIANGLEBOOK - ICSECHAPTER - PROPERTIES OF TRIANGLEEXERCISE - EXERCISE 7
38 Videos
Similar Questions
By examining the. chest X-ray, the probability that TB is detected when a person is actually suffering is 0.99. The probability of an healthy person diagnosed to have TB is 0.001. In a certain city, 1 in 1000 people suffers from TB. A person is selected at random and is diagnosed to have TB. What is the probability that he actually has TB?
View SolutionFrom the employees of a company, 5 persons are selected to represent them in the managing committee of the company. Particulars of five persons are as follows:
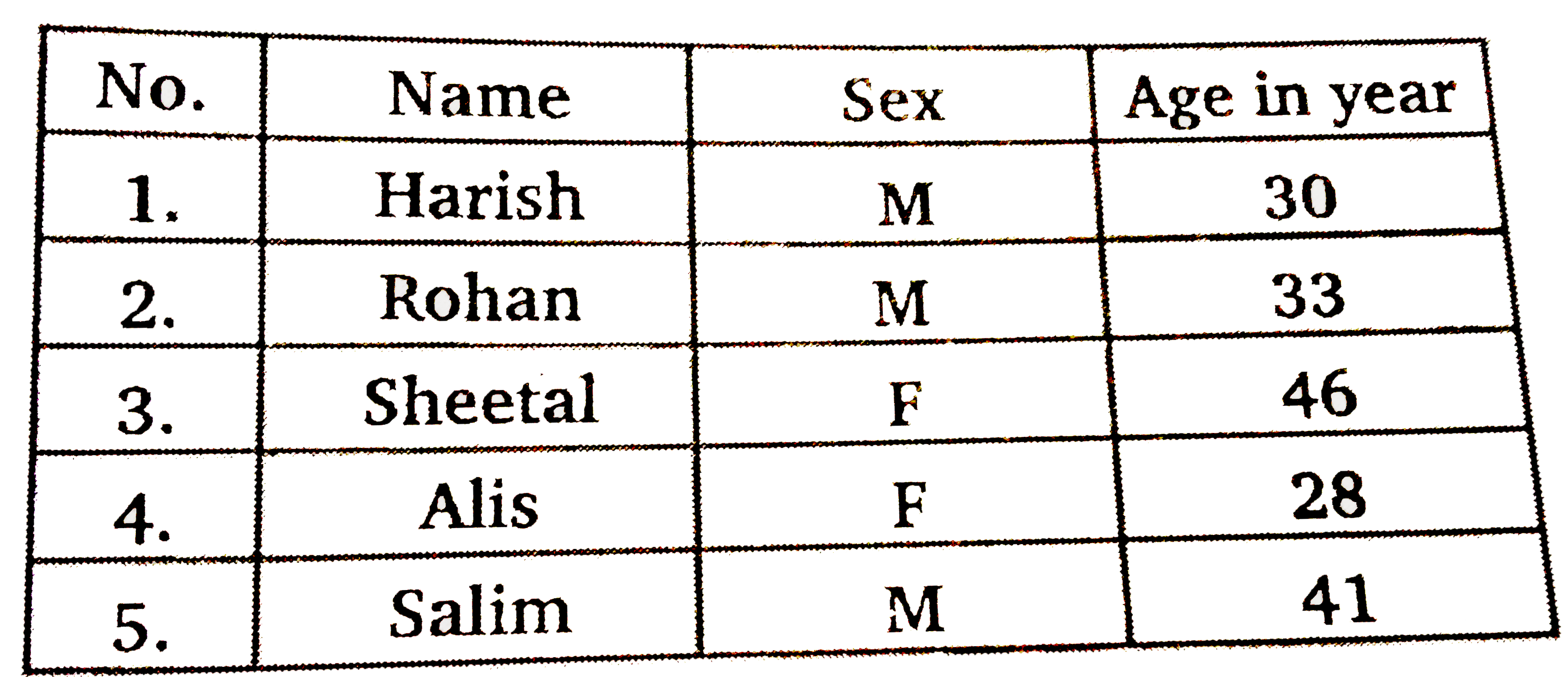
A person is selected at random from this group to act as a spokeperson. What is the probability that the spokesperson wil be either male or over 35 years?
View Solution
Knowledge Check
A bag contains 4 red balls, 5 green balls, and 3 blue balls. If a ball is selected at random from the bag, what is the probability that the ball selected will be green ?
A1/5B1/12C5/60D5/12Thirty percent of the 20 people in the math club have blonde hair. If 3 people are selecfted at random from the club what is the probability that none has blonde hair?
A0.1B0.25C0.32D0.4If x represents a number picked at random from the set {−3,−2,−1,0,1,2}, what is the probability that x will satisfy the inequality 4−3x<6?
A16B13C12D23
Suppose that the reliability of a HIV test is specified as follows:Of people having HIV, 90% of the test detect the disease but 10% go undetected. Of people free of HIV, 99% of the test are judged HIV-ive but 1% are diagnosed as showing HIV+ive. From a large population of which only 0.1% have HIV, one person is selected at random, given the HIV test, and the pathologist reports him/her as HIV+ive. What is the probability that the person actually has HIV?
View SolutionFrom the employees of a company 5 persons are elected to represent them n the managing committee of the company. Particulars of the five persons are as follows: S. No. Person Age (in years) 1 2 3 4 5 Male Male Female Female Male 30 33 46 28 41 A person is selected at random from this group to act as a spokesperson. What is the probability that the spokesperson will be either male or over 35 years?
View SolutionSuppose that 6% of the people with blood group O are left handed and 10% of those with other blood groups are left handed, 30 % of the people have blood groups O. If a left handed person os selected at random, what is the probability that he/she will have blood group O?
View SolutionSuppose that 6% of the people with blood group O are left handed and 10% of those with other blood groups are left handed, 30 % of the people have blood groups O. If a left handed person os selected at random, what is the probability that he/she will have blood group O?
View SolutionIn a certain town, 40% of the people have brown hair, 25% have brown eyes, and 15% have both brown hair and brown eyes. If a person selected at random from the town has brown hair, the probability that he also has brown eyes is 1/5 b. 3/8 c. 1/3 d. 2/3
View Solution
