A sealed cylindrical can holds three tennis balls each with diameter of 2.5 inches. If the can is designed to have the smallest possible volume, find the number of cubic inches of unoccopied space inside the can correct to the nearest tenth of a cubic inch.
The correct Answer is:12.3
Step by step video, text & image solution for A sealed cylindrical can holds three tennis balls each with diameter of 2.5 inches. If the can is designed to have the smallest possible volume, find the number of cubic inches of unoccopied space inside the can correct to the nearest tenth of a cubic inch. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - EXERCISE45videos
ADDITIONAL TOPICS IN MATH
- BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - Multiple Choice99videos
ADDITIONAL TOPICS IN MATH
- BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATH EXERCISE - PRACTICE TEST24videos
ADDITIONAL TOPICS IN MATH
- BOOK - ENGLISH SATCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE6videos
COMPLEX NUMBERS
Similar Questions
A laboratory supply company produces graduated cylinders, each with an internal radius of 2 inches and an internal height between 7.75 inches and 8 inches. What is one possible volume, rounded to the nearest cubic inch, of a graduated cylinder produced by this company?
View SolutionA coffee shop makes coffee in 5 gallon capacity urn and serves it in cylinderical-shaped mugs with an internal diameter of 3 inches. Coffee is poured into each mug at a height of about 4 inches. What is the lagrest number of full mugs of coffee that can be filled from the coffee urn if the urn is filled to capacity? (Note: There are 231 cubic inches in 1 gallon)
View Solution
Knowledge Check
- Question 1 - Select One
A 3-inch-tall rectangular box with a square base is constructed to hold a circular pie that has a diameter of 8 inches. Both are shown below. What is the volume, in cubic inches, of the smallest such box that can hold this pie?
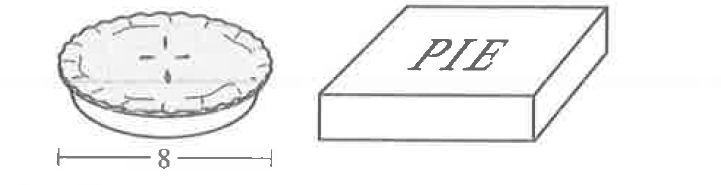 A24B64C72D192
A24B64C72D192 - Question 2 - Select One
Sophie has a hard rubber ball whose circumference measures 13 inches. She wants to store it in a box. What is the number of cubic inches in the volume of the smallest cube shaped box with integer dimensions that she can use?
A64B81C125D216 - Question 3 - Select One
A can of soda pop has the shape of a right circular cylinder with an inside height of 6 inches and an inside diameter of 2 inches. When you pour the soda pop from the full can into a cylindrical glas with an inside diameter of 3 inches, about how many inches high is the soda pop in the glass?
(Note: The volume of a right circular cylinder is πr2h)A223B4C5D623
An open metal bucket is in the shape of a frustum of a cone, mounted on a hollow cylindrical base made of the same metallic sheet. The diameters of the two circular ends of the bucket are 45 cm and 25 cm, the total vertical height of the bucket is 40cm and that of the cylindrical base is 6cm. Find the area of the metallic sheet used to make the bucket, where we do not take into account the handle of the bucket. Also, find the volume of water the bucket can hold. (Take π=22/7)
View SolutionAn open metal bucket is in the shape of a frustum of a cone, mounted on a hollow cylindrical base made of the same metallic sheet. The diameters of the two circular ends of the bucket are 45 cm and 25 cm, the total vertical height of the bucket is 40 cm and that of the cylindrical base is 6 cm. Find the area of the metallic sheet used to make the bucket, where we do not take into account the handle of the bucket. Also, find the volume of water the bucket can hold.
View SolutionThe vertical height of a conical tent is 42 dm and the diameter of its base is 5.4 m. Find the number of persons it can accommodate if each person is to be allowed 29.16 cubic dm.
View Solution
The head of a copper ''hexagon head screw bolt'' (one cross section of which is shown above) has the shape of a cylinder with a hole shaped like a regular hexagon. The cylindrical head is 2 cm thick with a base diameter of 3 cm. The hexagonal hole is only half the thickness of the entire head, and each side of a hexagonal cross section has a length of 1 cm. Given that the density of copper is 8.96 grams per cubic cm, and density is mass divided by volume, find the mass of the head to the nearest gram.View SolutionA software company is selling a new game in a standard edition and a collector’s edition. The box for the standard edition has a volume of 20 cubic inches, and the box for the collector’s edition has a volume of 30 cubic inches. The company receives an order for 75 copies of the game, and the total volume of the order to be shipped is 1,870 cubic inches. Which of the following systems of equations can be used to determine the number of standard edition games, s, and collector’s edition games, c, that were ordered?
View Solution

