A solid consisting of a right circular cone, standing on a hemisphere, is placed upright, in a right circular cylinder, full of water, and touches the bottom. Find the volume of water left in the cylinder, having given that the radius of the cylinder is 3 cm and its height is 6 cm, the radius of the hemisphere is 2 cm and the height of the cone is 4 cm. Give your answer correct to the nearest centimetre
( Take π=317)
The correct Answer is:136cm3
Step by step video, text & image solution for A solid consisting of a right circular cone, standing on a hemisphere, is placed upright, in a right circular cylinder, full of water, and touches the bottom. Find the volume of water left in the cylinder, having given that the radius of the cylinder is 3 cm and its height is 6 cm, the radius of the hemisphere is 2 cm and the height of the cone is 4 cm. Give your answer correct to the nearest centimetre ( Take pi = 3 (1)/(7)) by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
Similar Questions
A solid consisting of a right standing on a hemisphere is placed upright in a right circular cylinder full of water and touches the bottom. Find the volume of water left in the cylinder, if the radius of the cylinder is 60 cm and its height is 180 cm, the radius of the hemisphere is 60 cm and height of the cone is 120 cm, assuming that the hemisphere and the cone have common base.
View SolutionA solid consisting of a right circular cone of height 120 cm and radius 60 cm standing on a hemisphere of radius 60 cm is placed upright in a right circular cylinder full of water such that it touches the bottom. Find the volume of water left in the cylinder, if the radius of the cylinder is 60 cm and its height is 180 cm.
View SolutionA solid consisting of a right circular cone of height 120 cm and radius 60 cm standing on a hemisphere of radius 60 cm is placed upright in a right circular cylinder full of water such that it touches the bottom. Find the volume of water left in the cylinder, if the radius of the cylinder is 60 cm and its height is 180 cm.
View SolutionA solid right circular cone of height 120 cm and radius 60 cm is placed in a right circular cylinder full of water of height 180 cm.Such that it touhces the bottom .Find the volume of water of left in the cylinder, if the radius of the cylinder is equal to the radius to the cone.
 View Solution
View SolutionThe largest sphere is to be curved out of a right circular cylinder of radius 7 cm and height 14 cm. Find the volume of the sphere.
View SolutionThe largest sphere is to be curved out of a right circular cylinder of radius 7 cm and height 14 cm. Find the volume of the sphere.
View SolutionThe height of a circular cylinder is 20 cm and the radius of its base is 7 cm. Find :
the volumeView SolutionThe diameter of the base of a right circular cylinder is 42cm and its height is 10cm. Find the volume of the cylinder.
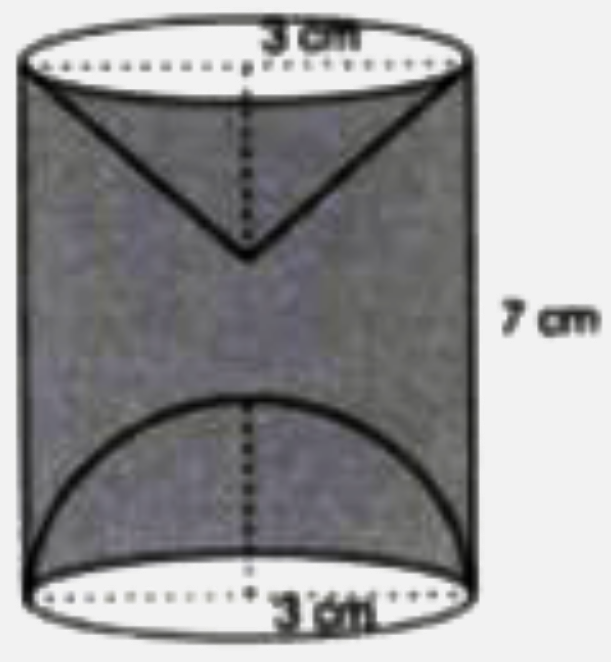
View SolutionA hemispherical and a conical hole are scooped out of a solid wooden cylinder. Find the volume of the remaining solid where the measurements are as as given alongside :
The height of the solid cylinder is 7 cm, radius of each of hemisphere, cone and cylinder is 3 cm. Height of cone is 3 cm.
Give your answer correct to the nearest whole number. Take π=227.
 View Solution
View SolutionThe height of a right circular cone is 7 cm and radius is 24 cm. Find the slant height of the cone.
View Solution
