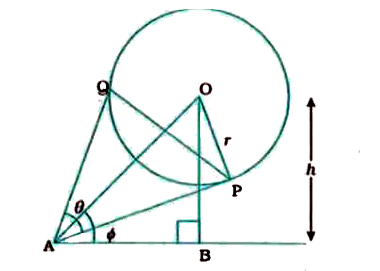
A spherical balloon of radius r subtends an angle α at the eye of anb observer. If the angle of elevation of the cerntre of the balloon be β, then prove that the height of the balloon is
r cosecα2sinβ. Solution in Bengali
[Hint : ∠QPC=α
∴ ∠QPB=∠BPC=α2
∠PQB=90∘
sin(α)2=QBPB=rl
⇒ lsin(α)2=r
⇒l=rsinα2=rcosecα2..............(1)
In ΔPOB,sinβ=OBPB=hl ltbtgt ⇒h=lsinβ
⇒h=rcosecα2sinβ.(Proved)]

Topper's Solved these Questions
- APPLICATION OF TRIGONOMETRIC RATIOS: HEIGHTS AND DISTANCESBOOK - CALCUTTA BOOK HOUSECHAPTER - APPLICATION OF TRIGONOMETRIC RATIOS: HEIGHTS AND DISTANCESEXERCISE - (Short-answer type question (SA):)
4 Videos
- COMPOUND INTERESTBOOK - CALCUTTA BOOK HOUSECHAPTER - COMPOUND INTERESTEXERCISE - SHORT ANSWERS TYPE QUESTIONS
10 Videos
Similar Questions
The shadow of a pole is 9 m long when the angle of elevation of the sun is 30∘.Find the length of shadow when the angle fo elelvation of the sun is 60∘.
View SolutionMr. Patul deposited Rs. 15000 in a bank. If the rate of annual compound interest be 12% then find the amount after 9 months when interests is compounded at the interval of 3 months.
View SolutionA round balloon of radius r subtends an angle alpha at the eye of the observer while the angle of elevation of its centre is beta Prove that the height of the centre of the balloon is r sin beta cos ec[(alpha)/(2)]
View SolutionA round balloon of radius r subtends an angle alpha at the eye of the observer while the angle of elevation of its centre is beta. Prove that the height of the centre of the balloon is r sin(beta)cos ec(alpha)/(2)
View SolutionA round balloon of radius r subtends an angle at the eye of the observer while the angle of elevation of its centre is β. Prove that the height of the centre of the balloon is rsinβcosecα2.
View SolutionA balloon of radius r subtends an angleα at the eyes of an observer and the center of balloon from the eye is β . Find the height of the center of the balloon from the eye of observer .
View Solutionएक गोलाकार गुब्बारा जिसकी त्रिजया r है, दर्शक की आंख पर α कोण बनाता है और इसके केन्द्र का आंख से उन्नयन कोण β हैं सिद्ध कीजिए कि गुब्बारे के केंद्र की पृथ्वी से ऊंचाई rsinβcosecα2 है।
View Solutionr त्रिज्या का एक गोल गुब्बारा प्रेक्षक की आँख पर उन्नयन कोण α बनाता है तथा इसके केंद्र का उन्नयन कोण β है| सिद्ध कीजिए की गुब्बारे के केंद्र की ऊंचाई rsinβcosecα2 है|
View Solutionr त्रिज्या वाला एक गोलाकार गुब्बारा, किसी प्रेक्षक की आँख पर कोण α करता है, जबकि इसके केन्द्रबिन्दु पर उन्नयन कोण β है । गुब्बारे के केन्द्रबिन्दु की ऊँचाई क्या है । (प्रेक्षक की ऊँचाई को नजर अन्दाज करते हुए ) ?
View SolutionA round balloon of radius 'r' subtends an angle α at the eye of the observer, while the angle of elevation of its centre is β. Find the height of the centre of balloon.
View SolutionA round balloon of radius r subtends an angle a at the eye of the observer while the angle of elevation of its centre is p. Prove that the height of the centre of the balloon B is r sin β cosec α2.
View Solutionएक r त्रिज्या का गोलीय गुब्बारा , किसी प्रेक्षक की आँख पर α कोण बनाता है। यदि गुब्बारे के केंद्र का उन्नयन कोण β हो, तो गुब्बारे के केंद्र की ऊंचाई है
View SolutionA spherical balloon subtends an angle 2α at a man's eye and the elevation of its centre is β. If θ is the elevation of the hightest point of the balloon at A then tanθ is equal to
View SolutionA balloon of radius r subtends an angleα at the eyes of an observer and the center of balloon from the eye is β . Find the height of the center of the balloon from the eye of observer .
View SolutionA spherical balloon of radius r subtends an angle θ at the eye of an observer. If the angle of elevation of its centre is ϕ, find the height of the centre of the balloon.
View Solution
