A square and a rectangle have :
only one line of symmetry each
two lines of symmetry each
four lines of symmetry each
an unequal number of lines of symmetry
To solve the question about the number of lines of symmetry in a square and a rectangle, we can follow these steps:
1. Understanding Symmetry Lines:
- A line of symmetry is a line that divides a shape into two identical halves. Each half is a mirror image of the other.
2. Finding Symmetry Lines in a Square:
- A square has four equal sides and four right angles.
- We can draw the following lines of symmetry:
- Vertical Line: Divides the square into two equal rectangles.
- Horizontal Line: Divides the square into two equal rectangles.
- Diagonal Line (from top left to bottom right): Divides the square into two equal triangles.
- Diagonal Line (from top right to bottom left): Divides the square into two equal triangles.
- Therefore, a square has 4 lines of symmetry.
3. Finding Symmetry Lines in a Rectangle:
- A rectangle has opposite sides that are equal in length.
- We can draw the following lines of symmetry:
- Vertical Line: Divides the rectangle into two equal parts.
- Horizontal Line: Divides the rectangle into two equal parts.
- Diagonal lines do not create equal halves in a rectangle.
- Therefore, a rectangle has 2 lines of symmetry.
4. Comparing the Symmetry Lines:
- A square has 4 lines of symmetry.
- A rectangle has 2 lines of symmetry.
- Thus, they do not have the same number of symmetry lines.
Final Answer:
- A square has 4 lines of symmetry, while a rectangle has 2 lines of symmetry. Therefore, they do not have the same number of symmetry lines.
- A line of symmetry is a line that divides a shape into two identical halves. Each half is a mirror image of the other.
- A square has four equal sides and four right angles.
- We can draw the following lines of symmetry:
- Vertical Line: Divides the square into two equal rectangles.
- Horizontal Line: Divides the square into two equal rectangles.
- Diagonal Line (from top left to bottom right): Divides the square into two equal triangles.
- Diagonal Line (from top right to bottom left): Divides the square into two equal triangles.
- Therefore, a square has 4 lines of symmetry.
- A rectangle has opposite sides that are equal in length.
- We can draw the following lines of symmetry:
- Vertical Line: Divides the rectangle into two equal parts.
- Horizontal Line: Divides the rectangle into two equal parts.
- Diagonal lines do not create equal halves in a rectangle.
- Therefore, a rectangle has 2 lines of symmetry.
- A square has 4 lines of symmetry.
- A rectangle has 2 lines of symmetry.
- Thus, they do not have the same number of symmetry lines.
- A square has 4 lines of symmetry, while a rectangle has 2 lines of symmetry. Therefore, they do not have the same number of symmetry lines.
Topper's Solved these Questions
Similar Questions
A square and a rectangle are equal in area. If the side of the square is 24 cm and the length of the rectangle is 32 cm, find its breadth.
View SolutionThe area of a square and a rectangle are equal.If the side of the square is 40 cm and the breadth of the rectangle is 25 cm,find the length of the rectangle. Also,find the perimeter of the rectangle.
View SolutionThe area of a square and a rectangle are equal. If the side of the square is40 cm and the breadth of the rectangle is 25 cm, find the length of therectangle. Also, find the perimeter of the rectangle.
View SolutionThe perimeter of a square and a rectangle are equal. If the square is of side 14 cm and the length of the rectangle is 16cm, find its breadth.
View SolutionThe area of a square and a rectangle are equal. If the length and breadth of the rectangle is 40 cm and 10 cm, respectively, find the side of the square. Also find the perimeter of the square.
View SolutionConsider the following sentence: Every square is a rectangle. Is it a statement?
View SolutionFill in the blanks in each of the following, so as to make the statement true: A rectangle is a parallelogram in which.... A square is a rhombus in which.... A square is a rectangle is which....
View SolutionFind the area of the field shown in Figure by dividing it into a square, a rectangle and a trapezium.
View SolutionIn each of the following cases ABCD is a square and PQRS is a rectangle. Find, in each case, the area of the shaded portion. (All measurements are in metre).
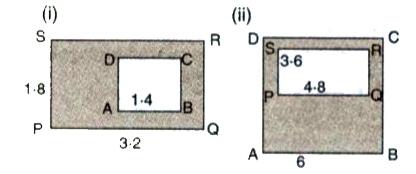 View Solution
View SolutionThe side of a square is 6cm. A rectangle has the same perimeter as that of the square.
Which has the greater area: the square or the rectangle?View Solution

