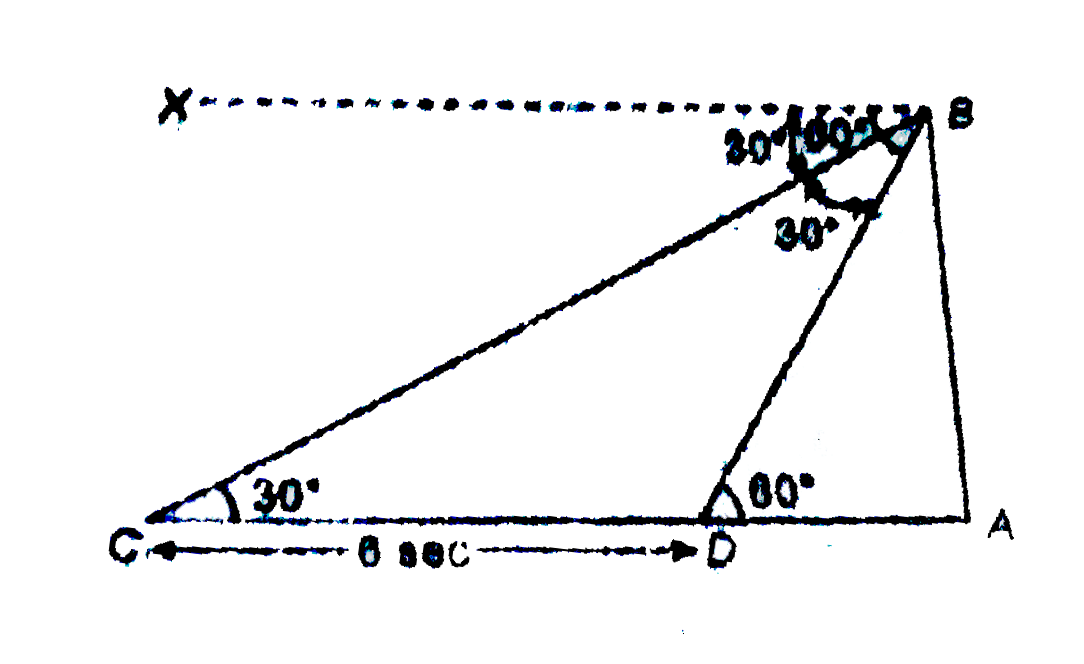
A straight highway leads to the foot of a tower. A man standing at the top of the tower observes a car at an angle of depression of 30o , which is approaching the foot of the tower with a uniform speed. Six seconds later, the angle of depres
Let AB be the height of the tower and C,D be the two positions of the car.
∠DBC=∠DBX−∠CBX=60∘−30∘=30∘
∴ In ΔBDC,
∠DBC=∠DCB(30∘ each )
∠CD=BD
(∴ sides opposite to equal angles are equal)
In right ΔBDC,
cos60∘=ADDB⇒12=ADDB⇒DB=2AD
Now, time taken to cover the distance CD = 6 sec.
∴ Time taken to cover the distance 2AD=6sec.
⇒ Time taken to cover the distance AD = 3 sec.
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Exercise32videos
SOME APPLICATIONS OF TRIGONOMETRY
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Revision Exercise (short Answer Questions)5videos
SOME APPLICATIONS OF TRIGONOMETRY
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - REAL NUMBERSEXERCISE - Revision Exercise Long Answer Questions5videos
REAL NUMBERS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions4videos
STATISTICS
Similar Questions
A straight highway leads to the foot of a tower. A man standing at the top of the tower observes a car at angle of depression of 30∘ , which is approaching to the foot of the tower with a uniform speed. Six seconds later, the angle of depression of the car is found to be 60∘ . Find the further time taken by the car to reach the foot of the tower.
View SolutionA straight highway leads to the foot of a tower of height 50 m. From the top of the tower, the angles of depression of two cars standing on the highway are 30∘ and 60∘ respectively. What is the distance between the two cars and how far is each car from the tower?
View SolutionFrom the top of a cliff 300 metres high, the top of a tower was observed at an angle of depression 30∘ and from the foot of the tower the top of the cliff was observed at an angle of elevation 45∘, The height of the tower is
View SolutionFrom the top of a 7m high building, the angle of elevation of the top of a cable tower is 60o and the angle of depression of its foot is 45o . Determine the height of the tower.
View SolutionFrom the top of a 7 m high building, the angle of elevation of the top of a tower is 60° and the angle of depression of the foot of the tower is 30°. Find the height of the tower.
View SolutionFrom the top of a 7m high building, the angle of elevation of the top of a cable tower is 60∘ and the angle of depression of its foot is 45∘. Determine the height of the tower.
View SolutionFrom the top of a 7 m high building, the angle of elevation of the top of a cable tower is 60oand the angle of depression of its foot is 45o. Determine the height of the tower.
View SolutionFrom the top of a tower h m high, angles of depression of two objects, which are in line with the foot of the tower are α and β(β>α). Find the distance between the two objects.
View SolutionFrom the top of a tower h m high, angles of depression of two objects, which are in line with the foot of the tower are α and β(β>α). Find the distance between the two objects.
View SolutionThe angle of elevation of the top of a tower from a point on the ground is 60∘. which is 25 m away from the foot of the tower. Find the height of the tower
View Solution
