A tangent is drawn to each of the circles x2+y2=a2 and x2+y2=b2. Show that if the two tangents are mutually perpendicular, the locus of their point of intersection is a circle concentric with the given circles.Solution in Bengali
In the figure, from variable point P(h,k) tangents PA and PB are drawn to two circles x2+y2=a2 and x2+y2=b2. Tangents PA and PB are perpendicuarl .
Clearly, OAPB is a reactangle.
∴OP2=OB2+BP2=h2+k2=a2+b2
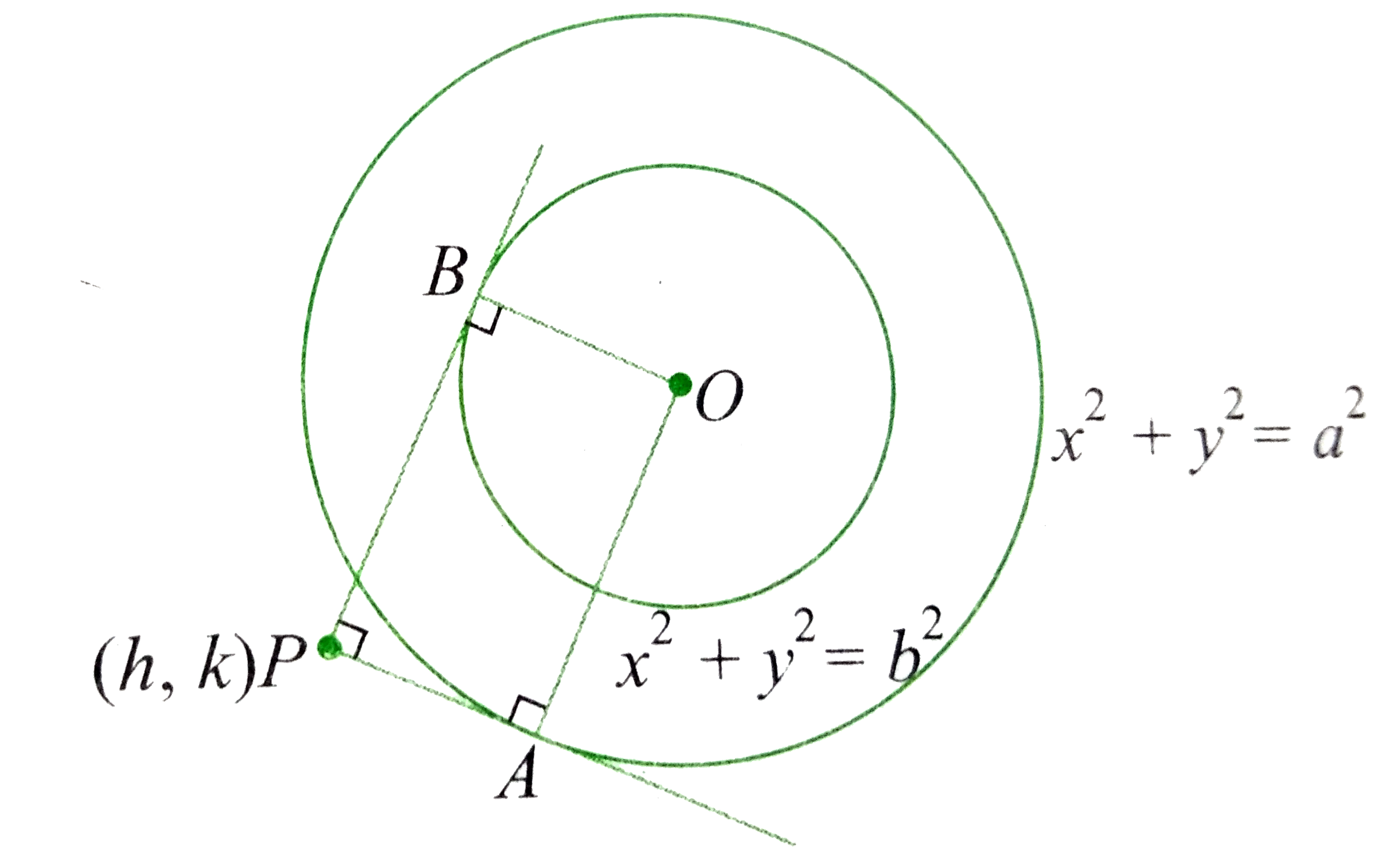
Therefore, required locus is x2+y2=a2+b2, which is concentric circle with given circles.
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.13
4 Videos
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.14
4 Videos
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.11
4 Videos
- BINOMIAL THEOREMBOOK - CENGAGE PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
- CIRCLESBOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
Similar Questions
Prove that the locus of the centroid of the triangle whose vertices are (acost,asint),(bsint,−bcost), and (1,0) , where t is a parameter, is circle.
View SolutionFind the locus of center of circle of radius 2 units, if intercept cut on the x-axis is twice of intercept cut on the y-axis by the circle.
View SolutionProve that the locus of the point that moves such that the sum of the squares of its distances from the three vertices of a triangle is constant is a circle.
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View Solution
In the diagram as shown, a circle is drawn with centre C(1,1) and radius 1 and a line L. The line L is tangent to the circle at Q. Further L meets the y-axis at R and the x-axis at P in such a way that the angle OPQ equals θ where 0<θ<π2.
Coordinate of Q is
View SolutionThe locus of the point of intersection of the two tangents drawn to the circle x^(2)+y^(2)=a^(2) which include are angle alpha is
View SolutionA tangent is drawn to each of the circles x2+y2=a2 and x2+y2=b2. Show that if the two tangents are mutually perpendicular, the locus of their point of intersection is a circle concentric with the given circles.
View SolutionLocus of the point of intersection of perpendicular tangents drawn one to each of the circles x2+y2=8andx2+y2=12 is
View SolutionThe locus of the point of intersection of perpendicular tangents to the circles x2+y2=a2 and x2+y2=b2 , is
View SolutionFind the equation of circle passing through (1, 1) belonging to the system of co-axial circles that are tangent at (2, 2) to the locus of the point of intersection of mutually perpendicular tangent to the circle x2+y2=4.
View SolutionThe locus of the point of intersection of two perpendicular tangents to the circle x^(2)+y^(2)=a^(2)is
View SolutionThe locus of the point of intersection of the perpendicular tangents to the circle x2+y2=a2,x2+y2=b is
View SolutionA tangent is drawn to each of the circles x2+y2=a2 and x2+y2=b2. Show that if the two tangents are mutually perpendicular, the locus of their point of intersection is a circle concentric with the given circles.
View SolutionA tangent at a point on the circle x2+y2=a2 intersects a concentric circle C at two points PandQ . The tangents to the circle X at PandQ meet at a point on the circle x2+y2=b2. Then the equation of the circle is x2+y2=ab x2+y2=(a−b)2 x2+y2=(a+b)2 x2+y2=a2+b2
View SolutionFind the locus of the feet of the perpendiculars drawn from the point (b, 0) on tangents to the circle x2+y2=a2
View SolutionTangents are drawn from the point (17, 7) to the circle x2+y2=169, Statement I The tangents are mutually perpendicular Statement, ll The locus of the points frorn which mutually perpendicular tangents can be drawn to the given circle is x2+y2=338
View Solution
