A telephone company in the town has 5000 subscribers on its list and collects fixed rent charges of Rs. 3000 per year from each person. The company proposes to increase the annual rent and it is believed that for every increase of 1 rupee in the rent, one subscriber will be discontinued. Find what increased annual rental will bring the maximum annual income to the company.
900
1000
1200
1500
The correct Answer is:B
Let the annual rental be increased by Rs. x.
Then x subscribers will discontinue.
Now, the new annual rental = Rs. (3000+x)
and the number of subscribers =5000−x
∴ the total annual income =(3000+x)(5000−x)
Let f(x)=(3000+x)(5000−x)=15000000+2000x−x2
∴f'(x)=ddx(15000000+2000x−x2)
=0+2000×1−2x=2000−2x
and f''(x)=ddx(2000−2x)=0−2×1=−2
Now, f'(x) = 0 gives 2000−2x=0
∴x=1000 and f''(1000)=−2<0
∴ by the second derivative test, f is maximum when x = 1000
Hence, the annual rental should be increased by Rs. 1000 for maximum annual income.
Topper's Solved these Questions
- APPLICATIONS OF DERIVATIVES BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - APPLICATIONS OF DERIVATIVES EXERCISE - Examples for Practice
61 Videos
- APPLICATIONS OF DERIVATIVES BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - APPLICATIONS OF DERIVATIVES EXERCISE - MULTIPLE CHOICE QUESTIONS
12 Videos
- APPLICATIONS OF DEFINITE INTEGRALS ( AREA ) BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - APPLICATIONS OF DEFINITE INTEGRALS ( AREA ) EXERCISE - EXAMPLES FOR PRACTICE (3 OR 4 MARKS )
5 Videos
- BINOMIAL DISTRIBUTIONBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - BINOMIAL DISTRIBUTIONEXERCISE - MULTIPLE CHOICE QUESTIONS
8 Videos
Similar Questions
A telephone company in a town has 500 subscribers on its list and collects fixed charges of Rs 300 per subscriber. The company proposes to increase the annual subscription and it is believed that every increase of Rs 1 one subscriber will discontinue the service. Find what increase will bring maximum revenue?
View SolutionA magazine seller has 500 subscribers and collects annual subscription charges of Rs.300 per subscriber. Sh e proposes to increase the annual subscription charges and it is believed that for every increase of Re 1, one subscriber will discontinue. What increase will bring maximum income to her? M solution. Write one important role of magazines in our lives. ake appropriate assumptions in order to apply derivatives to reach the
View Solution
Knowledge Check
The following graph shows the annual premium of an insurance company. Charged for an insurance of Rs.1000 for different ages.
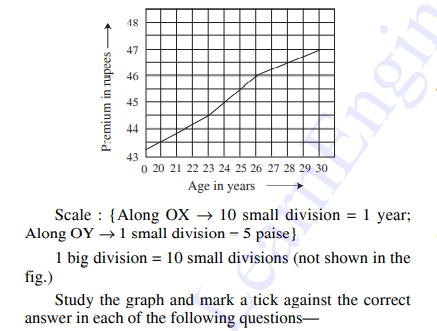
How much percent of the premium is increased if a man aged 30 years is insured for Rs.1000 instead of a man aged 23 years ?A4.75%B5.68%C6.24%D6%The following table shows the annual profit of a company (in Rs. lakh).

The period which has the maximum percentage increase in profit over the previous year is:A2018-2019B2015-2016C2017-2018D2016-2017
PVR is a renowned multiplex operators in India. It own 254 screens in 52 properties at 24 locations in the country. Considering the fact is more growing trend among the people to spend more of their disposable income on entertainment, company planned to add more screen at existing locations and start at new locations also they also plan to add food chain also at their locations. The company planned to float equity shares in market to raise the desired capital. The issue was fully subscribed and paid. Over the years the sale and the profit of the company have icreased tremendously and it has been declaring higher dividend and the market price of its share has increased manifolds.
(a) Name the different kinds of financial decisions taken by PVR Ltd. by quoting lines from para.
(b) Do you think the financial management team of the company has been able to achieve its prime objectivies why or why not.
View SolutionFlipkart is an e-commerce company founded in the year 2007 by Sachina Bansal and Binay Bansal. The company is registered in Singapore, but has its head quarters in Bangaluru, India. The company seeks to increase traffic on their site (more clicks on their product, and boost sale by next quarter).
Flipkart was the first company to offer "Cash on Delivery' facility. Before giving this offer, company scaned the environment and allocated huge fund to implement this offer. The company offer in general Two Weeks' Return option, but it may vary from product to product.
After receiving the order, the company follows a chronological sequence of steps till the delivery of product to customer. The company offer, various options of payment such as cash on delivery, Debit/Credit card, net banking, etc. This year company plants to spend Rs. 50 crore for advertisement to increase the sale. Flipkart reserve the right to terminate your membership or refuse to deliver product if it is discovered that you are under age of 18. This is because as per Indian Contact Act the minors are not eligible to use websites.
In context of above case, dentify and explain different types of plans being used by Flipkart by quoting the lines from the para.
View SolutionRising divdends can support valuations Over the next few years, companies cannot afforred to ignore dividends, Investors are looking for higher payouts and need the assureance of a stated dividend payouts and need the assureance of a stated dividends policy. In India , though there are frw compaines that are as consistent in divned payments , even over the past five years.
The dividend yield, thoughm has steadily declined and is now at an average of 1.1 per cent of a set of 800 companies. These companies form part of the various BSe and ESE indices. NOt only has the dividend yield gone down , there is not one company in this list that has increeased divdends in line with profit growth in each of the past five years.
Among compaines in the set, thouse that have steadily increased the payout ovber ther years include a number of mutinational compaines such as a high reutn on net worht. Companies such as Astrazeneca Pharma, Nestle India, Hindustan Lever, Clariant, Pfizer , GlxoSmithkine Consumer and Cummine Indida have enhanced divdnes to deliver value to shareholder.s Thse companies do not seem to be constrained for growth, either. Some Inidian companies that have also shown the way forward include Automative Axles, Ranbaxy LAbs, Hero Honda Motors, Asian Paints. Theremax and a number of banking and non-banking finance companies. These copmpanies , too, are growing fast, and the declaration of dividends has not dampened prospects.
Companies that have held on to profits and not declared dividends include e-serve, Cranes Software, Sesa Goa, Tata motors, Moser Baer, AVV ,MICO, Aztec Software, Havells Indian, Amtek Indai nad Sterlite Industries. This is only an indcative list and inclides many more. The dividend payout ratio in the case of the indicated companies is less than 20 per cent. Investors, however, need divends to risk and they aslo need a stated divdend policy. The earnings yield ( inverse of PE ratio) is now at about 6 per cent. if the payout ratio were stepped up to 40 per cent then the divden yield would rise to about 2.5 per cent.
Expain any three other factors affecting this desicison.
View SolutionRising divdends can support valuations Over the next few years, companies cannot afforred to ignore dividends, Investors are looking for higher payouts and need the assureance of a stated dividend payouts and need the assureance of a stated dividends policy. In India , though there are frw compaines that are as consistent in divned payments , even over the past five years.
The dividend yield, thoughm has steadily declined and is now at an average of 1.1 per cent of a set of 800 companies. These companies form part of the various BSe and ESE indices. NOt only has the dividend yield gone down , there is not one company in this list that has increeased divdends in line with profit growth in each of the past five years.
Among compaines in the set, thouse that have steadily increased the payout ovber ther years include a number of mutinational compaines such as a high reutn on net worht. Companies such as Astrazeneca Pharma, Nestle India, Hindustan Lever, Clariant, Pfizer , GlxoSmithkine Consumer and Cummine Indida have enhanced divdnes to deliver value to shareholder.s Thse companies do not seem to be constrained for growth, either. Some Inidian companies that have also shown the way forward include Automative Axles, Ranbaxy LAbs, Hero Honda Motors, Asian Paints. Theremax and a number of banking and non-banking finance companies. These copmpanies , too, are growing fast, and the declaration of dividends has not dampened prospects.
Companies that have held on to profits and not declared dividends include e-serve, Cranes Software, Sesa Goa, Tata motors, Moser Baer, AVV ,MICO, Aztec Software, Havells Indian, Amtek Indai nad Sterlite Industries. This is only an indcative list and inclides many more. The dividend payout ratio in the case of the indicated companies is less than 20 per cent. Investors, however, need divends to risk and they aslo need a stated divdend policy. The earnings yield ( inverse of PE ratio) is now at about 6 per cent. if the payout ratio were stepped up to 40 per cent then the divden yield would rise to about 2.5 per cent.
Which foctor affecting divinden decision is indicated in the first paragraph of the given case ?
View SolutionA private telephone company serving a small community makes a profit of Rs. 12.00 per subscriber, if it has 725 subscribers. It decides to reduce the rate by a fixed sum for each subscriber over 725, thereby reducing the profit by 1 paise per subscriber. Thus, there will be profit of Rs. 11.99 on each of the 726 subscribers, Rs. 11.98 on each of the 727 subscribers, etc. What is the number of subscribers which will give the company the maximum profit?
View SolutionA man borrows money from a finance company and has to pay it back in two equal half-yearly instalments of Rs. 7,396 each. If the interest is charged by the finance company at the rate of 15% per annum, compounded semi-annually, find the principal and the total interest paid.
View Solution
